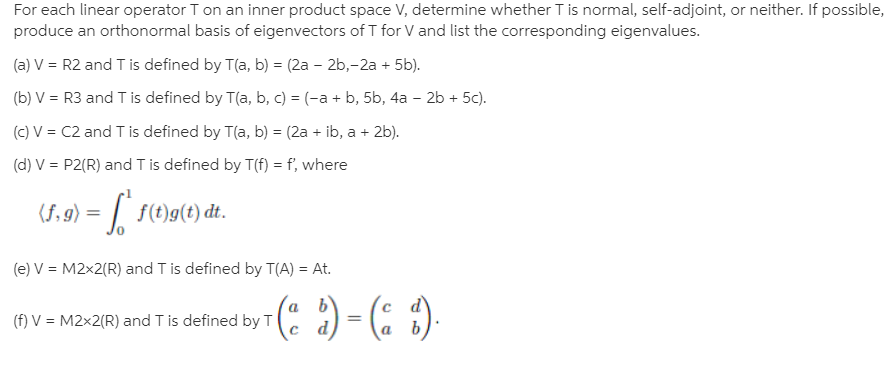
For each linear operator T on an inner product space V, determine whether T is normal, self-adjoint, or neither. If possible, produce an orthonormal basis of eigenvectors of T for V and list the corresponding eigenvalues. (a) V = R2 and T is defined by T(a, b) = (2a - 2b,-2a + 5b). (b) V = R3 and T is defined by T(a, b, c) = (-a + b, 5b, 4a - 2b + 5c). (c) V = C2 and T is defined by T(a, b) = (2a + ib, a + 2b). (d) V = P2(R) and T is defined by T(f) = f', where (S, 9) = | s(0)g(t) dt. (f. (e) V = M2x2(R) and T is defined by T(A) = At. %3! (* :) - (: ) (f) V = M2x2(R) and T is defined by T %3D

Consider again the matrix A in Exercise 35. Give conditions on a, b, c, and d such that A has two distinct real eigenvalues, one real eigenvalue, and no real eigenvalues.

Determine all nn symmetric matrices that have 0 as their only eigenvalue.

Define T:P2P2 by T(a0+a1x+a2x2)=(2a0+a1a2)+(a1+2a2)xa2x2. Find the eigenvalues and the eigenvectors of T relative to the standard basis {1,x,x2}.