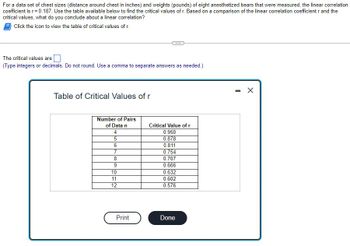
For a data set of chest sizes (distance around chest in inches) and weights (pounds) of eight anesthetized bears that were measured, the linear correlation coefficient is r = 0.187. Use the table available below to find the critical values of r. Based on a comparison of the linear correlation coefficient r and the critical values, what do you conclude about a linear correlation?

Listed below are annual data for various years. The data are weights (metric tons) of imported lemons and car crash fatality rates per 100,000 population. Construct a scatterplot, find the value of the linear correlation coefficient r, and find the P-value using a = 0.05. Is there sufficient evidence to conclude that there is a linear correlation between lemon imports and crash fatality rates? Do the results suggest that imported lemons cause car fatalities? Lemon Imports Crash Fatality Rate 231 266 359 480 532 15.9 15.7 15.4 15.2 14.8 Ay 17- Ay 17- Ay 17+ Ay 17- 16- 16- 16- 16- 15- 15- 15- 15- X 14- 14+ 14- 14- 200 400 600 200 400 600 200 400 600 200 400 600 The linear correlation coefficient r is (Round to three decimal places as needed.) The P-value is (Round to three decimal places as needed.) Because the P-value is than the significance level 0.05, there sufficient evidence to support the claim that there is a linear correlation between lemon imports and crash fatality rates for a…

A random sample of college students was surveyed about how they spend their time each week.  The scatterplot below displays the relationship between the number of hours each student typically works per week at a part- or full-time job and the number of hours of television each student typically watches per week.  The correlation between these variables is r = –0.63, and the equation we would use to predict hours spent watching TV based on hours spent working is as follows:             Predicted hours spent watching TV = 17.21 – 0.23(hours spent working) Since we are using hours spent working to help us predict hours spent watching TV, we’d call hours spent working a(n) __________________ variable and hours spent watching TV a(n) __________________ variable. The correlation coefficient, along with what we see in the scatterplot, tells us that the relationship between the variables has a direction that is _________________ and a strength that is ______________________. According to the…

Listed below are annual data for various years. The data are weights (metric tons) of imported lemons and car crash fatality rates per 100,000 population. Construct a scatterplot, find the value of the linear correlation coefficient r, and find the P-value using a = 0.05. Is there sufficient evidence to conclude that there is a linear correlation between lemon imports and crash fatality rates? Do the results suggest that imported lemons cause car fatalities? Lemon Imports Crash Fatality Rate 228 264 358 482 531 15.9 15.7 15.5 15.3 14.9

Listed below are the overhead widths (in cm) of seals measured from photographs and the weights (in kg) of the seals. Construct a scatterplot, find the value of the linear correlation coefficient r, and find the critical values of r using a = 0.05, Is there sufficient evidence to conclude that there is a linear correlation between overhead widths of seals from photographs and the weights of the seals? Overhead Width 7.1 7.5 178 Click here to view a table of critical values for the correlation coefficient. 9.6 9.4 8.8 8.2 Weight 112 205 238 204 188 OA. YB. OC. KD D. Aweight (kg) 300- A weight (kg) Aweight (kg) 300- Aweight (kg) 300- 300- .. 100 100+ 100 7. rect: 0 10 10 10 10 width (om) width (cm) width (on) widn (om) The linear correlation coefficient is r= (Round to three decimal places as needed.) Enter your answer in the answer box and then click Check Answer. O parts remaining Check Answer वी

Listed below are annual data for various years. The data are weights (metric tons) of imported lemons and car crash fatality rates per 100,000 population. Construct a scatterplot, find the value of the linear correlation coefficient r, and find the P-value using a= 0.05. Is there sufficient evidence to conclude that there is a linear correlation between lemon imports and crash fatality rates? Do the results suggest that imported lemons cause car fatalities? Lemon Imports Crash Fatality Rate 266 15.7 228 358 484 531 15.8 15.5 15.2 14.8 What are the null and alternative hypotheses? O B. Ho: p=0 O A. Ho: p#0 H1:p=0 H1:p0 H,: p#0 Construct a scatterplot. Choose the correct graph below. OA. B. Oc. OD. Ay 17- Ay 17- AY 17- Ay 17- 16- Q 16- 16- 16- 15- 15- 15- 15- 14- 14+ 14- 14- 200 400 600 200 400 600 200 400 6ỏ0 200 400 600 The linear correlation coefficient is r= (Round to three decimal places as needed.)

The table below includes data from taxi rides. The distances are in miles, the times are in minutes, the fares are in dollars, and the tips are in dollars. Is there sufficient evidence to conclude that there is a linear correlation between the time of the ride and the tip amount? Construct a scatterplot, find the value of the linear correlation coefficient r, and find the P-value of r. Determine whether there is sufficient evidence to support a claim of linear correlation between the two variables. Use a significance level of a = 0.01. Does it appear that riders base their tips on the time of the ride? Click here for information on the taxi rides. Construct a scatterplot. Choose the correct graph below. O A. Tip Amount ($) 25- Q 0 35 G Ride time (minutes) Determine the linear correlation coefficient. The linear correlation coefficient is r= (Round to three decimal places as needed.) Tip Amount ($) B. 25- 0- 0 35 G Ride time (minutes) Taxi data ip Amount (S C. 25- 0- 35 Ride time…

Listed below are the overhead widths (in cm) of seals measured from photographs and the weights (in kg) of the seals. Construct a scatterplot, find the value of the linear correlation coefficient r, and find the critical values of r using a = 0.01. Is there sufficient evidence to conclude that there is a linear correlation between overhead widths of seals from photographs and the weights of the seals? Overhead Width 7.2 7.5 9.8 9.3 8.9 8.1 Weight 119 172 248 201 205 181 Click here to view a table of critical values for the correlation coefficient. Construct a scatterplot. Choose the correct graph below. A. В. OC. D. Aweight (kg) 300- Aweight (kg) 300- Aweight (kg) 300- Aweight (kg) 300- 100+ 1004 100- 100+ 10 7 10 7 10 7 10 width (cm) width (cm) width (cm) width (cm) The linear correlation coefficient is r= (Round to three decimal places as needed.) The critical values are r= (Round to three decimal places as needed. Use a comma to separate answers as needed.) Because the absolute…

Police sometimes measure shoe prints at crime scenes so that they can learn something about criminals. Listed below are shoe print lengths, foot lengths, and heights of males. Construct a scatterplot, find the value of the linear correlation coefficient r, and find the P-value of r. Determine whether there is sufficient evidence to support a claim of linear correlation between the two variables. Based on these results, does it appear that police can use a shoe print length to estimate the height of a male? Use a significance level of a= 0.01. Shoe Print (cm) | 28.8 Foot Length (cm) 24.8 Height (cm) 30.8 30.4 31.1 28.6 24.6 27.8 26.1 25.3 177.6 179.2 179.2 169.4 169.5

Listed below are annual data for various years. The data are weights (metric tons) of imported lemons and car crash fatality rates per 100,000 population. Construct a scatterplot, find the value of the linear correlation coefficient r, and find the P-value using a = 0.05. Is there sufficient evidence to conclude that there is a linear correlation between lemon imports and crash fatality rates? Do the results suggest that imported lemons cause car fatalities? Lemon Imports Crash Fatality Rate 228 266 358 484 531 15.8 15.7 15.5 15.2 14.8 O A. Ho: pz0 H4:p= 0 O B. Ho: p=0 H1:p 0 H1: p#0 Construct a scatterplot. Choose the correct graph below. OA. YB. Oc. OD. Ay 17- Ay 17- Ay 17- Ay 17- 16- Q 16- 16- 16- 15- 15- 15- 15- X 14- 14- 14- 14+ 200 400 600 200 400 600 200 400 600 200 400 600 The linear correlation coefficient is r= - 0.971 (Round to three decimal places as needed.) The test statistic is t= (Round to three decimal places as needed.)