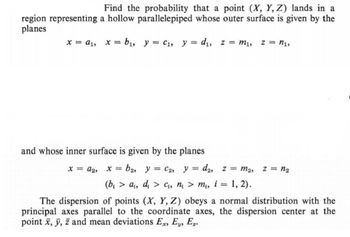
Find the probability that a point (X, Y, Z) lands in a a hollow parallelepiped whose outer surface is given by the region representing planes x = a₁, x=b₁, y = C₁, y = d₁, z = m₁, z = n₁, and whose inner surface is given by the planes X = A₂, b2, y = C2, y = d₂, z = m2, z = n₂ (b₁ > a₁, di > C₁₂ n₁ > m₁, i = 1, 2). The dispersion of points (X, Y, Z) obeys a normal distribution with the principal axes parallel to the coordinate axes, the dispersion center at the point x, y, z and mean deviations Ex, Ey, E.

List the vertices in R² of the convex region defined by Ax ≤ b and x ≥ A = 1 2 and b = 2 5 (A) (2,3), (½, ²), (2,0), (0,0), (0,3) (D) (2,3), ( ½, ³), (2,0), (0,0), (0,4) (G) (3,2), (½, ²), (2,0), (0,0), (0,4) where (B) (3,2), (½, ½), (2,0), (0, 0), (0,3) (E) (2,3), (½, ½), (2,0), (0, 0), (0, 3) (H) (3,2), (½, ½), (2,0), (0, 0), (0, 4) (C) (2,3), (2, 2), (2,0), (0,0), (0, 4) (F) (3,2), (3, 1), (2,0), (0,0), (0, 3)