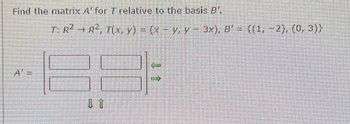
Find the matrix A' for T relative to the basis B'. Tea T: R² → R², T(x, y) = (x − y, y − 3x), B' = {(1, −2), (0, 3)} LE

Find the matrix A' for T relative to the basis B'. T: R³- →→>>> R³, T(x, y, z) = A' = (x - y + 7z, 7x + y - z, x + 7y + z), B' = {(1, 0, 1), (0, 2, 2), (1, 2, 0)}

Find the matrix A' for T relative to the basis B'. T: R² A' = X A' = 3 11/3 R², T(x, y) = (x - y, y - 2x), B' = {(1, -2), (0, 3)} -3 48 -16 -4 Find the matrix A' for T relative to the basis B'. ↓ 1 T: R² → R², T(x, y) = (-7x + y, 7x - y), B' = {(1, −1), (-1,5)} -72 24

Let f(u, v) = (u - v, 1, u + v) and g(x, y, z) = xyz. Find the entry a12 (i.e. the entry in the first row and second column) of the derivative matrices Df(u, v), Dg(x, y, z) and D(gof)(0, 1). (a) a 12 of Df(u, v) is (b) a12 of Dg(x, y, z) is (c) a12 of D(gof)(0, 1) is (d) Select the correct answer about D(g. f)(u, v) D(gof)(u, v) is a 1 x 2 matrix. D(gof)(u, v) is a 2 x 2 matrix. D(gof)(u, v) is a 3 x 2 matrix. D(gof)(u, v) is a 2 x 3 matrix. D(gof)(u, v) is a real-valued function of u and v. (e) Select the correct answer about D(f g)(x, y, z) OD(fog)(x, y, z) is not defined. D(fog)(x, y, z) is a real-valued function of x and y. D(fog)(x, y, z) is a 3 x 2 matrix. D(fog)(x, y, z) is a 2 x 2 matrix. D(fog)(x, y, z) is a 2 x 1 matrix.