Algebra and Trigonometry (6th Edition)
6th Edition
ISBN:9780134463216
Author:Robert F. Blitzer
Publisher:Robert F. Blitzer
ChapterP: Prerequisites: Fundamental Concepts Of Algebra
Section: Chapter Questions
Problem 1MCCP: In Exercises 1-25, simplify the given expression or perform the indicated operation (and simplify,...
Related questions
Question
Thank you in advance highly appreciated
![### Problem 2
**Task:**
Find the domain of the rational function and graph the function (show all work when graphing).
**Function:**
\[ f(x) = \frac{x}{x^2 - 16} \]
---
**Solution:**
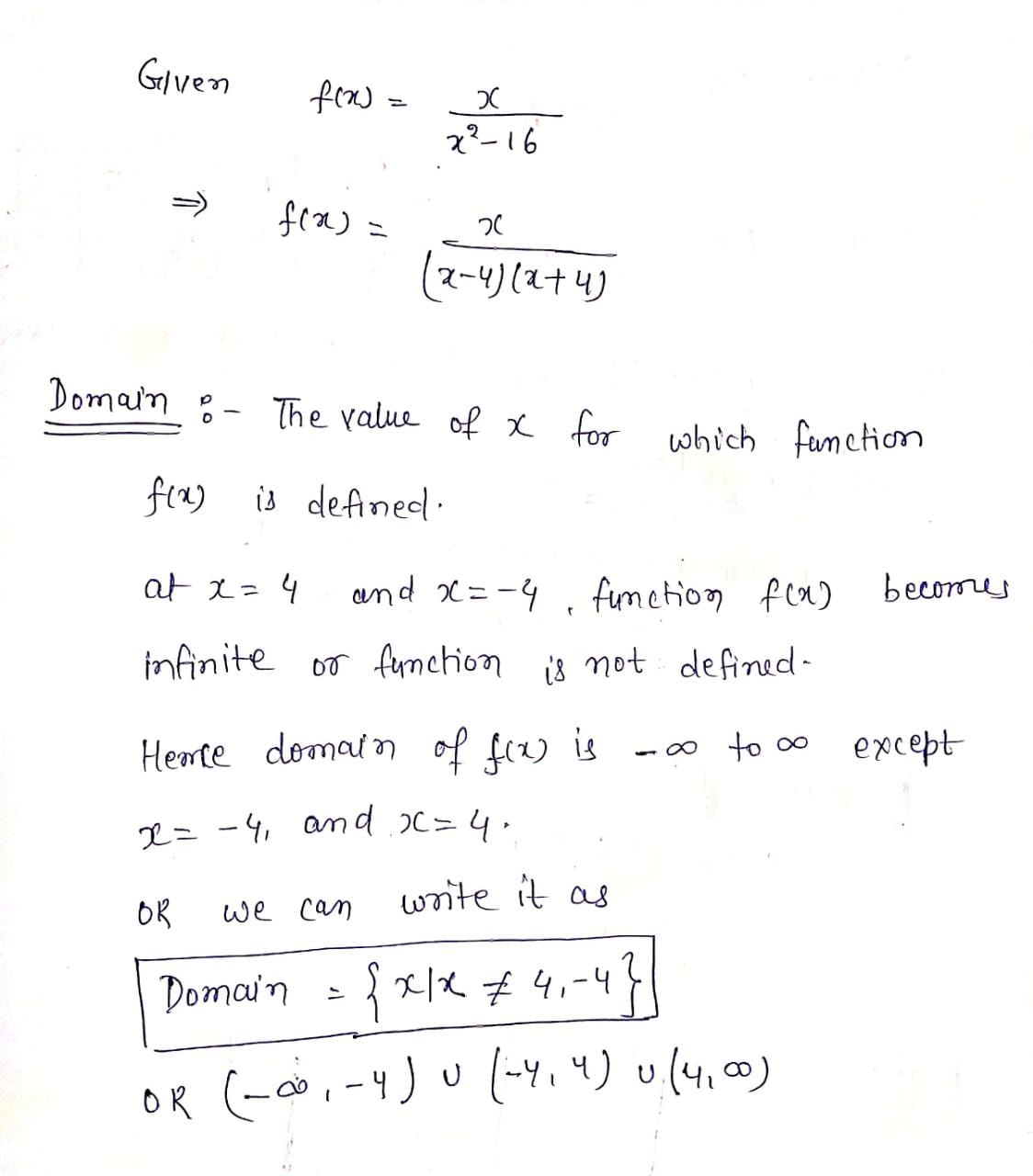
1. **Find the Domain:**
- The domain of a rational function excludes values that make the denominator equal to zero.
- Set the denominator equal to zero and solve for \( x \):
\[
x^2 - 16 = 0
\]
\[
x^2 = 16
\]
\[
x = \pm 4
\]
- Thus, the values \( x = 4 \) and \( x = -4 \) make the denominator zero.
- Therefore, the domain of \( f(x) \) is:
\[
\text{Domain: } \{ x \in \mathbb{R} \mid x \neq -4, x \neq 4 \}
\]
2. **Graph the Function:**
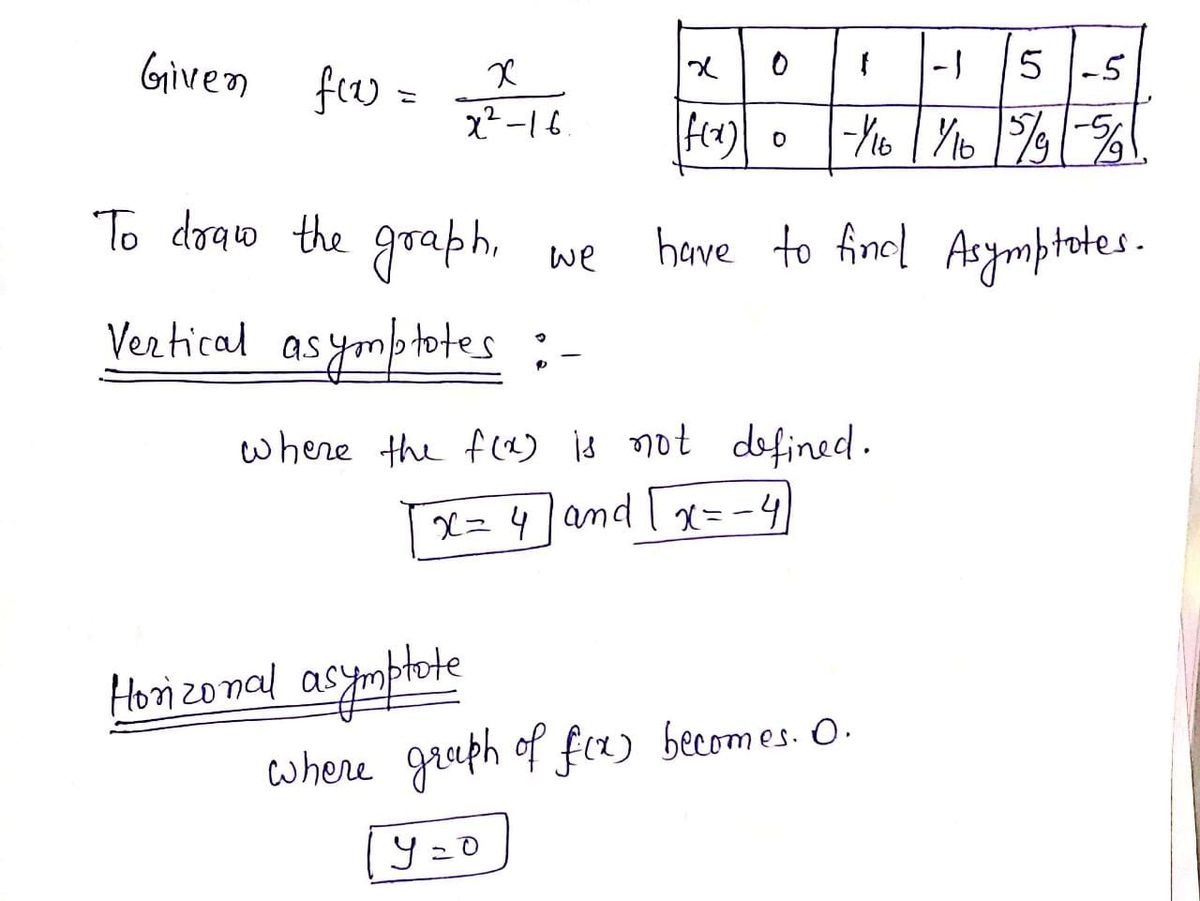
- To graph the function, plot several points for different values of \( x \).
- Identify vertical asymptotes at \( x = 4 \) and \( x = -4 \), where the function is undefined.
- Determine the behavior of the function as \( x \) approaches these asymptotes from both the left and right.
- Determine any horizontal asymptotes by analyzing the degrees of the polynomial in the numerator and the denominator.
- As \( x \) approaches large positive or negative values, because the degree of the numerator is 1 (which is less than the degree of the denominator, which is 2), the horizontal asymptote is \( y = 0 \).
- Plot additional points to create the curve of the function. Ensure to show all steps in the graphing process, including calculating values at various points and illustrating the asymptotic behavior.
**Note:** When graphing, start by plotting key points (such as intercepts), then move on to identify behavior near asymptotes, followed by plotting several additional points to accurately depict the curve.](/v2/_next/image?url=https%3A%2F%2Fcontent.bartleby.com%2Fqna-images%2Fquestion%2Fd59633dd-b674-4b8b-8a6e-1c7bb3ffab4f%2F1ba5bd69-0000-40f1-b0bb-a2bf29caada8%2F2px32c2i.jpeg&w=3840&q=75)
Transcribed Image Text:### Problem 2
**Task:**
Find the domain of the rational function and graph the function (show all work when graphing).
**Function:**
\[ f(x) = \frac{x}{x^2 - 16} \]
---
**Solution:**
1. **Find the Domain:**
- The domain of a rational function excludes values that make the denominator equal to zero.
- Set the denominator equal to zero and solve for \( x \):
\[
x^2 - 16 = 0
\]
\[
x^2 = 16
\]
\[
x = \pm 4
\]
- Thus, the values \( x = 4 \) and \( x = -4 \) make the denominator zero.
- Therefore, the domain of \( f(x) \) is:
\[
\text{Domain: } \{ x \in \mathbb{R} \mid x \neq -4, x \neq 4 \}
\]
2. **Graph the Function:**
- To graph the function, plot several points for different values of \( x \).
- Identify vertical asymptotes at \( x = 4 \) and \( x = -4 \), where the function is undefined.
- Determine the behavior of the function as \( x \) approaches these asymptotes from both the left and right.
- Determine any horizontal asymptotes by analyzing the degrees of the polynomial in the numerator and the denominator.
- As \( x \) approaches large positive or negative values, because the degree of the numerator is 1 (which is less than the degree of the denominator, which is 2), the horizontal asymptote is \( y = 0 \).
- Plot additional points to create the curve of the function. Ensure to show all steps in the graphing process, including calculating values at various points and illustrating the asymptotic behavior.
**Note:** When graphing, start by plotting key points (such as intercepts), then move on to identify behavior near asymptotes, followed by plotting several additional points to accurately depict the curve.
Expert Solution
Step 1
Step 2
Step by step
Solved in 3 steps with 3 images

Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, algebra and related others by exploring similar questions and additional content below.Recommended textbooks for you

Algebra and Trigonometry (6th Edition)
Algebra
ISBN:
9780134463216
Author:
Robert F. Blitzer
Publisher:
PEARSON

Contemporary Abstract Algebra
Algebra
ISBN:
9781305657960
Author:
Joseph Gallian
Publisher:
Cengage Learning

Linear Algebra: A Modern Introduction
Algebra
ISBN:
9781285463247
Author:
David Poole
Publisher:
Cengage Learning

Algebra and Trigonometry (6th Edition)
Algebra
ISBN:
9780134463216
Author:
Robert F. Blitzer
Publisher:
PEARSON

Contemporary Abstract Algebra
Algebra
ISBN:
9781305657960
Author:
Joseph Gallian
Publisher:
Cengage Learning

Linear Algebra: A Modern Introduction
Algebra
ISBN:
9781285463247
Author:
David Poole
Publisher:
Cengage Learning

Algebra And Trigonometry (11th Edition)
Algebra
ISBN:
9780135163078
Author:
Michael Sullivan
Publisher:
PEARSON

Introduction to Linear Algebra, Fifth Edition
Algebra
ISBN:
9780980232776
Author:
Gilbert Strang
Publisher:
Wellesley-Cambridge Press

College Algebra (Collegiate Math)
Algebra
ISBN:
9780077836344
Author:
Julie Miller, Donna Gerken
Publisher:
McGraw-Hill Education