
Advanced Engineering Mathematics
10th Edition
ISBN: 9780470458365
Author: Erwin Kreyszig
Publisher: Wiley, John & Sons, Incorporated
expand_more
expand_more
format_list_bulleted
Question
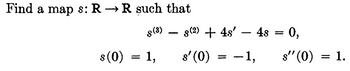
Transcribed Image Text:Find a map s: RR such that
S (3)
s(0)
= 1,
www.m
s(2) + 4s' - 4s = 0,
s'(0)
—1,
=
s'ʼ(0) = 1.
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution
Trending nowThis is a popular solution!
Step by stepSolved in 4 steps with 2 images

Knowledge Booster
Similar questions
- Q3. Using u = 1-2u u²e-u-u 2u-1 A) B) ²e+u 1-2u C) u²e-u-u D) E) X 1+2u u²e-u-u the ODE dyy²ex/y = x²-2xy du = xdx dx du = 1-2u u²e-¹+2u²-u X du =– xdx du = du =- dx X dx X dx becomesarrow_forward1) Given the mapping F(x,y,z)3(3e",xz sin 2y ,xy), v=(2,2,-1), using the definition, then the corollary (the Jacobian), compute the tangent map F., (v) at the point P-(-1,2,-2) ôf 2) Show that U:[S]- in R',where U, is the unit natural frame ôx, field and / is a real valued function on R'.arrow_forward9 c and darrow_forward
- 2. Let P denote the path that goes in straight lines from (1,1,1) to (-1,-1,1) to (-1,-1,-1) to (1,1,-1) and then back to (1,1, 1). 2xyz+z el +2 x²z+sin(z) Compute fp F. dr, where F(x, y, z) = Parrow_forward(i) Consider T: R³ R³ defined by T(T1, T2, T3) = (x₁, I₁+I₂, X₁ + x₂ + x3). If B = {₁, ₂, 3} and B' = {B₁, B2, B3}, where a₁ = (0, 1, 1), a₂ = (1,0,1), a3 = (1, 1,0), B₁ = (1,0,0), B₂ = (1,1,0), B3 = (1, 1, 1), what is the matrix [T]B-B?arrow_forward8) Evaluate F dr where F(r,U.2) (2yze )k and C is A curve with initial point 2e r(0)3(2,1,2) and final point r(1) ((0,2, 4). (Hint: Check if F is conservative irst)arrow_forward
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
 Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated
Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education
Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY
Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,


Advanced Engineering Mathematics
Advanced Math
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:9780073397924
Author:Steven C. Chapra Dr., Raymond P. Canale
Publisher:McGraw-Hill Education

Introductory Mathematics for Engineering Applicat...
Advanced Math
ISBN:9781118141809
Author:Nathan Klingbeil
Publisher:WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,

