Find a formula involving c and h for the local truncation error in the nth step if Euler's method is used. Consider the initial-value problem y'= 2x − 3y + 1, y(1) = 6. The analytic solution isy(x) = 11/12 + 3x +4/737279947 e-3(x - 1).

Given the IVP y′=2x−3y+1,y(1)=6.The analytic solution is .Formula:The local truncation error for the…

Answered: Find a formula involving c and h for…

Math

Advanced Math

Find a formula involving c and h for the local truncation error in the nth step if Euler's method is used

Advanced Engineering Mathematics

10th Edition

ISBN:9780470458365

Author:Erwin Kreyszig

Publisher:Erwin Kreyszig

Chapter2: Second-order Linear Odes

Section: Chapter Questions

Problem 1RQ

See similar textbooks

Related questions

Q: Bisection Solve for the root of f(x) = sin 4x if x₁ = −2 and XR = -1 Solve for the root of f(x) =…

A: note : As per our company guidelines we are supposed to answer ?️only the first question. Kindly…

Q: Given A(-2, 0) and B(2, 0), find a formula not containing radicals that expresses the fact that the…

A: Given A(-2,0), B(2,0)

Q: 3. Determine the equation of the quintic (5th degree) with single roots at -2, 3, and r, and a…

A: Let us find the quintic polynomial.

Q: Use (a) Fixed Point Iteration method (b) Newton-Rhapson method and (c) Secant Method to find the…

A: Solution:f(x)=x3-2x2-5a) Roots using fixed-point iteration method in the interval 1,4The initial…

Q: 2x²³²y" + 3xy + (2x-1)y=0

A: The given problem is to solve the given differential equation using forbenius method.

Q: Hind the cooffecPents + ax tb=0, where c = 3 anod x =-2 are tha roots a anod b f1om the oquatfon,

Q: Find the second approximation to the root of the equation x-4x-9 0using bisection method, if a, =…

A: Given: x3-4x-9=0 , a0=2, b0=3 f(x)=x3-4x-9 f(2)=23-4(2)-9 =8-8-9…

Q: 1. Given the function f (x) = 5x2 - x - 2, Determine the approximate root of the %3D function and…

A: We are authorized to answer one question at a time, since you have not mentioned which question you…

Q: how would you solve for x of the congruence equation? also why would (ax-12)/32 = 1 on the second…

Q: 2. Find the root of the following equation through the different methods, f(x) = x^3 − x − 1 a.…

A: note : As per our company guidelines we are supposed to answer ?️only first 3 sub-parts. Kindly…

Q: As the number of hours since you ate protein increases from 2 to 2.75 hours what is the average rate…

A: Given: At 2 hours B1=1100 mg/L At 2.75 hours B2=1025 mg/L

Q: The equation f(x)=ex -x has a single real root. Find it using the Bisection method (do at least 2…

A: To find : The root of the equation f(x) = ex - x using bisection method.

Q: e start at (0,0) and want to reach (6, 8). How many ways can we do this, assuming that we can only…

A: To determine the number of ways we can reach the point (6,8) if we start from (0,0).

Q: Assuming that the halving method starts with the interval [10,15], 10-30 relative How many steps…

Q: Find the second approximation to the root of the equation x-4x-9 = 0using bisection method, if a, =…

Q: B) Using two iterations of the Bisection Method, find the real root of the equation x²-x=6x with…

A: In Bisection Method, we find a root of f(x)=0, in an interval [a, b] as follows: (i) First we find…

Q: Q2: Use bisection method to determining the root of x³ - 10 = 0 .Assume that the root lies between 1…

A: The given function is f(x)=x3-10. Given: Root lies between 1 and 3. To find: The approximated root…

Q: f(x) — е -*(х2 -5х + 2) *(x²

A: Regula-Falsi method also known as the false position method uses the following formula to perform…

Q: solve the ODE using the Method of Undetermined Coefficients. Clearly label y, and y, and then state…

A: Given differential equation y''+6y'+5y=10x2+9x-9. The objective is to solve the differential…

Q: LAR널)] = Y(S) = [올 +2 Yi(s) = Y,(s) = (S+4)×2 블 +8S+20 N/A ( 52 ) ³²+ + 4 . √2 + 5 - S14 (S+4) e (5²…

A: To show:-

Q: use Rung-kutta method to so lve

A: It is given that y'=y with the initial condition y0=1. Comparing with the initial value problem…

Q: solve eiflerenticl equation. dy by Milne's methed with hzool and A dams-Moulton method with heol…

A: Since you've asked multiple questions, we are supposed to answer only the first question as per our…

Q: 6. Determine the four roots of the equation (z–2)*+(z+1)' = 0 and plot them on an Argand diagram.

Q: - (b) Without extracting the roots, determine which one is greater, VII-5 or, 19 – /13.

A: Solution

Q: 3.The iteration formula can be used to find (A) square root of A (B) cubic root of A (C) fourth root…

Q: Find all the value of (-8-8√31) 1/4 and (2+2;)²½/3

Q: 4. Let 0 ‡ b, c E Rn and define A = be E Rnxn. Show that (a) R(A) = span{b}, (b) N(A) = {x ≤ R" |…

A: (a) We are given that A = bcT where, c ∈ Rn So, R(A) = { Ax : x ∈ Rn×1 }…

Q: DO NOT USE A CALCULATOR IN THIS QUESTION. 10+2√5 The point (1-√√5, p) lies on the curve x² answer.…

A: given point 1-5, p and the curve y=10+25x2

Q: 2. Find the terminal point P (x, y) and the reference number determined by t=- Round the coordinates…

A: We have given t = -61×π/6 Terminal point P(x,y) is given by ( cos(t), sin(t) ) So we have…

Q: QI/ Fit a second order polynomial to the data in the following table : 10 5 11 3 4 6. 7 Y 4 8 13 9.…

A: The equation of the second-degree polynomial is given by the formula . Here, , and are the…

Question

Find a formula involving c and h for the local truncation error in the nth step if Euler's method is used.

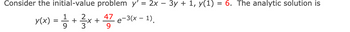
Consider the initial-value problem y'= 2x − 3y + 1, y(1) = 6. The analytic solution is
y(x) = 11/12 + 3x +4/73727
9
9
47 e-3(x - 1).

Expert Solution

This question has been solved!

Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.

SEE SOLUTION Check out a sample Q&A here

Step 1: Writing the given information

VIEW

Step 2: Finding the formula

VIEW

Solution

VIEW

Step by step

Solved in 3 steps with 6 images

SEE SOLUTION Check out a sample Q&A here

Follow-up Questions

Read through expert solutions to related follow-up questions below.

Follow-up Question

pproximate y(1.5) using

h = 0.1

and

h = 0.05

with Euler's method.

Solution

by Bartleby Expert

SEE SOLUTION

Follow-up Question

Find a bound for the local truncation error in each step if

h = 0.1

is used to approximate y(1.5)

Solution

by Bartleby Expert

SEE SOLUTION

Similar questions

Please finish this task in 45 min,ASAP,Use the half interval method and new raphson method
√(4-3.7)² + c-2-(-1))²- Vor0941 =VT. 67 tra 10. Find x. 12. Find PQ. 14. Find m/YWX. 10x-1 √85 +1.09 Vi7.64 14x-37 8x - 27 PO Р W (5x – 8) 2 S 11. Find x. 4x + 33 (9x-28)° R 13. Find Y 15.
Q.2) Use (a) fixed-point iteration and (b) the Newton-Raphson method to determine a root of Ax)=-x2+1.8x+2.5 using xo=5. Perform the computation until Ea is less than &=0.05%. Also perform an error check of your final answer.
Which of the following differential equations are ordinary? (Select all that apply) dy + 2 dx = cos t dt + sin 0 = 0 dt2 + cx + kx = 0 dt m yy + 2x = cos x
Q2 Needed to be solved correctly in 15 minutes in the order to get positive feedback please show me neat and clean work for it by hand solution needed
I need the answer as soon as possible
A value of x that satisfies the equation (1+ i)æ³ – 2i = 0 is: Select one: - V2(-1+i) (1+i) (-1+ i) /2(1+i) (1 – i)
Find the relative error and the number of significant digits in the approximation of π by 6pN
8) Use Newton's method to derive the ancient and long standing divide and average method for finding square roots by hand. It is also known as the Babylonian method. √r can be approximated by iterating X¡ +1² (x₁+1=1) using an initial guess x₁. Because √ is the positive solution to the equation x² - r = 0, derive the iterating formula above by simplifying the Newton's method formula using f(x)=x²-r. Note that since r is a constant, d/dx(r) = 0. 9) Use the divide and average method derived in Exercise 8 and modify your code from Exercise 5 (three times) to calculate the square roots of the following numbers to within 8 decimal places (a) r = 2 (b) r = 3 (c) r = 5 You do not need to include all of the code for these problems in the HW you turn in, as most of it will just be the same as number 5. However, write down the first 5 to 8 lines (depending on the language used) that define the function used, the initial guess, and the tolerance, along with the roots found in the end.