
Algebra and Trigonometry (6th Edition)
6th Edition
ISBN: 9780134463216
Author: Robert F. Blitzer
Publisher: PEARSON
expand_more
expand_more
format_list_bulleted
Question
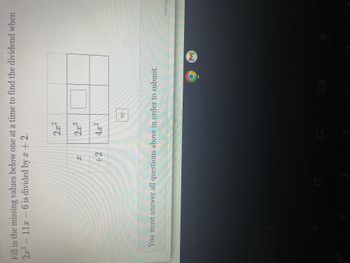
Transcribed Image Text:### Polynomial Long Division Practice
**Objective:**
Fill in the missing values below one at a time to find the dividend when \( 2x^3 - 11x - 6 \) is divided by \( x + 2 \).
#### Step-by-Step Instructions:
1. **Set up the long division:**
- **Dividend:** \( 2x^3 - 11x - 6 \)
- **Divisor:** \( x + 2 \)
The division process starts by dividing the leading term of the dividend by the leading term of the divisor.
2. **Place the first term in the quotient:**
- Divide \( 2x^3 \) by \( x \) to get \( 2x^2 \).
#### Fill in the missing terms in the grid below:
| | \( 2x^2 \) | |
|---------|------------|-----------|
| \( x \) | \( 2x^3 \) | |
| \( +2 \)| \( 4x^2 \) | |
#### Understanding the Diagram:
- The top left box contains the term \( 2x^2 \), which is the result of the first division step.
- The box directly beneath it contains \( 2x^3 \), which is the product of \( 2x^2 \) (from the quotient) and \( x \) (from the divisor).
- The bottom right corner box contains \( 4x^2 \), which is the product of \( 2x^2 \) (from the quotient) and \( 2 \) (from the divisor).
#### Next Steps:
- Subtract \( 2x^3 + 4x^2 \) from \( 2x^3 - 11x - 6 \).
- Continue the process by bringing down the next term and repeating the steps until the remainder is found or until there are no more terms to bring down.
Finally, click on the "try" button to check your answer.
**Note:** You must answer all questions above in order to submit your response.
---
By following this detailed step-by-step explanation, students can gain a deeper understanding of polynomial long division, reinforcing their algebra skills and ensuring they are well-prepared for more advanced topics.
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by stepSolved in 2 steps with 1 images

Knowledge Booster
Similar questions
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
 Algebra and Trigonometry (6th Edition)AlgebraISBN:9780134463216Author:Robert F. BlitzerPublisher:PEARSON
Algebra and Trigonometry (6th Edition)AlgebraISBN:9780134463216Author:Robert F. BlitzerPublisher:PEARSON Contemporary Abstract AlgebraAlgebraISBN:9781305657960Author:Joseph GallianPublisher:Cengage Learning
Contemporary Abstract AlgebraAlgebraISBN:9781305657960Author:Joseph GallianPublisher:Cengage Learning Linear Algebra: A Modern IntroductionAlgebraISBN:9781285463247Author:David PoolePublisher:Cengage Learning
Linear Algebra: A Modern IntroductionAlgebraISBN:9781285463247Author:David PoolePublisher:Cengage Learning Algebra And Trigonometry (11th Edition)AlgebraISBN:9780135163078Author:Michael SullivanPublisher:PEARSON
Algebra And Trigonometry (11th Edition)AlgebraISBN:9780135163078Author:Michael SullivanPublisher:PEARSON Introduction to Linear Algebra, Fifth EditionAlgebraISBN:9780980232776Author:Gilbert StrangPublisher:Wellesley-Cambridge Press
Introduction to Linear Algebra, Fifth EditionAlgebraISBN:9780980232776Author:Gilbert StrangPublisher:Wellesley-Cambridge Press College Algebra (Collegiate Math)AlgebraISBN:9780077836344Author:Julie Miller, Donna GerkenPublisher:McGraw-Hill Education
College Algebra (Collegiate Math)AlgebraISBN:9780077836344Author:Julie Miller, Donna GerkenPublisher:McGraw-Hill Education

Algebra and Trigonometry (6th Edition)
Algebra
ISBN:9780134463216
Author:Robert F. Blitzer
Publisher:PEARSON

Contemporary Abstract Algebra
Algebra
ISBN:9781305657960
Author:Joseph Gallian
Publisher:Cengage Learning

Linear Algebra: A Modern Introduction
Algebra
ISBN:9781285463247
Author:David Poole
Publisher:Cengage Learning

Algebra And Trigonometry (11th Edition)
Algebra
ISBN:9780135163078
Author:Michael Sullivan
Publisher:PEARSON

Introduction to Linear Algebra, Fifth Edition
Algebra
ISBN:9780980232776
Author:Gilbert Strang
Publisher:Wellesley-Cambridge Press

College Algebra (Collegiate Math)
Algebra
ISBN:9780077836344
Author:Julie Miller, Donna Gerken
Publisher:McGraw-Hill Education