Question
Can you do part A and B only
I don’t understand in A how they got v’-vT I’m getting the vice versa and I why is there a negative in the intergration

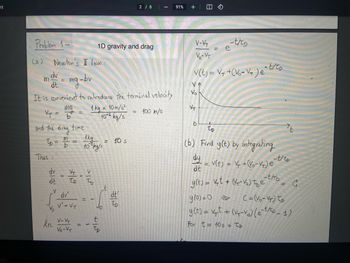
Transcribed Image Text:Problem 1-1D Gravity and Drag
A particle with mass m = 1 kg falls along the vertical y direction and is subject to a drag force FD = -bv, with b = 0.1 kg/s. Assume the y-axis directed downward,
so that the force of gravity is positive. The velocity of the particle at time t=0 is also along the y-direction, with positive (downward) value v(0) = Vo = 200
m/s, and the particle initial height is y(0) = 0. (a) Integrate Newton's second law and find the particle velocity v(t) as a function of time. Sketch the graph v(t).
(b) Find the particle position y(t) as a function of time. Estimate the particle's position at time t = 10 s (Hint: e¹¹ 0.37; in this problem, you can use g = 10
m/s²). (c) Consider the special case vo= 100 m/s. What happens in this case?
Problem 2-2D Collisions
marr me Before the collisions, particle B is at rest, while particle A moves along the x-axis
Transcribed Image Text:01
Problem 1-
(a)
m
Newton's I law:
-bv
ma
V₁ = b
dv
dt = mg-
It is convenient to introduce the terminal velocity
1 ка
x 10 m/s²
10 kg/s
and the drag time.
5 = 1/²
Thus :
dv
dt
V
V₂
=
V4
TD
dv'
V'- VT
ln V-VT
Vo-VT
=
1D gravity and drag
1kg
10 - ka/s
V
-
JO
t
هانا
=
10 s
2/8
dt'
ZD
=
I
100 m/s
91% +
V-V7
V-VT
VT
v(t)= V₁ +(V-V₁)e
V^
Vo
O
-t/TD
-t/TD
=.
TD
(b) Find y(t) by integrating
dy
dt
-t/tp
V(t) = +Vo-V+) e
-t/D
y(t) = + (-₂) e
+ G
y (0)=0 →
C=(V-VT) T
y(t) = vt + (₁-v₂) (e ths-1)
For t= 10s = p
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by stepSolved in 3 steps with 1 images

Follow-up Questions
Read through expert solutions to related follow-up questions below.
Follow-up Question
where is the explanation for part b??
Solution
by Bartleby Expert
Follow-up Questions
Read through expert solutions to related follow-up questions below.
Follow-up Question
where is the explanation for part b??
Solution
by Bartleby Expert
Knowledge Booster
Similar questions
- Car D is constantly 3.5 feet from the center of the race track and travels at a constant speed. The angle with a terminal ray passing through Car D sweeps out r radians per second. a. How many radians 0 does the angle sweep out in t seconds? Preview b. Define a function j that determines Car D's distance to the right of the center of the race track (in feet) as a function of the number of seconds t since the start of the race. j(t) = Preview c. The angle with a terminal ray passing through Car D must sweep out 27 radians to make one full rotation, and we know it sweeps out r radians per second. How many seconds will it take Car D to complete one full lap? | seconds Preview d. Sketch the graph of the function j below.arrow_forwardSolve d,e,f B) : v = -R w(sin(wt)î-cos(wt)j) C): v =( (sin(wt))^2 (cos(wt))^2)^1/2 For C I raised the entire equation to 1/2 because my keyboard doesn’t allow me to do square root but it is basically the Pythagorean theoremarrow_forwardA webassign.net/web/Student/Assignment-Responses/submit?dep=263123668tags=autosave#question4036946 0 M Gmail Maps YouTube Translate O Microsoft Office Ho... Check You: Paper f. Solve inequalities w. They can click and drag the black point at the outer end of the brown line to various points in the xy plane and see how its Cartesian and polar coordinates change in the readouts at the bottom. Note that 0 is defined as the angle measured in a direction counterclockwise from the positive x-axis. y (m) x (m) y =|-2,50| m -3.50 | m sin e- -0.58 4.30 m cos e = -0.81 216 degrees tan e- 0.71 Click bere to onen the simulation in a new window hparrow_forward
arrow_back_ios
arrow_forward_ios