
Trigonometry (11th Edition)
11th Edition
ISBN: 9780134217437
Author: Margaret L. Lial, John Hornsby, David I. Schneider, Callie Daniels
Publisher: PEARSON
expand_more
expand_more
format_list_bulleted
Topic Video
Question
Plz
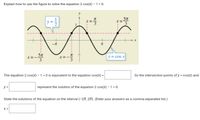
Transcribed Image Text:**How to Solve the Equation 2 cos(x) - 1 = 0 Using the Graph**
**Graph Explanation:**
The graph depicts the cosine function \( y = \cos(x) \), shown as a smooth, wavy line fluctuating between -1 and 1. Two labeled intersections indicate that \( y = \frac{1}{2} \) serves as a horizontal line intersecting the cosine curve.
Key points marked on the graph include:
- \( x = \frac{\pi}{3} \)
- \( x = \frac{5\pi}{3} \)
- \( x = -\frac{\pi}{3} \)
- \( x = -\frac{5\pi}{3} \)
**Explanation and Solution Steps:**
1. The equation \( 2 \cos(x) - 1 = 0 \) is equivalent to \( \cos(x) = \frac{1}{2} \).
2. Intersection points of \( y = \cos(x) \) and \( y = \frac{1}{2} \) are the solutions we seek.
3. Thus, the \( x \)-values corresponding to these intersections are the solutions to the equation.
**Formulation:**
- The equation \( \cos(x) = \frac{1}{2} \) gives the solution values for \( x \).
- The solutions within the interval \((-2\pi, 2\pi)\) are \( x = -\frac{5\pi}{3}, -\frac{\pi}{3}, \frac{\pi}{3}, \frac{5\pi}{3} \).
These solutions specify that the cosine curve intersects the horizontal line \( y = \frac{1}{2} \) at these points within the defined interval.
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution
Trending nowThis is a popular solution!
Step by stepSolved in 2 steps

Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, trigonometry and related others by exploring similar questions and additional content below.Similar questions
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
 Trigonometry (11th Edition)TrigonometryISBN:9780134217437Author:Margaret L. Lial, John Hornsby, David I. Schneider, Callie DanielsPublisher:PEARSON
Trigonometry (11th Edition)TrigonometryISBN:9780134217437Author:Margaret L. Lial, John Hornsby, David I. Schneider, Callie DanielsPublisher:PEARSON Trigonometry (MindTap Course List)TrigonometryISBN:9781305652224Author:Charles P. McKeague, Mark D. TurnerPublisher:Cengage Learning
Trigonometry (MindTap Course List)TrigonometryISBN:9781305652224Author:Charles P. McKeague, Mark D. TurnerPublisher:Cengage Learning
 Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning
Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning

Trigonometry (11th Edition)
Trigonometry
ISBN:9780134217437
Author:Margaret L. Lial, John Hornsby, David I. Schneider, Callie Daniels
Publisher:PEARSON

Trigonometry (MindTap Course List)
Trigonometry
ISBN:9781305652224
Author:Charles P. McKeague, Mark D. Turner
Publisher:Cengage Learning


Trigonometry (MindTap Course List)
Trigonometry
ISBN:9781337278461
Author:Ron Larson
Publisher:Cengage Learning