
Advanced Engineering Mathematics
10th Edition
ISBN: 9780470458365
Author: Erwin Kreyszig
Publisher: Wiley, John & Sons, Incorporated
expand_more
expand_more
format_list_bulleted
Question
Please do Exercise 17.2.18 part A,B,C,and D. Please show step by step and explain
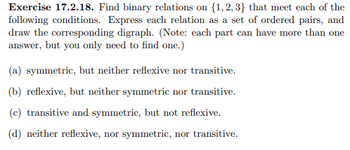
Transcribed Image Text:**Exercise 17.2.18**: Find binary relations on \(\{1, 2, 3\}\) that meet each of the following conditions. Express each relation as a set of ordered pairs, and draw the corresponding digraph. (Note: each part can have more than one answer, but you only need to find one.)
(a) Symmetric, but neither reflexive nor transitive.
(b) Reflexive, but neither symmetric nor transitive.
(c) Transitive and symmetric, but not reflexive.
(d) Neither reflexive, nor symmetric, nor transitive.
**Explanation:**
A binary relation from a set \(A\) to itself is a subset of \(A \times A\), the set of all ordered pairs of elements from \(A\). Below is a brief description of what each property means:
- **Symmetric**: If \((a, b)\) is in the relation, then \((b, a)\) must also be in the relation.
- **Reflexive**: Every element is related to itself; i.e., for each element \(a\) in the set, \((a, a)\) is in the relation.
- **Transitive**: If \((a, b)\) and \((b, c)\) are in the relation, then \((a, c)\) must be in the relation.
For each condition, a corresponding digraph (directed graph) can be drawn by representing each element as a vertex and each ordered pair \((a, b)\) as a directed edge from vertex \(a\) to vertex \(b\).
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution
Trending nowThis is a popular solution!
Step by stepSolved in 3 steps with 2 images

Knowledge Booster
Similar questions
- uta 8 D 24 (10727 20 A ADB is similar to ABEC Find what the line segment EC is? Is it 201arrow_forwardbard.com Question Completion Status: QUESTION 3 Which of the following relations are functions where y is a function of x? A. B. Click Save and Submit to stue hid si bmit. Click Save All Answers to save all answers. Sa ch F2 F3 F4 FSarrow_forwardEquation: Answer: -29802 RM 4. A city of 100,000 is having pollution problems and is decreasing in size 2% annually (every year). Find the population of this city in 100 years. Equation: Answer: 5. In 1982, the number of Starbucks was 5 shops. It has exponentially grown by 21% yearly. Let t= the number of years since 1982. Find an equation for this growth and find the number of Starbucks predicted in 2015. Equation: Answer: 6. A computer's value declines about 7% yearly. Sally bought a computer for $800 in 2005. How much is it worth in 2009.arrow_forward
Recommended textbooks for you
 Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated
Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education
Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY
Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,


Advanced Engineering Mathematics
Advanced Math
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:9780073397924
Author:Steven C. Chapra Dr., Raymond P. Canale
Publisher:McGraw-Hill Education

Introductory Mathematics for Engineering Applicat...
Advanced Math
ISBN:9781118141809
Author:Nathan Klingbeil
Publisher:WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,

