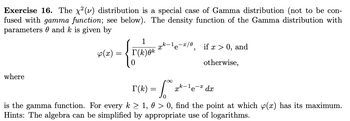
Exercise 16. The x²(v) distribution is a special case of Gamma distribution (not to be con- fused with gamma function; see below). The density function of the Gamma distribution with parameters and k is given by where 4(x) = { 0 1 r(k) ok T(k): xk-le-x/0 е = 2 ∞ • 10 201² is the gamma function. For every k ≥ 1, 0 > 0, find the point at which p(x) has its maximum. if x > 0, and otherwise, -x dx

The cumulative distribution function of the continuous uniform distribution between con- stants a and b is given by F(x) = P(X ≤ x) = x-a - a x b (a) The probability density function is f(x) = F(r). Find the form of f(x). (b) Find the derivative of f(x) for x = [a, b]. Is f(x) decreasing, increasing or flat in this region? (c) Does f(x) have a single maximum in the region [a,b]? If so, what is it, or if not, why not?

The lifetime, X, of a particular integrated circuit has an exponential distribution with rate of λ=0.5 per year. Thus, the density of X is: f(x,x) = 1 e-^x for 0 ≤ x ≤ ∞o, λ = 0.5. λ is what R calls rate. Hint: This is a problem involving the exponential distribution. Knowing the parameter for the distribution allows you to easily answer parts a,b,c and use the built-in R functions for the exponential distribution (dexp(), pexp(), qexp()) for other parts. Or (not recommended) you should be able to use the R integrate command with f(x) defined as above or with dexp() for all parts. d) What is the probability that X is greater than its expected value? e) What is the probability that X is > 5? f) What is the probability that X is> 10? g) What is the probability that X > 10 given that X > 5? h) What is the median of X? Please solution USING R script

Consider times at which a parent provisions nestlings, that is, the time be- tween arrivals of a parent carrying food for the nestlings. Suppose that the waiting time between arrivals of a parent bird follows the exponential density function f (t) = 0.6e-0,61 for t z 0 and f (t) = 0 for t < 0, where the waiting times are in minutes. (a) What is the mean waiting time? (b) Find the probability that the wait will be between 2 and 5 minutes. (c) Find the probability that the wait will be at least 3 minutes.