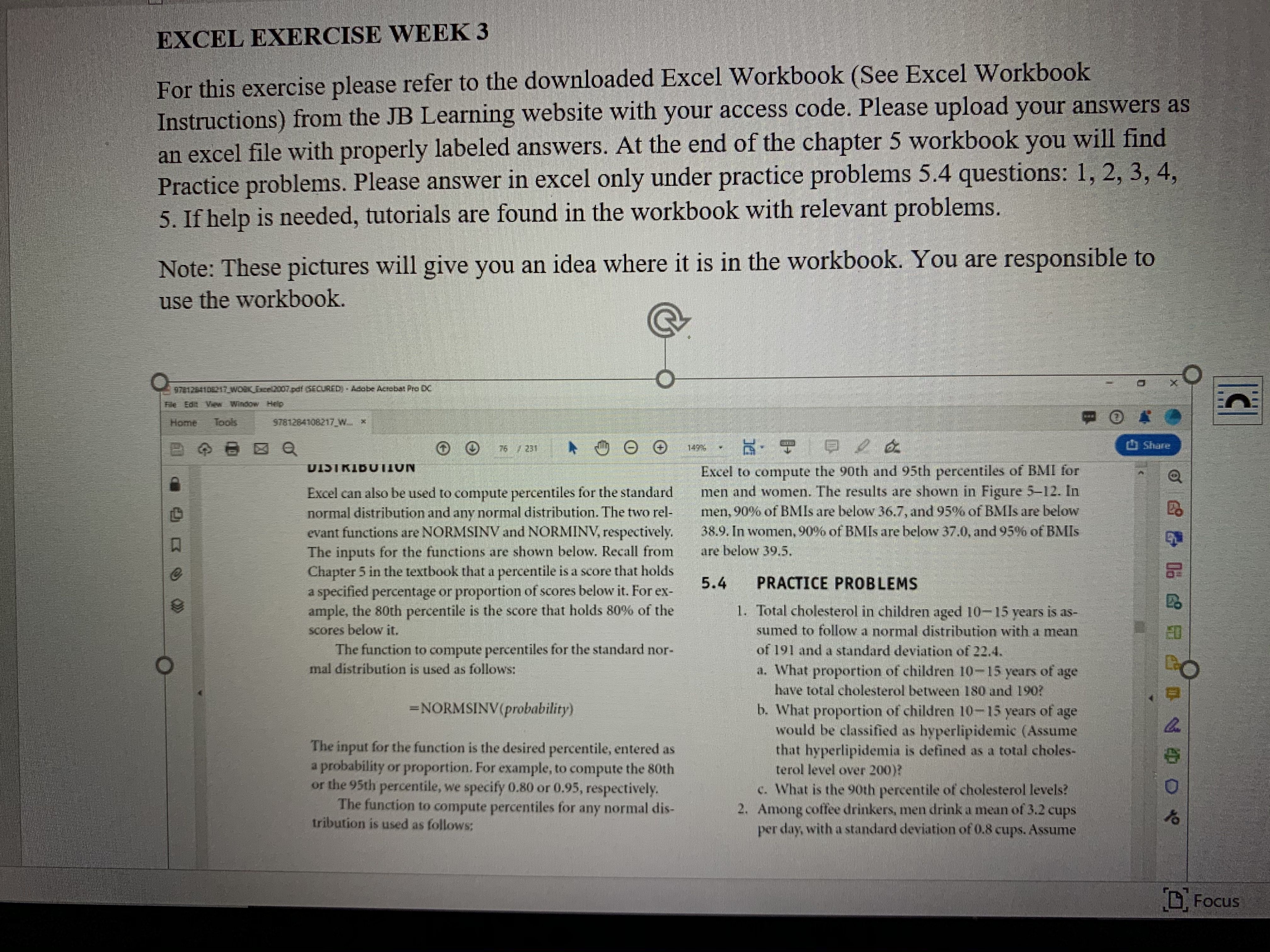
EXCEL EXERCISE WEEK 3 For this exercise please refer to the downloaded Excel Workbook (See Excel Workbook Instructions) from the JB Learning website with your access code. Please upload your answers as an excel file with properly labeled answers. At the end of the chapter 5 workbook you will find Practice problems. Please answer in excel only under practice problems 5.4 questions: 1, 2, 3, 4, 5. If help is needed, tutorials are found in the workbook with relevant problems. Note: These pictures will give you an idea where it is in the workbook. You are responsible to use the workbook. 978126410217 WOK Exce200/pdf.5ECURED)-Adabe Acreba Pro DC He Edit VaM Window Help. Home Tools 9781284108217/W * 149 Share Excel to compute the 90th and 95th percentiles of BMI for men and women. The results are shown in Figure 5-12. In men, 90% of BMIS are below 36.7, and 95% of BMIS are below 38.9. In women, 90% of BMIS are below 37.0, and 95% of BMIS UISTRIBUTION Excel can also be used to compute percentiles for the standard normal distribution and any normal distribution. The two rel- evant functions are NORMSINV and NORMINV, respectively. The inputs for the functions are shown below. Recall from Chapter 5 in the textbook that a percentile is a score that holds a specified percentage or proportion of scores below it. For ex- ample, the 80th percentile is the score that holds 80% of the scores below it. are below 39.5, 5.4 PRACTICE PROBLEMS 1. Total cholesterol in children aged 10-15 years is as- sumed to follow a normal distribution with a mean of 191 and a standard deviation of 22.4. a. What proportion of children 10-15 years of age have total cholesterol between 180 and 190? b. What proportion of children 10-15 years of age would be classified as hyperlipidemic (Assume that hyperlipidemia is defined as a total choles- terol level over 200)? ED The function to compute percentiles for the standard nor- mal distribution is used as follows: =NORMSINV(probability) The input for the function is the desired percentile, entered as a probability or proportion. For example, to compute the 80th or the 95th percentile, we specify 0.80 or 0.95, respectively. The function to compute percentiles for any normal dis- tribution is used as follows: c. What is the 9oth percentile of cholesterol levels? 2. Among coffee drinkers, men drink a mean of 3.2 cups per day, with a standard deviation of 0.8 cups. Assume OFocus

Evaluate I = | 5+ 3r3 dr

Perform the operation

I have attached the question in the image upload below. Please refer to the attachments for the question details. Please read the questions carefully and provide correct answers as well as the solutions. I appreciate your help, thank you.