
College Physics
11th Edition
ISBN: 9781305952300
Author: Raymond A. Serway, Chris Vuille
Publisher: Cengage Learning
expand_more
expand_more
format_list_bulleted
Question
The example below comes out of griffiths intro to electrodynamics. I was wondering if you can make a spherical and cylindrical problem like this example, one of each and use to divergence theorem to prove both sides of integrals from gauss divergence theorem. Hope this makes sense and thank
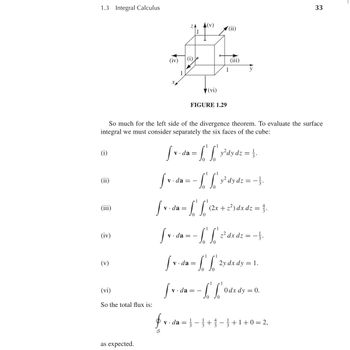
Transcribed Image Text:1.3 Integral Calculus
(iv)
1
ZA
1
(v)
(ii)
(vi)
FIGURE 1.29
1
So much for the left side of the divergence theorem. To evaluate the surface
integral we must consider separately the six faces of the cube:
(i)
(ii)
(iii)
(iv)
(v)
(vi)
So the total flux is:
1 1
[ v · da = f' f' y²dy dz = }
[ v. da
v.da
[ v · da = L
1 1
- S² S² y² dy dz = − } .
[ =
Sv.
$
S
v.da
L
1
1
(2x + z²) dx dz = 3.
1
- S' S² ² dx d² = − } .
L
1 1
[ v-da = L'S'
dz
S' S² 2y dx dy = 1.
Sv da = - S' S
L L
· ½
1
0dx dy = 0.
v da = -+-+1+0=2,
as expected.
33
33
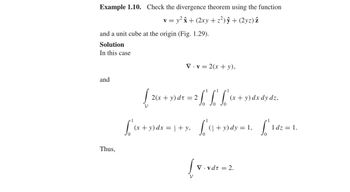
Transcribed Image Text:Example 1.10. Check the divergence theorem using the function
v = y²â+ (2xy + z²) ŷ + (2yz) z
and a unit cube at the origin (Fig. 1.29).
Solution
In this case
V.v=2(x + y),
and
1
{2(x + y) dt = 2 f f f (x +
y)dx dy dz.
1
S'
L'
=
(x + y) dx = + y,
= 1+y, f² (4 + y) dy =
1,
[ '1dz = 1.
0
Thus,
V.vdt = 2.
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by stepSolved in 2 steps

Knowledge Booster
Similar questions
- QEX. Need help with part B. Need theory and full detailed solution.arrow_forwardA 93.0 A current circulates around a 2.30-mm-diameter superconducting ring. Y Part A What is the ring's magnetic dipole moment? Express your answer in amperes times meter squared. ▸ View Available Hint(s) JAM Submit Part B 195] ΑΣΦΑ R What is the on-axis magnetic field strength 5.90 cm from the ring? Express your answer with the appropriate units. View Available Hint(s) Valua ? Unite A-marrow_forwardFor multipole expansion, under what conditions, if any, is it an exact expression, and under what conditions, if any, is it an approximation? In what limits/cases is it the most useful?arrow_forward
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
 College PhysicsPhysicsISBN:9781305952300Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning
College PhysicsPhysicsISBN:9781305952300Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning University Physics (14th Edition)PhysicsISBN:9780133969290Author:Hugh D. Young, Roger A. FreedmanPublisher:PEARSON
University Physics (14th Edition)PhysicsISBN:9780133969290Author:Hugh D. Young, Roger A. FreedmanPublisher:PEARSON Introduction To Quantum MechanicsPhysicsISBN:9781107189638Author:Griffiths, David J., Schroeter, Darrell F.Publisher:Cambridge University Press
Introduction To Quantum MechanicsPhysicsISBN:9781107189638Author:Griffiths, David J., Schroeter, Darrell F.Publisher:Cambridge University Press Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Lecture- Tutorials for Introductory AstronomyPhysicsISBN:9780321820464Author:Edward E. Prather, Tim P. Slater, Jeff P. Adams, Gina BrissendenPublisher:Addison-Wesley
Lecture- Tutorials for Introductory AstronomyPhysicsISBN:9780321820464Author:Edward E. Prather, Tim P. Slater, Jeff P. Adams, Gina BrissendenPublisher:Addison-Wesley College Physics: A Strategic Approach (4th Editio...PhysicsISBN:9780134609034Author:Randall D. Knight (Professor Emeritus), Brian Jones, Stuart FieldPublisher:PEARSON
College Physics: A Strategic Approach (4th Editio...PhysicsISBN:9780134609034Author:Randall D. Knight (Professor Emeritus), Brian Jones, Stuart FieldPublisher:PEARSON

College Physics
Physics
ISBN:9781305952300
Author:Raymond A. Serway, Chris Vuille
Publisher:Cengage Learning

University Physics (14th Edition)
Physics
ISBN:9780133969290
Author:Hugh D. Young, Roger A. Freedman
Publisher:PEARSON

Introduction To Quantum Mechanics
Physics
ISBN:9781107189638
Author:Griffiths, David J., Schroeter, Darrell F.
Publisher:Cambridge University Press

Physics for Scientists and Engineers
Physics
ISBN:9781337553278
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning

Lecture- Tutorials for Introductory Astronomy
Physics
ISBN:9780321820464
Author:Edward E. Prather, Tim P. Slater, Jeff P. Adams, Gina Brissenden
Publisher:Addison-Wesley

College Physics: A Strategic Approach (4th Editio...
Physics
ISBN:9780134609034
Author:Randall D. Knight (Professor Emeritus), Brian Jones, Stuart Field
Publisher:PEARSON