Advanced Engineering Mathematics
Advanced Engineering Mathematics
10th Edition
ISBN: 9780470458365
Author: Erwin Kreyszig
Publisher: Wiley, John & Sons, Incorporated
Bartleby Related Questions Icon

Related questions

Q: 2.0xl 794.781 こ 20
A:
Q: The number 6545 can be written as a product of a pair of positive two-digit numbers, what are these…
A: solution:-The given number =65456545=5×7×11×17the two numbers are two digit numberthenthe number…
Q: not rounded to 4 decimals as
A: The mean is 64,271 and the standard deviation is 17,329.
Q: 5475
A: Simplification
Q: Write a number less than 900 that rounds to 900 when rounding to the nearest hundred. Wh
A: Any number between 851 to 899
Q: Evaluate fi frydzdy
A:
Q: What is the number of months?
A: 12
Q: 404÷133= %3D
A:
Q: Perform the following computation using your calculator: (report your answer to 4 significant digits…
A:
Q: what is 1 hour to 72 minutes in simplest form
A: what is 1 hour to 72 minutes in simplest form
Q: How to divide powers
A: Formula used:
Q: Calculate Z0.2
A:
Q: calculate logs To,125
A:
Q: 100+50097=
A: Given:   100+50097
Q: Se* 5x dx .4 2
A: on solving this we get the
Q: Is that 1 dollar per month or 1 months per dollar
A:
Q: xedx
A: Solve by using limit
Q: Please show the work for 1524 Divided by 6?
A:
Q: Verify that an acre contains 43,560 square feet.
A: Here we have to verify that an acre contains 43,560 square feet.
Question

The numbers in the red box will have annotations below

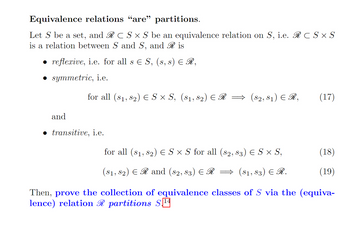
Equivalence relations “are" partitions. Let S be a set, and RC S × S be an equivalence relation on S, i.e. RC S × S is a relation between S and S, and Ris • reflexive, i.e. for all s € S, (s, s) ≤ R, • symmetric, i.e. and for all (8₁, 82) € S × S, (S1, S2) ≤ R transitive, i.e. ($2,81) € R, for all (8₁, 82) € S × S for all (s2, 83) € S × S, (81, 82) € R and (82, 83) € R ($1,$3) € R. (17) (18) (19) Then, prove the collection of equivalence classes of S via the (equiva- lence) relation R partitions S1
expand button
Transcribed Image Text:Equivalence relations “are" partitions. Let S be a set, and RC S × S be an equivalence relation on S, i.e. RC S × S is a relation between S and S, and Ris • reflexive, i.e. for all s € S, (s, s) ≤ R, • symmetric, i.e. and for all (8₁, 82) € S × S, (S1, S2) ≤ R transitive, i.e. ($2,81) € R, for all (8₁, 82) € S × S for all (s2, 83) € S × S, (81, 82) € R and (82, 83) € R ($1,$3) € R. (17) (18) (19) Then, prove the collection of equivalence classes of S via the (equiva- lence) relation R partitions S1
14Recall an equivalence class of S via the (equivalence) relation R is a subset of S, consisting of elements, all of whom are related to one another (by R). More precisely (at the expense of intuition!), an equivalence class of S via the (equivalence) relation R is a subset (20) TCS such that there exists t € S such that T = :{s € S|(s, t) ≤ R}. We may choose, for each equivalence class T of S via the (equivalence) relation R, a repre- sentative t € T, i.e. a specific element t € T to which all other elements of T are equivalent via R, i.e. a specific element t € T such that for all s € T, (s, t) € R. Having selected a representative, t, for an equivalence class T of S via the (equivalence) relation R, we may denote T instead, more suggestively, by [t], which signifies “all of the elements of S which are equivalent to t via the (equivalence) relation R.”" - For example, let S := Z × Z − {(0,0)} =: Zײ – {(0,0)} and R := {((x, y), (z, w)) € Sx S|xw = yz} C S x S. We have shown (and you should check once more!) RC S x S is an equivalence relation on S. The set T := {..., (-3, −6), (−2, −4), (−1, −2), (1, 2), (2, 4), (3, 6), ...} (21) is an equivalence class of S via the (equivalence) relation R, and happens to be the equiv- alence class of t := (1, 2) (ort := (−3, −6), or t := (−2, −4), or t := (−1, −2), or t := : (2,4), or t := (3,6), etc.) Remind yourself that the equivalence classes of S via the (equivalence) relation in this specific example do indeed partition S (into a collection of disjoint lines).
expand button
Transcribed Image Text:14Recall an equivalence class of S via the (equivalence) relation R is a subset of S, consisting of elements, all of whom are related to one another (by R). More precisely (at the expense of intuition!), an equivalence class of S via the (equivalence) relation R is a subset (20) TCS such that there exists t € S such that T = :{s € S|(s, t) ≤ R}. We may choose, for each equivalence class T of S via the (equivalence) relation R, a repre- sentative t € T, i.e. a specific element t € T to which all other elements of T are equivalent via R, i.e. a specific element t € T such that for all s € T, (s, t) € R. Having selected a representative, t, for an equivalence class T of S via the (equivalence) relation R, we may denote T instead, more suggestively, by [t], which signifies “all of the elements of S which are equivalent to t via the (equivalence) relation R.”" - For example, let S := Z × Z − {(0,0)} =: Zײ – {(0,0)} and R := {((x, y), (z, w)) € Sx S|xw = yz} C S x S. We have shown (and you should check once more!) RC S x S is an equivalence relation on S. The set T := {..., (-3, −6), (−2, −4), (−1, −2), (1, 2), (2, 4), (3, 6), ...} (21) is an equivalence class of S via the (equivalence) relation R, and happens to be the equiv- alence class of t := (1, 2) (ort := (−3, −6), or t := (−2, −4), or t := (−1, −2), or t := : (2,4), or t := (3,6), etc.) Remind yourself that the equivalence classes of S via the (equivalence) relation in this specific example do indeed partition S (into a collection of disjoint lines).
Expert Solution
Check Mark
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
bartleby
This is a popular solution
See solutionCheck out a sample Q&A here
Step 1: We are using the definition of equivalence class.
VIEW
Step 2: We are using the properties of equivalence class.
VIEW
Solution
VIEW
bartleby

Trending nowThis is a popular solution!

bartleby

Step by stepSolved in 3 steps with 3 images

See solution
Check out a sample Q&A here
Blurred answer
Knowledge Booster
Background pattern image
Similar questions
Recommended textbooks for you
Text book image
Advanced Engineering Mathematics
Advanced Math
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Wiley, John & Sons, Incorporated
Text book image
Numerical Methods for Engineers
Advanced Math
ISBN:9780073397924
Author:Steven C. Chapra Dr., Raymond P. Canale
Publisher:McGraw-Hill Education
Text book image
Introductory Mathematics for Engineering Applicat...
Advanced Math
ISBN:9781118141809
Author:Nathan Klingbeil
Publisher:WILEY
Text book image
Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,
Text book image
Basic Technical Mathematics
Advanced Math
ISBN:9780134437705
Author:Washington
Publisher:PEARSON
Text book image
Topology
Advanced Math
ISBN:9780134689517
Author:Munkres, James R.
Publisher:Pearson,
SEE MORE TEXTBOOKS