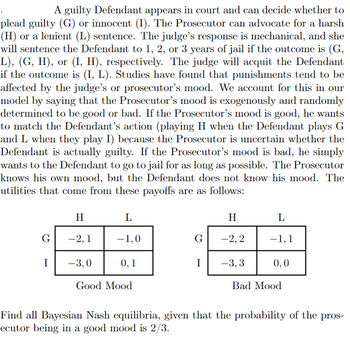
A guilty Defendant appears in court and can decide whether to plead guilty (G) or innocent (I). The Prosecutor can advocate for a harsh (H) or a lenient (L) sentence. The judge's response is mechanical, and she will sentence the Defendant to 1, 2, or 3 years of jail if the outcome is (G, L), (G, H), or (I, H), respectively. The judge will acquit the Defendant if the outcome is (I, L). Studies have found that punishments tend to be affected by the judge's or prosecutor's mood. We account for this in our model by saying that the Prosecutor's mood is exogenously and randomly determined to be good or bad. If the Prosecutor's mood is good, he wants to match the Defendant's action (playing H when the Defendant plays G and L when they play I) because the Prosecutor is uncertain whether the Defendant is actually guilty. If the Prosecutor's mood is bad, he simply wants to the Defendant to go to jail for as long as possible. The Prosecutor knows his own mood, but the Defendant does not know his mood. The utilities that come from these payoffs are as follows: G I H -2,1 -3,0 L -1,0 0,1 Good Mood G I H -2,2 -3,3 L -1,1 0,0 Bad Mood Find all Bayesian Nash equilibria, given that the probability of the pros- ecutor being in a good mood is 2/3.

High quality information gruarantees that every decisions made will be a successful one that benefits the organization

how  you know what c value to look at

Explain ZONING AND LAND USE?