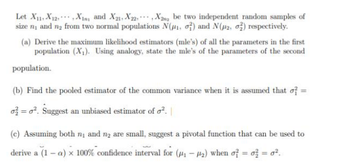
Let X₁, X12, Xim and X21, X22X2n be two independent random samples ofsize n, and n₂ from two normal populations N(₁, 0) and N(2, 2) respectively.(a) Derive the maximum likelihood estimators (mle's) of all the parameters in the firstpopulation (X₁). Using analogy, state the mle's of the parameters of the secondpopulation.(b) Find the pooled estimator of the common variance when it is assumed that of =o=0². Suggest an unbiased estimator of o².(c) Assuming both n₁ and n₂ are small, suggest a pivotal function that can be used toderive a (1-a) x 100% confidence interval for (#4₁-4₂) when o² = 0 = 0².

Step: 1Let be two independent random samples of size n1 and n2 from two normal populations…

Answered: e maximum likelihood estimators (mle's)…

A First Course in Probability (10th Edition)

10th Edition

ISBN:9780134753119

Author:Sheldon Ross

Publisher:Sheldon Ross

Chapter1: Combinatorial Analysis

Section: Chapter Questions

Problem 1.1P: a. How many different 7-place license plates are possible if the first 2 places are for letters and...

See similar textbooks

Similar questions

Suppose that the general fertility rate, g f rt , is following an AR(1) process shown in the image below A) if y1<1, what is the expression for the mean and variance of g f t rt showing any assumptions that were made . b) suppose that Var (€|gfrt-1)= as shown in the image below. explain why you may not obtain a best linear unbiased estimator of y0 and y1 by estimating (3) using OLS.
Please help me for Question 1
A population is distributed with a known standard deviation, σ = 18 units. A random sample of size 35 is obtained from this population. The mean of this sample is 70. True or False: Since the sample size is greater than 25 the distribution of sample means from this population should be approximately normally distributed. What is the lower limit of the 95% confidence interval for the population mean μ? (2 dp) What is the upper limit of the 95% confidence interval for the population mean μ? (2 dp). Based on your confidence interval, would you believe that the true mean of this population could be 75?
Let X1, X2, .. ., Xn be a random sample from a normal distribution with mean u and variance o?. Find an estimator for o? using the method of moments. Is this estimator bi- ased/unbiased? Is it consistent?
A random sample of 25 students obtained a mean of 78 and a variance of s2 = 15 on a college entrance exam in engineering. Assuming the scores are normally distributed, construct a 98% confidence interval of σ2
In a trivariate distribution, it 112= 0.7, 113 = 0.61 and 223 = 0.4 find all the multiple correlation coefficients. Also obtain the standard of estimates 01.23 61:23, 02:13 and 53:12 given that: 52= 1:06, 62 = 2.15 and 3= 3.46.
(e) Show that the sample mean of a simple random sample (drawn without replacement from a normal population) is dis- tributed normally with mean u and variance -² (1--=-1). n where N and n are population and sample sizes respectively. 4 In the 1 1 TI
I need help with this one, please. I need it to be step-by-step please for me to understand.
An experiment is conducted to compare the maximum load capacity in tons (the maximum weight that can be tolerated without breaking) for two alloys A and B It is Kknown that the two standard deviations in load capacity are equal at 7 tons each. The experiment is conducted on 50 specimens of each alloy (A and B) and the results are X = 75.8. X = 71.8, and X-X=4. The manufacturers of alloy A are convinced that this evidence shows conclusively that Hug and strongly supports the claim that their alloy is superior. Manufacturers of alloy 8 claim that the experiment could easily have given X-X 4 even if the two population means are equal. Complete parts (a) and (b) below. Click here to view page 1 of the standard normal distribution table. Click here to view page 2 of the standard normal distribution table. (a) Make an argument that manufacturers of alloy B are wrong. Do it by computing P(XA-XB41 PA-HB) P(XA-X > 4) - @
Suppose X1,..., Xn is a random sample from an exponential distribution with mean e. If X = 17.9 with n = 50, find (a) a one-sided 95% confidence interval for 0, and (b) a two-sided 95% confidence interval for 0.
If a is a hypergeometric random bie, compute the mean the variance, 'he standard deviation and the population coection factorfach the following cases: : (a) N= 8, M = 15, n = 13, r = 1 H = 195/8 o2 = 975/64 population correction factor= (b) N = 7, M = 8, n = 14, x = 7 H= 16 population correction factor= (c) N = 14, M = 15, n = 11, x = 5 population correction factor= (d) N = 10, M = 15, n = 6, x = 5 population correction factor=
A random sample of 10 observations from population A has sample mean of 152.3 and a sample standard deviation of 1.83. Another random sample of 8 observations from population B has a sample standard deviation of 1.94. Assuming equal variances in those two populations, a 99% confidence interval for μA − μB is (-0.19, 4.99), where μA is the mean in population A and μB is the mean in population B. (a) What is the sample mean of the observations from population B? (b) If we test H0 : μA ≤ μB against Ha : μA > μB, using α = 0.02, what is your conclusion?

SEE MORE QUESTIONS

Recommended textbooks for you

A First Course in Probability (10th Edition)

Probability

ISBN:

9780134753119

Author:

Sheldon Ross

Publisher:

PEARSON

A First Course in Probability

Probability

ISBN:

9780321794772

Author:

Sheldon Ross

Publisher:

PEARSON

A First Course in Probability (10th Edition)

Probability

ISBN:

9780134753119

Author:

Sheldon Ross

Publisher:

PEARSON

A First Course in Probability

Probability

ISBN:

9780321794772

Author:

Sheldon Ross

Publisher:

PEARSON

SEE MORE TEXTBOOKS