
Advanced Engineering Mathematics
10th Edition
ISBN: 9780470458365
Author: Erwin Kreyszig
Publisher: Wiley, John & Sons, Incorporated
expand_more
expand_more
format_list_bulleted
Question
Draw approx. solution for the directional field for the first order with the initial condition y(0) = -1.8
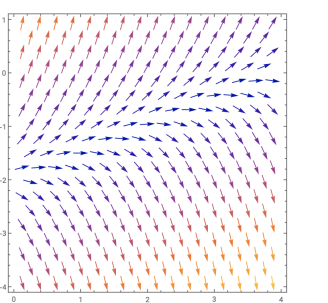
Transcribed Image Text:This image represents a vector field plot, which is a way to depict vector functions that assign a vector to every point in a subset of space. The plot displays vectors as arrows directing and indicating the magnitude and direction at various points on a two-dimensional grid.
### Description
- **Axes**: The plot has two axes, with the horizontal axis (x-axis) ranging from 0 to 4 and the vertical axis (y-axis) ranging from -4 to 1.
- **Arrows**: Each arrow in the plot represents a vector. The direction of the arrow indicates the direction of the vector at that point, and the length and color of the arrow indicate the magnitude of the vector.
- The vectors above the y-axis between y = -1 and y = 1 appear to have a horizontal direction.
- As we move away from the center line (y=0), the direction of the arrows becomes more diagonal.
- **Color Gradient**: The colors of the vectors vary from blue to red as the vectors change their direction. Typically, blue represents lower magnitudes, while red represents higher magnitudes, though the actual relationship depends on the specific context of the vector field.
These plots are useful in many fields such as physics, engineering, and meteorology to visualize fields like velocity fields in fluid dynamics, electromagnetic fields, or gradient fields. This specific vector field seems to demonstrate a transitioning behavior in terms of vector directionality and magnitude.
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by stepSolved in 3 steps with 3 images

Knowledge Booster
Similar questions
- Calculate the first derivative of a general cubic Bezier curve .We have a Bezier curve C1, that is described from the control points P0(0,0) , P1(3,3,),P2(6,3), and P3(8,1), and curve C2 that is described from the control point P4,P5,P6(11,5) and P7(15,9). Draw the curve C1 approximately and a control polygonarrow_forwardCompute the gradients of each of the following functions: o = xyz & = ln [(x² - y²)-¹/2] r = || = √√√x² + y² + x² 1/r Note: Remember that your tutorial has Hints in it, at the end of each chapter. If you're stuck, they are often quite useful! • If the answer is a scalar, you can just type it in the box (using the Calcpad if you like, or using / for fractions, ^ for exponents, shift and - for subscripts, etc.) For multiplication, you can either leave a space, or use *. So x*x=xx= x², Note that this is not the same as xx without a space; that will get read as an entirely different variable! • If you need to enter a vector, enter an ordered list of components, so for A you can enter either A = (A₂, Ay, A₂) = {A₂, Ay, A₂}. Note that the system isn't great with multiplying through by overall factors, so it's better not to write e.g. Vo= V = Vr = V= (2A, 2A, 2A₂).arrow_forward
Recommended textbooks for you
 Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated
Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education
Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY
Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,


Advanced Engineering Mathematics
Advanced Math
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:9780073397924
Author:Steven C. Chapra Dr., Raymond P. Canale
Publisher:McGraw-Hill Education

Introductory Mathematics for Engineering Applicat...
Advanced Math
ISBN:9781118141809
Author:Nathan Klingbeil
Publisher:WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,

