
Advanced Engineering Mathematics
10th Edition
ISBN: 9780470458365
Author: Erwin Kreyszig
Publisher: Wiley, John & Sons, Incorporated
expand_more
expand_more
format_list_bulleted
Question

Transcribed Image Text:1. Creating the Objective Function:
The cost to build any section of the shed is the unit cost (2) to build a section multiplied by the
number of square feet of a section. We have the unit costs, so now we need to find the square footage
of each section in terms of x and/or y.
a. The base:
Depth =
c. The roof:
Depth =
Length =
b. The sides:
1. Left side and right side. Since these sides have the same dimensions, find the area and cost of
the left side and then double it.
Depth =
Total Cost =
Height =
(cost of base)
Area =
2. Front side and back side. Since these sides have the same dimensions, find the area and cost of
the front side and then double it.
Length =
Height =
Cost =
Length =
Area of both =
Cost of both =
Area of both =
Cost of both
Area =
d. Now we can write our cost equation in terms of x and y.
The objective function is the total cost to build the shed. Total cost is the cost to build the base and
the sides and the roof. Since the cost to build the left and right sides are the same (and the front and
back) combine those cost terms to get:
Cost =
(cost of left and right) (cost of front and back) (cost of roof)
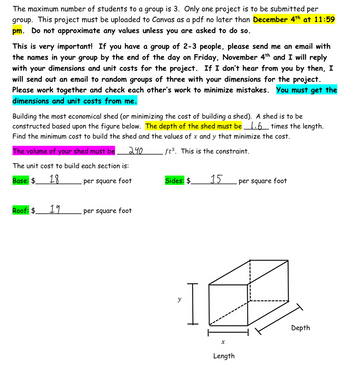
Transcribed Image Text:The maximum number of students to a group is 3. Only one project is to be submitted per
group. This project must be uploaded to Canvas as a pdf no later than December 4th at 11:59
pm. Do not approximate any values unless you are asked to do so.
This is very important! If you have a group of 2-3 people, please send me an email with
the names in your group by the end of the day on Friday, November 4th and I will reply
with your dimensions and unit costs for the project. If I don't hear from you by then, I
will send out an email to random groups of three with your dimensions for the project.
Please work together and check each other's work to minimize mistakes. You must get the
dimensions and unit costs from me.
Building the most economical shed (or minimizing the cost of building a shed). A shed is to be
constructed based upon the figure below. The depth of the shed must be 1.6 times the length.
Find the minimum cost to build the shed and the values of x and y that minimize the cost.
240
ft³. This is the constraint.
The volume of your shed must be
The unit cost to build each section is:
Base: $
18
per square foot
Roof: $
19
per square foot
Sides: $
I
15
X
Length
per square foot
Depth
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution
Trending nowThis is a popular solution!
Step by stepSolved in 3 steps

Follow-up Questions
Read through expert solutions to related follow-up questions below.
Follow-up Question
Could you also assist me with these steps as well? I just have a lot of trouble understanding what its exactly asking of me

Transcribed Image Text:3. The Critical Values of the Objective Function:
a. Now we need to find our derivative, C'(x). Again, depending upon your numbers, a fractional
coefficient might contain a decimal. DO NOT APPROXIMATE. I would use the MATH FRAC feature on a
graphing calculator to convert this quantity to a fraction.
(x)
b. and then find any critical values on our domain. Show the work below to obtain your critical value.
State the exact value and if you need to approximate, do so to 4 decimal places.
Critical value (exact): x =
Critical value (approximate to 4 decimal places): x =
4. Verification That Our Critical Value is the Location of the Absolute Minimum Cost:
Verification is a required part of all optimization problems. Recall that the First and Second Derivative
Tests are a test for local extrema, so we have to do a little work to make sure that our critical value is
the location of the absolute minimum cost. Since our domain is the open interval (0, ∞), you have to do
the following:

Transcribed Image Text:After combining like terms: Total Cost =
240
The constraint is that the volume of the shed must be
of our shed in terms of x and y is: (Recall volume of a box is length times width times height)
(our volume) (length times width times height)
Solve this volume equation for y:
y =
C(x) =
ft³. The formula for the volume
Replace y in your cost function to make it a function of x only. When you are done simplifying the cost
equation you should get two terms: one with an x², one with an x in the denominator of a fraction.
Depending upon your numbers, the coefficient of the quantity with x in the denominator might contain a
decimal. DO NOT APPROXIMATE. I would use the MATH FRAC feature on a graphing calculator to
convert this quantity to a fraction. Show your work below.
2. The domain of the Objective Function:
The domain in this problem represents the possible lengths of the base of our shed that can created
with our fixed volume. Since our shed can be either very wide (large value of x) and short or very thin
(small value of x) and tall, the domain of x is (0,∞).
Solution
by Bartleby Expert
Follow-up Question
Do you think that it's possible to do this problem without the Lagrange multiplier? I haven't learned about that concept yet
Solution
by Bartleby Expert
Follow-up Questions
Read through expert solutions to related follow-up questions below.
Follow-up Question
Could you also assist me with these steps as well? I just have a lot of trouble understanding what its exactly asking of me

Transcribed Image Text:3. The Critical Values of the Objective Function:
a. Now we need to find our derivative, C'(x). Again, depending upon your numbers, a fractional
coefficient might contain a decimal. DO NOT APPROXIMATE. I would use the MATH FRAC feature on a
graphing calculator to convert this quantity to a fraction.
(x)
b. and then find any critical values on our domain. Show the work below to obtain your critical value.
State the exact value and if you need to approximate, do so to 4 decimal places.
Critical value (exact): x =
Critical value (approximate to 4 decimal places): x =
4. Verification That Our Critical Value is the Location of the Absolute Minimum Cost:
Verification is a required part of all optimization problems. Recall that the First and Second Derivative
Tests are a test for local extrema, so we have to do a little work to make sure that our critical value is
the location of the absolute minimum cost. Since our domain is the open interval (0, ∞), you have to do
the following:

Transcribed Image Text:After combining like terms: Total Cost =
240
The constraint is that the volume of the shed must be
of our shed in terms of x and y is: (Recall volume of a box is length times width times height)
(our volume) (length times width times height)
Solve this volume equation for y:
y =
C(x) =
ft³. The formula for the volume
Replace y in your cost function to make it a function of x only. When you are done simplifying the cost
equation you should get two terms: one with an x², one with an x in the denominator of a fraction.
Depending upon your numbers, the coefficient of the quantity with x in the denominator might contain a
decimal. DO NOT APPROXIMATE. I would use the MATH FRAC feature on a graphing calculator to
convert this quantity to a fraction. Show your work below.
2. The domain of the Objective Function:
The domain in this problem represents the possible lengths of the base of our shed that can created
with our fixed volume. Since our shed can be either very wide (large value of x) and short or very thin
(small value of x) and tall, the domain of x is (0,∞).
Solution
by Bartleby Expert
Follow-up Question
Do you think that it's possible to do this problem without the Lagrange multiplier? I haven't learned about that concept yet
Solution
by Bartleby Expert
Knowledge Booster
Similar questions
- Assume that a cylinder has a fixed volume of 125 m³ with an open top. Find the radius and height of the cylinder that can minimize its surface area. The surface area of the cylinder is A = (radius)2 + 2 radius height The volume of the cylinder = (radius)². height. → radius heightarrow_forwardSuppose you use exactly 222 feet of wire fencing to enclose a rectangular grazing area for your goats. If the grazing area is half as long as it is wide, find its dimensions. Length: Width: FINISHarrow_forwardPlease don't provide handwritten solution ....arrow_forward
Recommended textbooks for you
 Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated
Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education
Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY
Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,


Advanced Engineering Mathematics
Advanced Math
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:9780073397924
Author:Steven C. Chapra Dr., Raymond P. Canale
Publisher:McGraw-Hill Education

Introductory Mathematics for Engineering Applicat...
Advanced Math
ISBN:9781118141809
Author:Nathan Klingbeil
Publisher:WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,

