Determine the speed of the block with a mass of 0.60 kg after it has fallen 145 cm. The sphere is a shell with M = 4.5 kg and R = 8.5 cm. The pully has a rotational inertia of 0.0030 kg m2 and r = 5.0 cm. Use g = 9.8 m/s/s. Use conservation of energy.
Determine the speed of the block with a mass of 0.60 kg after it has fallen 145 cm. The sphere is a shell with M = 4.5 kg and R = 8.5 cm. The pully has a rotational inertia of 0.0030 kg m2 and r = 5.0 cm. Use g = 9.8 m/s/s. Use conservation of energy.
Related questions
Question
Determine the speed of the block with a mass of 0.60 kg after it has fallen 145 cm. The sphere is a shell with M = 4.5 kg and R = 8.5 cm. The pully has a rotational inertia of 0.0030 kg m2 and r = 5.0 cm. Use g = 9.8 m/s/s. Use conservation of energy.
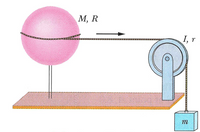
Transcribed Image Text:М, R
I, r
m
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by step
Solved in 3 steps with 3 images
