
Calculus: Early Transcendentals
8th Edition
ISBN: 9781285741550
Author: James Stewart
Publisher: Cengage Learning
expand_more
expand_more
format_list_bulleted
Question
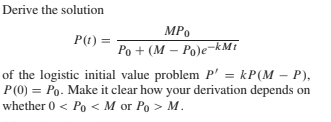
Transcribed Image Text:Derive the solution
P(t) =
MPo
Ро + (М — Роје-
of the logistic initial value problem P' = kP(M – P),
-kMt
P(0) = Po. Make it clear how your derivation depends on
whether 0 < Po <M or Po > M.
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution
Trending nowThis is a popular solution!
Step by stepSolved in 5 steps with 6 images

Knowledge Booster
Similar questions
- Assume A population of tigers in a reserve grows according to the logistic differential equation. There are initially 30 tigers released but grown to a population of 50 after one year. It is estimated the reserve cannot support more than 600 tigers. Find an expression that will model the population of leopards at any time (t)arrow_forwardSuppose you want to find an equation for a curve that passes through the point (1, 2x3 y 2) and whose slope at any point (x, y) is A. B. C. You'd solve the differential equation Solving this differential equation with the initial condition, you get: E. 2y2 = x4 +7 نيا v2 = 2x4+2 ² = x4 +3 Op. ²x4+4 = D. 1²=14+3 p2 dx -2x³ = with initial condition y(1) = 2.arrow_forwardA firm uses capital (K) and labour (E) to produce output according to the production function Q(K,E)=lnK+lnE for K > 0 and E > 0, where ln is the natural logarithm. When either K = 0 or E = 0 or both, output is zero. The firm's cost function is C(K,E) = 9K^2+E^2. The firm wants to choose K and E so as to maximize its output subject to spending exactly $1,800. Solve the firm's constrained maximization problem and its Lagrangian function. Derive the first order conditions and find the candidate solution.The unique stationary point of the firm's constrained profit maximization problem is given by K*=? E*=?arrow_forward
- The population of an island from 1970 to 2020 is modeled by the logistic function: P(t)= 150,000/1+5e^-.124t where t is the number of years that have passed since 1970. A.) find the exact population of the island in 1970 and 2020 B.) given that the logistic growth model will also be valid in the future, write down the largest possible population that the island will ever be able to accommodate C.) find the number of years it took for the population of the island to reach 100,000arrow_forward5. The accumulated average price in millions of dollars of a two-bedroom apartment in downtown New York City from 1990 to 2010 could be approximated by P(t) = = 0.63e0.25t, where t is time in years since 1990. a) Determine P(6) and interpret your answer in context of the problem. b) Determine P'(6) and interpret your answer in context of the problem c) Determine P"(6) and interpret your answer in context of the problem.arrow_forward
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
 Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning
Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON
Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON
Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
 Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning
Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning

Calculus: Early Transcendentals
Calculus
ISBN:9781285741550
Author:James Stewart
Publisher:Cengage Learning

Thomas' Calculus (14th Edition)
Calculus
ISBN:9780134438986
Author:Joel R. Hass, Christopher E. Heil, Maurice D. Weir
Publisher:PEARSON

Calculus: Early Transcendentals (3rd Edition)
Calculus
ISBN:9780134763644
Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric Schulz
Publisher:PEARSON

Calculus: Early Transcendentals
Calculus
ISBN:9781319050740
Author:Jon Rogawski, Colin Adams, Robert Franzosa
Publisher:W. H. Freeman


Calculus: Early Transcendental Functions
Calculus
ISBN:9781337552516
Author:Ron Larson, Bruce H. Edwards
Publisher:Cengage Learning