Question
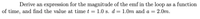
Transcribed Image Text:Derive an expression for the magnitude of the emf in the loop as a function
of time, and find the value at timet = 1.0 s. d= 1.0m and a = 2.0m.
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by stepSolved in 4 steps

Knowledge Booster
Similar questions
- An inductor of L = 6.95 H with negligible resistance is placed in series with a 8 = 15.7 V battery, a R = 3.00 resistor, and a switch. The switch is closed at time t = 0 seconds. L. Calculate the initial current at t= 0 seconds. ww R. 1(t = 0 s) = Calculate the current as time approaches infinity. Imax = Calculate the current at a time of 1.85 s. I(t = 1.85 s) = %3D %3D Determine how long it takes for the current to reach half of its maximum. S 0000arrow_forwardIn the figure, R = 11.0 Ω, C = 6.82 μF, and L = 54.0 mH, and the ideal battery has emf = 32.0 V. The switch is kept in position a for a long time and then thrown to position b. What are the (a) frequency and (b) current amplitude of the resulting oscillations? Image is attached, thanksarrow_forward006 In a model AC generator, a 485-turn rect- angular coil, 3.8 cm by 17 cm, rotates at 54 rev/min in a uniform magnetic field of 0.36 T.arrow_forward
- Initially, an inductor with no resistance carries a constant current. Then the current is brought to a new constant value twice as large. After this change, when the current is constant at its higher value, what has happened to the emf in the inductor?arrow_forwardAn RL circuit consists of a switch, an emf source E,, a 3.0 ka resistor, and a 1.0H inductor. If the potential across the resistor is 15.0 V 8.0 ms after the switch is closed, find the source emf, E..arrow_forwardIn the figure, R = 11.0 Ω, C = 6.82 μF, and L = 54.0 mH, and the ideal battery has emf = 32.0 V. The switch is kept in position a for a long time and then thrown to position b. What are the (a) frequency and (b) current amplitude of the resulting oscillations?arrow_forward
- ℇ = 100 V, R = 20.0 Ω, and L = 100.0 mH. The switch is closed att = 0. (a) What is the time constant of the circuit shown below? (b) Find the currentat t = 0.050 sec, (c) at what time the current is 40% of its maximum value?arrow_forwardTwo resistors of resistance R1and R2 are placed in series across a battery with voltage V, such that a current I=V/(R1+R2) flows around the circuit. If a coil with inductance L is then placed inparallel withR1, what is the new currentIa long time after the coil has been added? Give your final answer.arrow_forwardConsider the circuit shown in the figure below. Take E = 6.00 V, L = 7.92 mH, and R = 3.60 Ω. S + I R ww (a) What is the inductive time constant of the circuit? ms (b) Calculate the current in the circuit 250 us after the switch is closed. A (c) What is the value of the final steady-state current? A (d) How long does it take the current to reach 81.0% of its maximum value? msarrow_forward
- The current in the RL circuit shown below reaches one-fourth its maximum value in 2.75 ms after the switch s, is thrown. R (a) Determine the time constant of the circuit (in ms). ms (b) Determine the resistance of the circuit (in 0) if L = 200 mH.arrow_forwardConsider a 22.5 mH inductor that has a resistance of 4.1 Ω. Part (a) What is the time constant, τ, of the inductor, in seconds? Part (b) If the inductor is connected to a 12.0 V battery, what is the current, I, in amperes, after 10.5 ms?arrow_forward
arrow_back_ios
arrow_forward_ios