
MATLAB: An Introduction with Applications
6th Edition
ISBN: 9781119256830
Author: Amos Gilat
Publisher: John Wiley & Sons Inc
expand_more
expand_more
format_list_bulleted
Question

Transcribed Image Text:**Uniform Distribution Problem**
Suppose a random variable has a continuous uniform distribution between 0 and 10, such that its probability density function is: \( f(x) = 1/10 \).
**Questions:**
a. What is the cumulative density function for \( x \)?
b. What is the mean (expected value) of \( x \)?
c. What is the variance of \( x \)?
d. According to Chebychev's rule, what is the smallest probability a random \( x \) will be within 2 standard deviations of its mean?
- i.e. \( P(|x - \mu| < 2 \ast \sigma) \)
e. What is the exact probability that a random \( x \) will fall within 2 standard deviations of its mean for this uniform distribution?
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution
Trending nowThis is a popular solution!
Step by stepSolved in 3 steps with 2 images

Follow-up Questions
Read through expert solutions to related follow-up questions below.
Follow-up Question
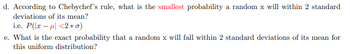
Transcribed Image Text:d. According to Chebychev’s rule, what is the smallest probability a random x will within 2 standard deviations of its mean?
i.e. \( P(|x - \mu| < 2 \ast \sigma) \)
e. What is the exact probability that a random x will fall within 2 standard deviations of its mean for this uniform distribution?
Solution
by Bartleby Expert
Follow-up Questions
Read through expert solutions to related follow-up questions below.
Follow-up Question
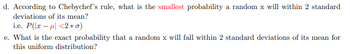
Transcribed Image Text:d. According to Chebychev’s rule, what is the smallest probability a random x will within 2 standard deviations of its mean?
i.e. \( P(|x - \mu| < 2 \ast \sigma) \)
e. What is the exact probability that a random x will fall within 2 standard deviations of its mean for this uniform distribution?
Solution
by Bartleby Expert
Knowledge Booster
Similar questions
- Given the probability density function f(x) = over the interval [2, 7], find the expected value, the mean, 5 the variance and the standard deviation. Expected value: Mean: Variance: Standard Deviation: > Next Questionarrow_forwardAssume that the amounts of weight that male college students gain during their freshman year are normally distributed with a mean of μ = 1.1 kg and a standard deviation of o=4.9 kg. Complete parts (a) through (c) below. a. If 1 male college student is randomly selected, find the probability that he gains between 0 kg and 3 kg during freshman year. The probability is (Round to four decimal places as needed.) -Carrow_forwardA random variable X has density 3x2 for 0 < x < 1. Find its variance.(use a decimal number, rounded to the nearest 1,000th. For example, 0.123)arrow_forward
- Consider a random variable X that follows a normal distribution with a mean (μ) of 50 and a standard deviation (σ) of 5. Calculate the probability that X is less than 40.arrow_forwardSuppose X is a random variable defined as final cost of project estimated cost of project which has a PDF as follows: [0 xa a. Determine the value of a. b. What is the probability that the final cost of a project will exceed its estimated cost by 25%? c. Determine the mean value and standard deviation of X.arrow_forwardChoose ALL THE CORRECT STATEMENTS in the following ones about probability distribution. i. Probability mass function indicates the probability distribution of a continuous random variable. ii. Probability mass function indicates the probability distribution of a discrete random variable. iii. The probability mass function of a discrete random variable X at a is denoted by P(X < a). iv. The probability function of a continuous random variable Y can be described by using P(Y= a).arrow_forward
- Q1. Suppose X is a continuous random variable. Find an example of a probability density function for X giving expected value E(X) = 1 and variance V (X) = 3 if X has . . . (a.) a uniform distribution. (b.) an exponential distribution. (c.) a normal distribution. In each case, if there is no such probability density function, explain why this is so.arrow_forwardGiven the random variable X and its probability density function below find the standard deviation of X. Pr(X) 0.1 0.9 3.arrow_forward
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
 MATLAB: An Introduction with ApplicationsStatisticsISBN:9781119256830Author:Amos GilatPublisher:John Wiley & Sons Inc
MATLAB: An Introduction with ApplicationsStatisticsISBN:9781119256830Author:Amos GilatPublisher:John Wiley & Sons Inc Probability and Statistics for Engineering and th...StatisticsISBN:9781305251809Author:Jay L. DevorePublisher:Cengage Learning
Probability and Statistics for Engineering and th...StatisticsISBN:9781305251809Author:Jay L. DevorePublisher:Cengage Learning Statistics for The Behavioral Sciences (MindTap C...StatisticsISBN:9781305504912Author:Frederick J Gravetter, Larry B. WallnauPublisher:Cengage Learning
Statistics for The Behavioral Sciences (MindTap C...StatisticsISBN:9781305504912Author:Frederick J Gravetter, Larry B. WallnauPublisher:Cengage Learning Elementary Statistics: Picturing the World (7th E...StatisticsISBN:9780134683416Author:Ron Larson, Betsy FarberPublisher:PEARSON
Elementary Statistics: Picturing the World (7th E...StatisticsISBN:9780134683416Author:Ron Larson, Betsy FarberPublisher:PEARSON The Basic Practice of StatisticsStatisticsISBN:9781319042578Author:David S. Moore, William I. Notz, Michael A. FlignerPublisher:W. H. Freeman
The Basic Practice of StatisticsStatisticsISBN:9781319042578Author:David S. Moore, William I. Notz, Michael A. FlignerPublisher:W. H. Freeman Introduction to the Practice of StatisticsStatisticsISBN:9781319013387Author:David S. Moore, George P. McCabe, Bruce A. CraigPublisher:W. H. Freeman
Introduction to the Practice of StatisticsStatisticsISBN:9781319013387Author:David S. Moore, George P. McCabe, Bruce A. CraigPublisher:W. H. Freeman

MATLAB: An Introduction with Applications
Statistics
ISBN:9781119256830
Author:Amos Gilat
Publisher:John Wiley & Sons Inc

Probability and Statistics for Engineering and th...
Statistics
ISBN:9781305251809
Author:Jay L. Devore
Publisher:Cengage Learning

Statistics for The Behavioral Sciences (MindTap C...
Statistics
ISBN:9781305504912
Author:Frederick J Gravetter, Larry B. Wallnau
Publisher:Cengage Learning

Elementary Statistics: Picturing the World (7th E...
Statistics
ISBN:9780134683416
Author:Ron Larson, Betsy Farber
Publisher:PEARSON

The Basic Practice of Statistics
Statistics
ISBN:9781319042578
Author:David S. Moore, William I. Notz, Michael A. Fligner
Publisher:W. H. Freeman

Introduction to the Practice of Statistics
Statistics
ISBN:9781319013387
Author:David S. Moore, George P. McCabe, Bruce A. Craig
Publisher:W. H. Freeman