d) To explain the quantization observed in the emission spectra of hydrogen, Bohr introduced 'quantization' to the classical model by suggesting the angular momentum of the electron was quantized. This means the angular momentum can only have discrete values. Bohr quantized the angular momentum in multiples of Planck's constant; (4) In this equation m is the mass of the electron m = 9.11 x 10-31 kg and ħ is the modified Plancks's constant h/2r; where h = h/2π = 1.05 x 10-34 J-s = 6.58 x 10-16 eV-s. mvr = nħ n = 1,2,3,.... Re-arrange the angular momentum expression given in Eqn. (4) for v and substitute into Eqn. (1) to prove the radius of the electron's orbit is given by r = n²ħ²/(e²km) where k= (1/4πeo) = 8.99 x 10⁹ N-m²/C². This is the general equation for the allowed radii of the electron's 'orbit' in Bohr's model of hydrogen. Show all work.
d) To explain the quantization observed in the emission spectra of hydrogen, Bohr introduced 'quantization' to the classical model by suggesting the angular momentum of the electron was quantized. This means the angular momentum can only have discrete values. Bohr quantized the angular momentum in multiples of Planck's constant; (4) In this equation m is the mass of the electron m = 9.11 x 10-31 kg and ħ is the modified Plancks's constant h/2r; where h = h/2π = 1.05 x 10-34 J-s = 6.58 x 10-16 eV-s. mvr = nħ n = 1,2,3,.... Re-arrange the angular momentum expression given in Eqn. (4) for v and substitute into Eqn. (1) to prove the radius of the electron's orbit is given by r = n²ħ²/(e²km) where k= (1/4πeo) = 8.99 x 10⁹ N-m²/C². This is the general equation for the allowed radii of the electron's 'orbit' in Bohr's model of hydrogen. Show all work.
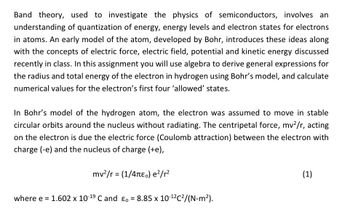
Transcribed Image Text:Band theory, used to investigate the physics of semiconductors, involves an
understanding of quantization of energy, energy levels and electron states for electrons
in atoms. An early model of the atom, developed by Bohr, introduces these ideas along
with the concepts of electric force, electric field, potential and kinetic energy discussed
recently in class. In this assignment you will use algebra to derive general expressions for
the radius and total energy of the electron in hydrogen using Bohr's model, and calculate
numerical values for the electron's first four 'allowed' states.
In Bohr's model of the hydrogen atom, the electron was assumed to move in stable
circular orbits around the nucleus without radiating. The centripetal force, mv²/r, acting
on the electron is due the electric force (Coulomb attraction) between the electron with
charge (-e) and the nucleus of charge (+e),
mv²/r = (1/4π) e²/r²
where e = 1.602 x 10-19 C and Eo = 8.85 x 10-¹²C²/(N-m²).
(1)
Transcribed Image Text:c) The total mechanical energy of the electron is the sum of its kinetic energy and
potential energy. Derive an expression for the total mechanical energy of the electron
in the hydrogen atom;
(3)
Your result should be negative. The negative result means energy must be added to
excite the electron to higher energy levels 'farther away' from the nucleus. In this
coordinate system the total energy is zero at an infinite distance from the nucleus.
E = KE + U
d) To explain the quantization observed in the emission spectra of hydrogen, Bohr
introduced 'quantization' to the classical model by suggesting the angular momentum
of the electron was quantized. This means the angular momentum can only have
discrete values. Bohr quantized the angular momentum in multiples of Planck's
constant;
(4)
In this equation m is the mass of the electron m = 9.11 x 10-31 kg and ħh is the modified
Plancks's constant h/2; where ħ = h/2π = 1.05 x 10-34 J-s = 6.58 x 10-16 eV-s.
n=1-+
(-13.6 eV)
mvr = nh
Re-arrange the angular momentum expression given in Eqn. (4) for v and substitute
into Eqn. (1) to prove the radius of the electron's orbit is given by r = n²ħ²/(e²km)
where k= (1/4πeo) = 8.99 x 10° N-m²/C². This is the general equation for the allowed
radii of the electron's 'orbit' in Bohr's model of hydrogen. Show all work.
n = 1,2,3,....
-n=2
(-3.39 eV)
n=3
(-1.51 eV)
e) Next substitute r = n²ħ²/(e²km) into the expression for the total mechanical energy of
the electron, Eqn. (3), to obtain E = (-1/n²) (me4/8e02 h²). This result gives the general
equation for the electron's allowed energy values in Bohr's model of the hydrogen
atom. Show all work.
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, chemistry and related others by exploring similar questions and additional content below.
ChemistryChemistryISBN:9781259911156Author:Raymond Chang Dr., Jason Overby ProfessorPublisher:McGraw-Hill Education
Principles of Instrumental AnalysisChemistryISBN:9781305577213Author:Douglas A. Skoog, F. James Holler, Stanley R. CrouchPublisher:Cengage Learning
Organic ChemistryChemistryISBN:9780078021558Author:Janice Gorzynski Smith Dr.Publisher:McGraw-Hill Education
Chemistry: Principles and ReactionsChemistryISBN:9781305079373Author:William L. Masterton, Cecile N. HurleyPublisher:Cengage Learning
Elementary Principles of Chemical Processes, Bind...ChemistryISBN:9781118431221Author:Richard M. Felder, Ronald W. Rousseau, Lisa G. BullardPublisher:WILEY