
Advanced Engineering Mathematics
10th Edition
ISBN: 9780470458365
Author: Erwin Kreyszig
Publisher: Wiley, John & Sons, Incorporated
expand_more
expand_more
format_list_bulleted
Question
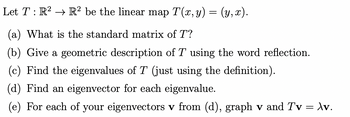
Transcribed Image Text:Let T : R² → R² be the linear map T(x, y)
=
(y,x).
(a) What is the standard matrix of T?
(b) Give a geometric description of T using the word reflection.
(c) Find the eigenvalues of T (just using the definition).
(d) Find an eigenvector for each eigenvalue.
(e) For each of your eigenvectors v from (d), graph v and Tv = \v.
Expert Solution
arrow_forward
Step 1
Note : " As per our guidelines we will solve three subparts . If you want any specific subpart to be solved please specify that subpart or post only that subpart . "
(.) Linear map given by ,
(.) Standard basis of is .
(.) Let be a linear transformation , then is a eigen value of if and only if is singular . Here is an identity transformation and is a vector space over the field .
(.) Singular linear transformation : A linear transformation is said to be singular if there exists a vector such that .
Step by stepSolved in 2 steps

Follow-up Questions
Read through expert solutions to related follow-up questions below.
Follow-up Question
Could you give the specific details about d) and e)?
Solution
by Bartleby Expert
Follow-up Questions
Read through expert solutions to related follow-up questions below.
Follow-up Question
Could you give the specific details about d) and e)?
Solution
by Bartleby Expert
Knowledge Booster
Similar questions
- 4. Let W be the xy-plane in R³. (a) Find an orthonormal basis ū₁, 2 for W. (b) Construct the matrix Q = [ ₁ ü₂ ] and calculate the matrix QQT. (c) Consider the linear transformation T: R³ → R³ that projects each vector orthogonally onto the xy-plane. Note that T() = QQT. Use the matrix to calculate T(₁), T(2), and T(ē3). (d) Explain how your answers make sense geometrically.arrow_forward(1) For the following linear transformations T find its matrix representation with respect to the standard basis.arrow_forwardLet T be the linear transformation associated with the matrix: 0 1 -1 0 Find T(V) if V is the vector: 2 2 Is the transformation a rotation or a reflection? Justify why.arrow_forward
- Let T : P2 → M2x2 be the linear transformation defined by T(ax? + bx + c) |0 a (a) Find the matrix for T with respect to the standard bases of P2 and M2x2- (b) Find the matrix for T with respect t B basis for M2x2. {1, x+1, x²+2x+1} and the standardarrow_forwardFind the matrix of the linear transformation T from R? R?, where T([1, 0]) = [1, -2], and T([2, 1]) = [2, 3] (Note: matrix A = [T([1,0]), T([0, 1])] ). 1 (a) 1 (b) 1. (c) -2 1 -2 3 -2 1 (d) 0. 3arrow_forwardLet T : R2 → R² be the linear transformation which rotates vectors counter-clockwise about the origin by 7T/3 radians (60 degrees). (i) Find the standard matrix of the linear transformation T. (ii) Use your matrix to rotate the vector v = counterclockwise about the origin by 4 T/3 radians.arrow_forward
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
 Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated
Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education
Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY
Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,


Advanced Engineering Mathematics
Advanced Math
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:9780073397924
Author:Steven C. Chapra Dr., Raymond P. Canale
Publisher:McGraw-Hill Education

Introductory Mathematics for Engineering Applicat...
Advanced Math
ISBN:9781118141809
Author:Nathan Klingbeil
Publisher:WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,

