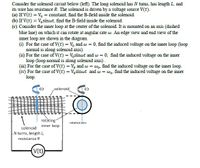
Consider the solenoid circuit below (left). The long solenoid has N tums, has length L, and its wire has resistance R. The solenoid is driven by a voltage source V(t). (a) If V(t) = V, = constant, find the B-field inside the solenoid. (b) If V(t) = V,sinwt, find the B-field inside the solenoid. (c) Consider the inner loop at the center of the solenoid. It is mounted on an axis (dashed blue line) on which it can rotate at angular rate w. An edge view and end view of the inner loop are shown in the diagram. (i) For the case of V(t) = V, and w = 0, find the induced voltage on the inner loop (lop normal is along solenoid axis). (i) For the case of V(t) = V,sinwt and w = 0, find the induced voltage on the inner loop (loop normal is along solenoid axis). (iii) For the case of V(t) = V, and w = awo. find the induced voltage on the inner loop. (iv) For the case of V(t) = Vosinwt and w = wo, find the induced voltage on the inner loop.

Let's consider a perfect solenoid: radius R and infinite length.    A current I flows through it. Assuming that B is zero outside the solenoid    of the solenoid (true if L tends to infinity...!) use Ampère's law to calculate    to calculate B inside the solenoid.

A solenoid that is 111 cm long has a cross-sectional area of 19.8 cm². There are 1170 turns of wire carrying a current of 8.89 A. (a) Calculate the energy density of the magnetic field inside the solenoid. (b) Find the total energy in joules stored in the magnetic field there (neglect end effects).

An aluminum ring of radius 5.00 cm and resistance 3.00 × 10–4 O is placed on top of a long air-core solenoid with 1 000 turns per meter and radius 3.00 cm, as shown in the sketch below. Over the area of the end of the solenoid, assume that the axial component of the field produced by the solenoid is half as strong as at the center of the solenoid. Assume the solenoid produces negligible field outside its cross-sectional area. The current in the solenoid is increasing at a rate of 270 A/s. (a) What is the induced current in the ring? At the center of the ring, what are (b) the magnitude and (c) the direction of the magnetic field produced by the induced current in the ring?

A toroidal solenoid has a magnitude of induced emf of 10.3 [mV] when the rate of change of the current is 0.0250 [A/s]. How many turns does it have if the average flux through each turn is 0.00145 [Wb] when the current is 1.12 [A]?

For a solenoid in vacuum of length l = 10 cm with 104 turns per meter calculate the current required to create the field B = 70 µT. Provide your answer in Sl units.

b' ε A solenoid has a length = 11.34 cm, a radius a = 1.85 cm, and 1627 turns. The solenoid has a net resistance R sol = 144.9 2. A circular loop with radius b = 3.77 cm is placed around the solenoid, such that it lies in a plane whose normal is aligned with the solenoid axis, and the center of the outer loop lies on the solenoid axis. The outer loop has a resistance Ro = 1651.6 2. At a time t = 0 s, the solenoid is connected to a battery that supplies a potential & = 34.95 V. At a time t = 2.58 μs, what current flows through the outer loop? Ax 1.4e-5 14x10-5

. The following picture shows a LONG conductor carrying current 1. Nearby there is a conducting rectangular loop with sides a = 8 cm and b = 4 cm. The loop also carries a resistance R = 10 ohms. The curent is constant and has a value of I = 6.0 Amperes. The loop is moving away to the right with a constant velocity, V = 2 m/s. Answer the following questions at the instant of time t" when the left edge of the loop is at position "x" as shown below Use the coordinate system , x to the right, y into the board, z upward a) Write an expression for the magnetic field as a function of the distance "x" (from the LONG conductor to the loop. ) USE “+" for CCW circulation and “.“ for CW circulation. b) Write the magnetic field in "i-j-k" format at point "x" to the right of the current carrying wire in the "i-zZ" plane R a c) Write the infinitesimal area vector for the loop in "i-j-k" format d) Write the explicit integral for the magnetic flux through the area of the loop using the answer for B and…

A long solenoid radius 2.16 m is wound with 20.5 turns/m and carries a current that changes at the rate of 29.7A / s as in the figure below. What is the magnitude of the emf induced in the square conducting loop surrounding the centre of the solenoid?The induced emf is e= ? mv . (Note: 1 mV=10^ -3 V)

For a technical application, an electrical engineer needs to create a solenoid using copper wire with diameter d = 0.500 mm. The resistance of the solenoid needs to be 5.10 2 and the magnetic field of the solenoid with a current of 3.10 A should be 5.90 x 10-2 T. Assume the resistance does not change significantly with temperature, and the radius of the solenoid is 1.00 cm. (Use p = 1.70 x 1080 m for the resistivity of copper.) (a) How many turns of wire does the electrical engineer need? turns (b) Determine the required length (in cm) of the solenoid. cm