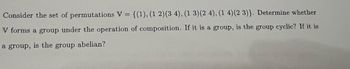
Consider the set of permutations V = {(1), (1 2) (3 4), (1 3) (2 4), (1 4) (2 3)}. Determine whether V forms a group under the operation of composition. If it is a group, is the group cyclic? If it is a group, is the group abelian?

Let G be a group, and assume that a and b are two elements of order 2 in G. If ab = ba, then what can you say about (a, b)?

Write out the Cayley table for the group {1, -1, i, -i} under multiplication where i = sqrt(-1). (In general, we apply the row element on the left of the column element.)

19. 20. 21. 22. 23. 24. 25. Consider the groups U(8) and Z4 c) d) e) f) Let H₁, H₂ and H, be abelian groups. Prove or disprove: H, xH₂x H, is an abelian group. List the elements of U(8) x Z4 Determine the identity element of this group. Determine all the elements which are of order two in this group. Determine all the elements of order 4 in this group.? Determine the subgroup generated by the element (7,1) Let K = {xe G|xg=gx Vge G} Show that K is a normal subgroup of G. Define f: R² R² by f(x,y) = (x + 2y, 0) h) i) a) b) Show that f is a homomorphism from into itself Find Ker(f) Let 0: - be defined by 0 la b C by σ(n) =i" a) b) Prove that is a homomorphism Determine Ker (0) Let G= - Verify that o is a homomorphism Find Ker(0) (a,b,c,de R ) Use the Fundamental homomorphism theorem to prove that 45

is it a binary option? is it associative? identity element= inverse elements=

Let G = Zp × Zp. Is this group cyclic? As you know any cyclic group canbe generated by one element. If G is not cyclic how many elements you need to generate this group. What is the smallest size of a generating set?