Question
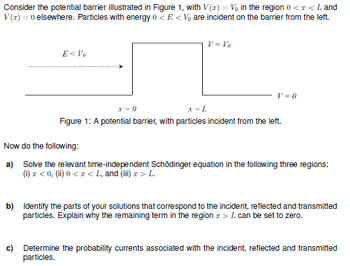
Transcribed Image Text:Consider the potential barrier illustrated in Figure 1, with V(x) = V₁ in the region 0 < x < L and
V(T) = 0 elsewhere. Particles with energy 0 < E<V₁ are incident on the barrier from the left.
E< Vo
V = Vo
V = 0
x = 0
x=L
Figure 1: A potential barrier, with particles incident from the left.
Now do the following:
a) Solve the relevant time-independent Schödinger equation in the following three regions:
(i) r < 0, (ii) 0 < r < L, and (iii) 2 > L.
b) Identify the parts of your solutions that correspond to the incident, reflected and transmitted
particles. Explain why the remaining term in the region > L can be set to zero.
c) Determine the probability currents associated with the incident, reflected and transmitted
particles.
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution
Trending nowThis is a popular solution!
Step by stepSolved in 5 steps with 5 images

Knowledge Booster
Similar questions
- Calculate the expectation value of the momentum (p) and the square of the momentum (p2) for the particle in a box of length L and infinite potential outside the box.arrow_forward4.3 A particle with mass m and energy E is moving in one dimension from right to left. It is incident on the step potential V(x) = 0 for x 0, as shown on the diagram. The energy of the particle is E > Vo. = V(x) V = Vo V=0 x = 0 (a) Solve the Schrödinger equation to derive 4(x) for x 0. Express the solution in terms of a single unknown constant. (b) Calculate the value of the reflection coefficient R for the parti- cle.arrow_forwardFor the potential-energy functions shown below, spanning width L, can a particle on the left side of the potential well (x 0.55L)? If not, why not? b. c. 0 a. 0o 00 E E E-arrow_forward
- Consider a finite potential step with V = V0 in the region x < 0, and V = 0 in the region x > 0 (image). For particles with energy E > V0, and coming into the system from the left, what would be the wavefunction used to describe the “transmitted” particles and the wavefunction used to describe the “reflected” particles?arrow_forwardProblem 15. In the case of a particle tunneling through the barrier, we made equal both functions and derivatives at the boundary between region I and II, and between II and III, see eq. (9a – 9d). But in the case of the particle in the box, we made both functions equal at x = 0 and at x = L. Explain why we did not consider derivatives in this case.arrow_forward
arrow_back_ios
arrow_forward_ios