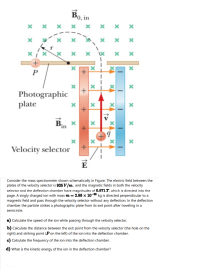
Consider the mass spectrometer shown schematically in Figure. The electric field between the plates of the velocity selector is 925 V/m, and the magnetic fields in both the velocity selector and the deflection chamber have magnitudes of 0.871 T, which is directed into the page. A singly charged ion with mass m=2.98 x 10-26 kg is directed perpendicular to a magnetic field and pass through the velocity selector without any deflection. In the deflection chamber the particle strikes a photographic plate from its exit point after traveling in a semicircle. Calculate the speed of the ion while passing through the velocity selector. b) Calculate the distance between the exit point from the velocity selector (the hole on the right) and striking point (Pon the left) of the ion into the deflection chamber. c) Calculate the frequency of the ion into the deflection chamber.

The electric field between the plates of the velocity selector is 970 V/m, and the magnetic fields in both the velocity selector and the deflection chamber have magnitudes of 0.945 T. Calculate the radius r of the path for a singly charged ion with mass m = 2.20 ✕ 10−26 kg.

An ion that is doubly ionized passes through the velocity selector and into the deflection chamber of a mass spectrometer, as shown below. In the velocity selector the electric field has a magnitude of 7761 V/m, and the magnitude of the magnetic field in both the velocity selector and the deflection chamber is 0.0903 T. If in the deflection chamber the ion is detected at a distance of 11.9 cm from its entry point, determine the following. B + + (a) mass-to-charge ratio of the ion kg/C (b) mass of the ion kg (c) identity of the ion, assuming it's an element (Use only the masses of elements in their most common form as listed on the periodic table of elements.)

Consider the mass spectrometer shown schematically in the figure below. The magnitude of the electric field between the plates of the velocity selector is 2,463.22 V/m, and the magnetic field in both the velocity selector and the deflection chamber has a magnitude of 0.03 T. Calculate the radius of the path in meter for a singly charged ion having a mass m=2.10x10-26 kg. (Do not include the units and keep two decimal places.) The charge of an electron is 1.6x10-19 c. Во, in х х х х Detector array in Velocity selector -- +