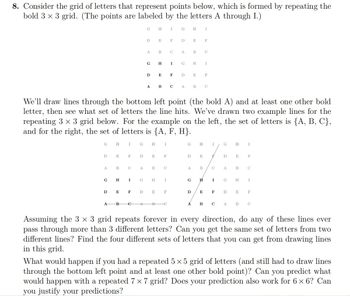
Consider the grid of letters that represent points below, which is formed by repeating the bold 3 x 3 grid. (The points are labeled by the letters A through I.) G D E A A G DE A B H I D 6 A B GHI HIG H I E GHIGH_ DEFDE FDE F B CA B C в с F We'll draw lines through the bottom left point (the bold A) and at least one other bold letter, then see what set of letters the line hits. We've drawn two example lines for the repeating 3 x 3 grid below. For the example on the left, the set of letters is {A, B, C}, and for the right, the set of letters is {A, F, H}. G H I/G H I C F A B € F G H I DE F A B C GHI DE F A DE A в с G D X DE F B C A B C HIGHI E F DE F A B CAB C

I need to integrate e2xsinxdx but I am unsure of what method to use?

Where did 11/16,723,070 came from?

Hi, I need some help foor this question. Please do not copy with chatgpt. Please write clear I need some explain. The chatgpt is not correct. Thanks!  Rick and Morty accidentally created a highly contagious virus that is capable of killing the aliens on planet Zoinks in under a day. So far, only 8% of the population of Zoinks has been infected. Rick and Morty whipped up a test for the virus, but the test is not perfect: 90% of the time it correctly identifies a healthy alien as not having the virus, but 25% of the time it incorrectly identifies an infected alien as virus-free. One of the aliens on the planet is worried that they may have contracted the virus, so they takes 20 independent tests. Of these 20 tests, 6 indicate that they have the disease and the remaining 14 indicate that they do not. With this information, what is the probability that the alien actually has the virus?  Please First use Bayes' formula, then for the conditional probability, and then need to use binomial…

In a recent article published by Scientific American found that only 82% of Millennials are certain the world is round. That seems low to you, you believe that more than 82% of Millennials believe the world is round. You randomly sample 75 Millennials and find that 68 of them believe the earth is round. The others didn't necessarily believe it is flat they might just be unsure of the earth's shape. Use your choice of Excel or Ti Calculator to complete a one proportion Z-test at 0.01 significance level. If using Excel, copy and Paste the appropriate Excel calculator into the sheet labeled Proportions.  Or if using Ti Calculator, type an explanation of the Ti Calculator features you used and include the Z test statistic, and the p-value.

Please do not rely too much on chatgpt, because its answer may be wrong. Please consider it carefully and give your own answer. You can borrow ideas from gpt, but please do not believe its answer.Very very grateful!Please do not rely too much on chatgpt, because its answer may be wrong. Please consider it carefully and give your own answer. You can borrow ideas from gpt, but please do not believe its answer.   Very very grateful!

Please do not rely too much on chatgpt, because its answer may be wrong. Please consider it carefully and give your own answer. You can borrow ideas from gpt, but please do not believe its answer.Very very grateful!Please do not rely too much on chatgpt, because its answer may be wrong. Please consider it carefully and give your own answer. You can borrow ideas from gpt, but please do not believe its answer.Very very grateful!

Please do not rely too much on chatgpt, because its answer may be wrong. Please consider it carefully and give your own answer. You can borrow ideas from gpt, but please do not believe its answer.Very very grateful!Please do not rely too much on chatgpt, because its answer may be wrong. Please consider it carefully and give your own answer. You can borrow ideas from gpt, but please do not believe its answer. and Very very grateful!

What about third one?

could you provide an example please?