Consider the function f: R → R defined by f(x) = cos(x), where n is an even integer. It can be shown that any positive power of cos x can be expressed as a sum of multiples of terms of the form cos(kx), where the k are non-negative integers. Note that the term where k = 0 is the constant term. It can also be shown that any odd positive power of sin a can be expressed as a sum of multiples of terms of the form sin(kx), where the k are non-negative integers. (a) What is the constant term in the expansion of f(x) described above? & A Syntax advice: Remember to use correct Maple syntax. For example, • the factorial n! may be written as n! or factorial (n) the binomial coefficient (R) • the term a* is written a^x may be written as binomial (n,k) (b) Now, consider the function g: R → R defined by g(x) = sin 975 (x)f(x). Briefly explain in words why g(x) may be written as a sum of multiples of terms of the form sin(kx), where k are non-negative integers.
Consider the function f: R → R defined by f(x) = cos(x), where n is an even integer. It can be shown that any positive power of cos x can be expressed as a sum of multiples of terms of the form cos(kx), where the k are non-negative integers. Note that the term where k = 0 is the constant term. It can also be shown that any odd positive power of sin a can be expressed as a sum of multiples of terms of the form sin(kx), where the k are non-negative integers. (a) What is the constant term in the expansion of f(x) described above? & A Syntax advice: Remember to use correct Maple syntax. For example, • the factorial n! may be written as n! or factorial (n) the binomial coefficient (R) • the term a* is written a^x may be written as binomial (n,k) (b) Now, consider the function g: R → R defined by g(x) = sin 975 (x)f(x). Briefly explain in words why g(x) may be written as a sum of multiples of terms of the form sin(kx), where k are non-negative integers.
Related questions
Question
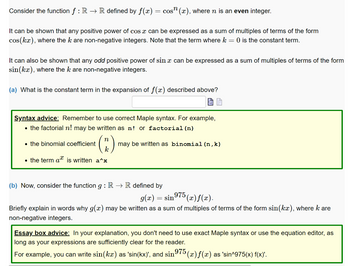
Transcribed Image Text:Consider the function f : R → R defined by f(x) cos(x), where n is an even integer.
It can be shown that any positive power of cos x can be expressed as a sum of multiples of terms of the form
cos(kx), where the k are non-negative integers. Note that the term where k = 0 is the constant term.
It can also be shown that any odd positive power of sin x can be expressed as a sum of multiples of terms of the form
sin(kx), where the k are non-negative integers.
(a) What is the constant term in the expansion of f(x) described above?
Syntax advice: Remember to use correct Maple syntax. For example,
• the factorial n! may be written as n! or factorial (n)
(2)
• the term a is written a^x
• the binomial coefficient
may be written as binomial (n,k)
(b) Now, consider the function g: R → R defined by
g(x) = sin
975 (x) f(x).
Briefly explain in words why g(x) may be written as a sum of multiples of terms of the form sin(kx), where k are
non-negative integers.
Essay box advice: In your explanation, you don't need to use exact Maple syntax or use the equation editor, as
long as your expressions are sufficiently clear for the reader.
975
For example, you can write sin(kx) as 'sin(kx)', and sin
'(x)f(x) as 'sin^975(x) f(x)'.
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by step
Solved in 6 steps with 5 images
