
Advanced Engineering Mathematics
10th Edition
ISBN: 9780470458365
Author: Erwin Kreyszig
Publisher: Wiley, John & Sons, Incorporated
expand_more
expand_more
format_list_bulleted
Question
thumb_up100%
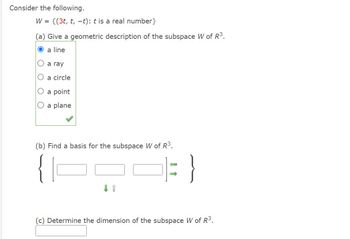
Transcribed Image Text:Consider the following.
W = {(3t, t, -t): t is a real number}
(a) Give a geometric description of the subspace W of R³.
O a line
a ray
O a circle
a point
O a plane
(b) Find a basis for the subspace W of R³.
-
}
(c) Determine the dimension of the subspace W of R³.
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution
Trending nowThis is a popular solution!
Step by stepSolved in 3 steps with 3 images

Knowledge Booster
Similar questions
- 2arrow_forward8arrow_forwardThe set = 211 {(2a, b, a - b): a, b = R} 0,00) quo & jon ai & Jon ai (o.A) toda wodewoled ol is a subspace of R³. (a) Show that B = {(2, 1, 0), (2, 2, -1)} is a basis for S. (b) Is S a point, a line, a plane or R³ itself? (c) Use Gram-Schmidt orthogonalisation to find an orthogonal basis for S that includes the vector (2, 1,0). ai deda odmun oduo ovidiecq to ocharrow_forward
- Let Vi and V2 be subspaces of R" (over R) Their intersection V=V,nV, is the set of all vectors that lie both in Vị and in V2. Show that V is a subspace of R".arrow_forwardLet W be the set of all vectors in R² With second component equal to twice the first, that all vectors of the form (9,20) (a) Use the Test for a Subspace to show that W is a subspace of R². (6) Using the x-y plane to represent R² a sketch of W. makearrow_forwardA,B,C pleasearrow_forward
- Subspace W of R* is spanned by the vectors v1 = [1 – 2 3 – 1] and v2 = [1 1 – 2 3]1. - Then, what can be said about the subspace W? a) dim W = 1 b) dim W 2 c) dim W = 3 d) dim W = 4arrow_forwardHow to find the subspace of R4 ? I have attached the image with this question Its kinda very complicated to undertand it properly.arrow_forward
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
 Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated
Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education
Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY
Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,


Advanced Engineering Mathematics
Advanced Math
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:9780073397924
Author:Steven C. Chapra Dr., Raymond P. Canale
Publisher:McGraw-Hill Education

Introductory Mathematics for Engineering Applicat...
Advanced Math
ISBN:9781118141809
Author:Nathan Klingbeil
Publisher:WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,

