Introductory Circuit Analysis (13th Edition)
13th Edition
ISBN: 9780133923605
Author: Robert L. Boylestad
Publisher: PEARSON
expand_more
expand_more
format_list_bulleted
Related questions
Question
thumb_up100%
Consider the continuous-time periodic signal x(t) = Summation (k=−∞ to ∞) p(t + kT), where p(t) is defined within one period as:
p(t) = e^(−t), for − 1 ≤ t < 1
with the fundamental period T = 2.
a) Calculate the Fourier series coefficients ak for x(t).
b) Use FS properties to find Fourier series coefficients for x(t − 2), x(−t), x(2t).
c) Verify whether ak you obtain for x(t) satisfy conjugate symmetry
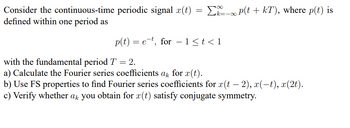
Transcribed Image Text:Consider the continuous-time periodic signal x(t) = ∞ P(t + kT), where p(t) is
defined within one period as
p(t) = e, for 1≤t<1
with the fundamental period T = 2.
a) Calculate the Fourier series coefficients ak for x(t).
-
b) Use FS properties to find Fourier series coefficients for x(t − 2), x(−t), x(2t).
c) Verify whether ak you obtain for x(t) satisfy conjugate symmetry.
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by stepSolved in 2 steps
Knowledge Booster
Similar questions
- -T ↑ f(t) a) List all symmetries applicable to the periodic function shown above. b) Find the Fourier series for f(t). c) Using the formula for the rms value of a periodic function, show that 100 7-²2 In 1,3,5... 1 2T n² [Hint: first find the rms value directly in the time domain (easy), then make use of the frequency domain formula.]arrow_forwardPart b onlyarrow_forward3. Let D, be the Fourier coefficients of periodic signal f (t). (a) When H, is the Fourier coefficients of h(t) =f(t)+1, explain the relation between H, and D.. (b) When P is the Fourier coefficients of p(t)=f(t-1), explain the relation between P and Darrow_forward
- I need help figuring out how to do these, step by step. Thank you.arrow_forwarda) Explain with the aid of sketches the meaning of the following terms, as related to periodic waveforms. i) Even symmetry i) Odd symmetry ii) Half wave symmetry b) Determine the Fourier series of the following function: - f(t) = 3 f(t) = 5 0arrow_forwardFind the Fourier series for the periodic extension of the signal x(t): = -1 -.5arrow_forwardFor a periodic waveform h(t), if its complex Fourier coefficients are T T 2n²n² 4nn T when n is even and not zero 4nn please derive the first five non-zero terms of the real Fourier series for h(t). C) T -j when n is oddarrow_forwardIn the following, write out the Fourier series for the following. You should do the integrals yourself (show the steps), rather than just asking a program for the result. A. A triangular wave: -p < x < 0, f(x) = 0 0 < x < p, f(x) = x B. A half-wave rectifier -p < x < 0, f(x) = 0, 0 < x < p, f(x) = sin xarrow_forwardPut (TRUE) or (FALSE) and say why : - Fourier series is only applicable for periodic signals.arrow_forwardarrow_back_iosSEE MORE QUESTIONSarrow_forward_iosRecommended textbooks for you
- Introductory Circuit Analysis (13th Edition)Electrical EngineeringISBN:9780133923605Author:Robert L. BoylestadPublisher:PEARSON
Delmar's Standard Textbook Of ElectricityElectrical EngineeringISBN:9781337900348Author:Stephen L. HermanPublisher:Cengage Learning
Programmable Logic ControllersElectrical EngineeringISBN:9780073373843Author:Frank D. PetruzellaPublisher:McGraw-Hill Education 
Fundamentals of Electric CircuitsElectrical EngineeringISBN:9780078028229Author:Charles K Alexander, Matthew SadikuPublisher:McGraw-Hill Education
Electric Circuits. (11th Edition)Electrical EngineeringISBN:9780134746968Author:James W. Nilsson, Susan RiedelPublisher:PEARSON
Engineering ElectromagneticsElectrical EngineeringISBN:9780078028151Author:Hayt, William H. (william Hart), Jr, BUCK, John A.Publisher:Mcgraw-hill Education,
Introductory Circuit Analysis (13th Edition)Electrical EngineeringISBN:9780133923605Author:Robert L. BoylestadPublisher:PEARSONDelmar's Standard Textbook Of ElectricityElectrical EngineeringISBN:9781337900348Author:Stephen L. HermanPublisher:Cengage LearningProgrammable Logic ControllersElectrical EngineeringISBN:9780073373843Author:Frank D. PetruzellaPublisher:McGraw-Hill EducationFundamentals of Electric CircuitsElectrical EngineeringISBN:9780078028229Author:Charles K Alexander, Matthew SadikuPublisher:McGraw-Hill EducationElectric Circuits. (11th Edition)Electrical EngineeringISBN:9780134746968Author:James W. Nilsson, Susan RiedelPublisher:PEARSONEngineering ElectromagneticsElectrical EngineeringISBN:9780078028151Author:Hayt, William H. (william Hart), Jr, BUCK, John A.Publisher:Mcgraw-hill Education,