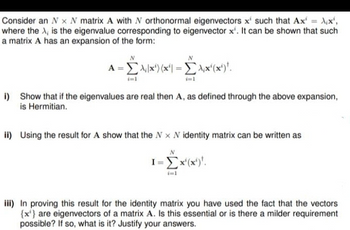
Consider an Nx N matrix A with N orthonormal eigenvectors x such that Ax' = ₁x¹, where the X, is the eigenvalue corresponding to eigenvector x'. It can be shown that such a matrix A has an expansion of the form: A = ΣA₁x¹)(x¹=A₁x²(x¹). i) Show that if the eigenvalues are real then A, as defined through the above expansion, is Hermitian. ii) Using the result for A show that the Nx N identity matrix can be written as N I= Σx'(x¹)¹. iii) In proving this result for the identity matrix you have used the fact that the vectors {x} are eigenvectors of a matrix A. Is this essential or is there a milder requirement possible? If so, what is it? Justify your answers.

The matrix A = has eigenvalues λ = 1, 2, 3, 4. Is - B -4 5 5 5 -6 26 6 -7 0 3 7 -8 5 5 9 = -3 55 5 -6 3 6 6 -7 0 4 7 -8 5 5 10 diagonalizable? Without calculating eigenvectors or eigenvalues, explain why or why not (you will lose points if you calculate eigenvectors or eigenvalues).

Suppose v is an eigenvector of a matrix A with corresponding eigenvalue A, and suppose c is a (fixed) scalar. Show based on the definition of eigenvector and eigenvalue that v is an eigenvector of cI – A with corresponding eigenvalue c – A.