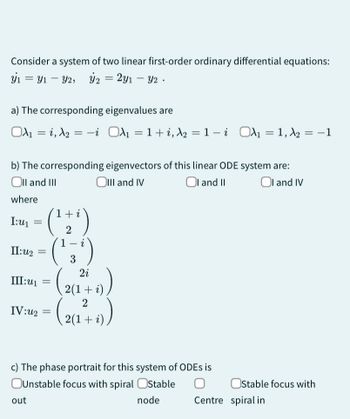
Consider a system of two linear first-order ordinary differential equations: y₁ = y₁ - y2, y2 = 2y1 - y2 a) The corresponding eigenvalues are OA₁i, A₂ = -i OA₁ = 1+i, A₂ = 1-i O₁ = 1, A₂ = -1 = b) The corresponding eigenvectors of this linear ODE system are: Oll and III OI and IV OIII and IV OI and II where I:U₁ = II:u₂ = III:u₁ = IV:42 out 1+ 1 + i 2 (¹-1) 3 2i ( 2 ( 1² + i)) (2(1²+ 4)) i) c) The phase portrait for this system of ODEs is OUnstable focus with spiral Stable node Ostable focus with Centre spiral in

For the system of differential equations, X1, X2 a) Find the characteristic polynomial of the matrix of coefficients A. CA(X) b) Find the eigenvalues of A. Enter the eigenvalues as a list in ascending order separated by commas. U₁ = U2 = = y' = c) Find the eigenvectors assuming u₁ is the eigenvector associated with the smaller eigenvalue X₁ and u2 is the eigenvector associated with the larger eigenvalue A2. 5 2 - 1 Y2 (t) d) Determine two linearly independent solutions to the system. Enter the first solution in the format y₁ (t) y = = y₁ (t) Enter the second solution in the format y₂(t) = ƒ2(t) (v3h₁(t) + v₁h₂(t)) = : fi(t) (vigi(t) – v2g2(t)) . +

Suppose the eigenvalues associated with a 2 by 2 matrix of a system of linear ODE's are complex conjugates. (a) Use Euler's formula to rewrite the solution in the form x(t) = u(t) + iv(t). (b) Show that x(t) = u(t) + v(t) is also a solution of the system.

Suppose a system of first-order linear differential equations has eigenvalues A₁ = -3, A2=-2.3. The equilibrium point (origin) can be classified as: O Flux Point O Saddle Point O Origin Point O Stable Node (Sink) O Unstable Node (Source)

Let M be a 2 x 2 matrix with eigenvalues A₁ = 0.3, A2 = -0.25 with corresponding eigenvectors = [2²] ²2² = [9] · V2 Consider the difference equation with initial condition xo That is, write xo = C1V1 + C₂V2 H Write the initial condition as a linear combination of the eigenvectors of M. In general, X = Specifically, X4 V1 = For large k, xk Xk+1 = V₁+ )k v₁+ Mxk A V2 7)k V₂