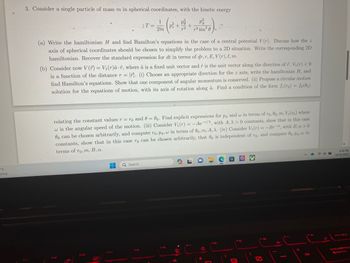
Consider a single particle of mass m in spherical coordinates, with the kinetic energy P² p²+ + 7.2 p² r2 sin² 0 IT= 1 2m (a) Write the hamiltonian H and find Hamilton's equations in the case of a central potential V(r). Discuss how the z axis of spherical coordinates should be chosen to simplify the problem to a 2D situation. Write the corresponding 2D hamiltonian. Recover the standard expression for dt in terms of dr, r, E, V(r), l, m. (b) Consider now V(r) = V₁(r)âà f, where â is a fixed unit vector and is the unit vector along the direction of F. V₁ (r) <0 is a function of the distance r = r. (i) Choose an appropriate direction for the z axis, write the hamiltonian H, and find Hamilton's equations. Show that one component of angular momentum is conserved. (ii) Propose a circular motion solution for the equations of motion, with its axis of rotation along â. Find a condition of the form fr(ro) = fe(o)

1

Calculate the constants b₁ and b2 in the the following equation 1 Imax d²x(t) for the condition (0) ) = xmax, the maximum extension of the oscillator. What is v(0) for this condition? Match the items in the left column to the appropriate blanks in the equations on the right. Make certain each equation is complete before submitting your answer. ?). dt² ∞ b₂ 0 t=0 dx (t) (da)₁-0 dt t=0 b₁ x(t) v(0) = xmax = b₁ co Therefore, b₁ = 0 + b₂ sin and b₂ = (√5.0) k μl • (√) +0 k COS 00 (√5-0) x(t) = b₁ cos Reset t + b₂ sin 2 sin (√) Help

Lagrangian Dynamics Ep = 0 A pendulum of length / and mass m is mounted on a block of mass M. The block can move freely without friction on a horizontal surface as shown in the adjacent figure H. 1. Find the velocity of mass m, w.r.t the origin O 2. Write the Lagrangian of the system 3. Derive the Euler Lagrange equations