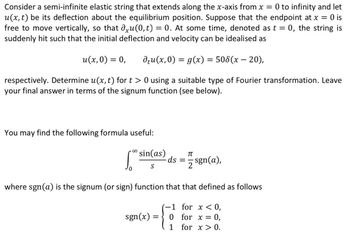
Consider a semi-infinite elastic string that extends along the x-axis from x = 0 to infinity and let u(x, t) be its deflection about the equilibrium position. Suppose that the endpoint at x = 0 is free to move vertically, so that axu(0,t) = 0. At some time, denoted as t = 0, the string is suddenly hit such that the initial deflection and velocity can be idealised as u(x,0) = 0, du(x,0) = g(x) = 508(x - 20), respectively. Determine u(x, t) for t> 0 using a suitable type of Fourier transformation. Leave your final answer in terms of the signum function (see below). You may find the following formula useful: 0 sir sin(as) S ds = sgn(x): = TT zsgn(a), where sgn(a) is the signum (or sign) function that that defined as follows -1 for x < 0, 0 for x = 0, 1 for x > 0.

The answer is correct for simplifying, but please explain the step that is circled in red. I don't understand how sec2a turns into the square root of 1+tan^2 2a

l Xfinity Mobile 10:35 AM O 66% A webassign.net Your last submission is used for your score. 1. [-/1 Points) DETAILS LARPCALC10 6.5.011. MY NOTES ASK YOUR TEACHER Match the complex number with its representation in the complex plane. 3 - Imaginary axis Imaginary ахis Imaginary axis (2 1) 1+ 1+ (2, 0) Real Real Real i 2 axis axis -1 -1 (3, –1) -2+ -2+ Imaginary Imaginary Imaginary ахis ахis ахis 1- 3•(0, 3) Real Real axis 2- i 2 (-2, -1) axis 1- Real (-1, -3) i axis -3- -1- Imaginary Imaginary axis axis (-3, 1) 1- 2- • (1, 2) Real axis T- -2 -i Real -2+ Need Help? Read It 2. [-/1 Points] DETAILS LARPCALC10 6.5.021. MY NOTES ASK YOUR TEACHER PRACTICE ANOTHER Find the sum of the complex numbers in the complex plane. (3 +) + (2 + 71) Need Help? Read It 3. [-/2 Points] DETAILS LARPCALC10 6.5.037. MY NOTES ASK YOUR TEACHER PRACTICE ANOTHER Plot the complex number and its complex conjugate. 2+ 7i Imaginary axis Imaginary axis 2 - Real axis Real axis -10 -8 -6 -4 -2. 2 4 6 8 10 -10- 2 4 6 8 10…

Ok, I see how sinx derives to cosx using that formula sin^2x + cos^2x = 1, thx it helps