Consider a ray incident on the end of a fiber-optic cable as illustrated in the figure below. In the experiment, you will explore the behavior of the fiber as a function of the angle of incidence a. When a ray strikes the end of the fiber it is bent toward the normal. By Snell's law, the angle inside the core is sin core (no/n₁) sin a. Once inside the core, the ray travels until it strikes the cladding of the fiber. The angle of incidence at the cladding i is the complementary angle of core. If i is a small angle, the ray will propagate into the cladding and be lost from the fiber. If i is large, however, the ray will be internally reflected and bounce down the fiber. By Snell's law, the critical angle for the ray to be internally reflected is sin icrit = n₂/n₁. In the experiment, you cannot directly measure angles inside the fiber but you can measure a. Using the facts that, (i) for complementary angles, sin core = cos i, (ii) the trigonometric identity cos i = √I sin² i, and (iii) the index of refraction of air is no = 1, it can be shown that sin acrit = √√²-n². This quantity is known as the numerical aperture (N.A.) of the fiber. For a cable with a core that has an index of refraction of 1.5 and a cladding with an index of refraction of 1.25, what do you expect the critical angle acrit to be (in degrees)? 40.8 Cladding → Core → ecore refracted reflected
Consider a ray incident on the end of a fiber-optic cable as illustrated in the figure below. In the experiment, you will explore the behavior of the fiber as a function of the angle of incidence a. When a ray strikes the end of the fiber it is bent toward the normal. By Snell's law, the angle inside the core is sin core (no/n₁) sin a. Once inside the core, the ray travels until it strikes the cladding of the fiber. The angle of incidence at the cladding i is the complementary angle of core. If i is a small angle, the ray will propagate into the cladding and be lost from the fiber. If i is large, however, the ray will be internally reflected and bounce down the fiber. By Snell's law, the critical angle for the ray to be internally reflected is sin icrit = n₂/n₁. In the experiment, you cannot directly measure angles inside the fiber but you can measure a. Using the facts that, (i) for complementary angles, sin core = cos i, (ii) the trigonometric identity cos i = √I sin² i, and (iii) the index of refraction of air is no = 1, it can be shown that sin acrit = √√²-n². This quantity is known as the numerical aperture (N.A.) of the fiber. For a cable with a core that has an index of refraction of 1.5 and a cladding with an index of refraction of 1.25, what do you expect the critical angle acrit to be (in degrees)? 40.8 Cladding → Core → ecore refracted reflected
College Physics
11th Edition
ISBN:9781305952300
Author:Raymond A. Serway, Chris Vuille
Publisher:Raymond A. Serway, Chris Vuille
Chapter1: Units, Trigonometry. And Vectors
Section: Chapter Questions
Problem 1CQ: Estimate the order of magnitude of the length, in meters, of each of the following; (a) a mouse, (b)...
Related questions
Concept explainers
Refraction of Light
Refraction is a change in the direction of light rays when they travel from one medium to another. It is the bending of light when it goes through different media.
Angle of Refraction
Light is considered by many scientists to have dual nature, both particle nature and wave nature. First, Particle nature is one in which we consider a stream of packets of energy called photons. Second, Wave nature is considering light as electromagnetic radiation whereas part of it is perceived by humans. Visible spectrum defined by humans lies in a range of 400 to 700 nm wavelengths.
Index of Refraction of Diamond
Diamond, the world’s hardest naturally occurring material and mineral known, is a solid form of the element carbon. The atoms are arranged in a crystal structure called diamond cubic. They exist in a huge variety of colours. Also, they are one of the best conductors of heat and have a very high melting point.
Question
100%
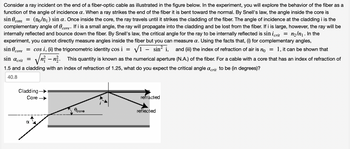
Transcribed Image Text:Consider a ray incident on the end of a fiber-optic cable as illustrated in the figure below. In the experiment, you will explore the behavior of the fiber as a function of the angle of incidence α. When a ray strikes the end of the fiber it is bent toward the normal. By Snell's law, the angle inside the core is
sin θ_core = (n₀/n₁) sin α.
Once inside the core, the ray travels until it strikes the cladding of the fiber. The angle of incidence at the cladding i is the complementary angle of θ_core. If i is a small angle, the ray will propagate into the cladding and be lost from the fiber. If i is large, however, the ray will be internally reflected and bounce down the fiber. By Snell's law, the critical angle for the ray to be internally reflected is
sin i_crit = n₂/n₁.
In the experiment, you cannot directly measure angles inside the fiber but you can measure α. Using the facts that, (i) for complementary angles,
sin θ_core = cos i,
(ii) the trigonometric identity
cos i = √(1 - sin² i),
and (iii) the index of refraction of air is n₀ = 1, it can be shown that
sin α_crit = √(n₁² - n₂²).
This quantity is known as the numerical aperture (N.A.) of the fiber. For a cable with a core that has an index of refraction of 1.5 and a cladding with an index of refraction of 1.25, what do you expect the critical angle α_crit to be (in degrees)?
40.8
**Diagram Explanation:**
The diagram shows the structure of a fiber-optic cable with two main parts: the core and the cladding. A light ray enters the core at an angle α. As it enters, it refracts and bends towards the normal, traveling through the core with an angle θ_core. The ray then reaches the boundary between the core and cladding, where it encounters the critical angle i. If this angle is greater than i_crit, the ray reflects internally and continues to bounce down the fiber. Otherwise, it refracts out into the cladding and is lost.
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution!
Trending now
This is a popular solution!
Step by step
Solved in 5 steps with 5 images
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, physics and related others by exploring similar questions and additional content below.Recommended textbooks for you
College Physics
Physics
ISBN:
9781305952300
Author:
Raymond A. Serway, Chris Vuille
Publisher:
Cengage Learning
University Physics (14th Edition)
Physics
ISBN:
9780133969290
Author:
Hugh D. Young, Roger A. Freedman
Publisher:
PEARSON
Introduction To Quantum Mechanics
Physics
ISBN:
9781107189638
Author:
Griffiths, David J., Schroeter, Darrell F.
Publisher:
Cambridge University Press
College Physics
Physics
ISBN:
9781305952300
Author:
Raymond A. Serway, Chris Vuille
Publisher:
Cengage Learning
University Physics (14th Edition)
Physics
ISBN:
9780133969290
Author:
Hugh D. Young, Roger A. Freedman
Publisher:
PEARSON
Introduction To Quantum Mechanics
Physics
ISBN:
9781107189638
Author:
Griffiths, David J., Schroeter, Darrell F.
Publisher:
Cambridge University Press
Physics for Scientists and Engineers
Physics
ISBN:
9781337553278
Author:
Raymond A. Serway, John W. Jewett
Publisher:
Cengage Learning
Lecture- Tutorials for Introductory Astronomy
Physics
ISBN:
9780321820464
Author:
Edward E. Prather, Tim P. Slater, Jeff P. Adams, Gina Brissenden
Publisher:
Addison-Wesley
College Physics: A Strategic Approach (4th Editio…
Physics
ISBN:
9780134609034
Author:
Randall D. Knight (Professor Emeritus), Brian Jones, Stuart Field
Publisher:
PEARSON