
Advanced Engineering Mathematics
10th Edition
ISBN: 9780470458365
Author: Erwin Kreyszig
Publisher: Wiley, John & Sons, Incorporated
expand_more
expand_more
format_list_bulleted
Question
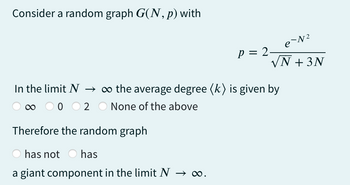
Transcribed Image Text:Consider a random graph G(N, p) with
e-№²
√N + 3N
Therefore the random graph
has not has
a giant component in the limit N → ∞.
P = 2₁
In the limit N → ∞o the average degree (k) is given by
∞
None of the above
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by stepSolved in 4 steps with 30 images

Knowledge Booster
Similar questions
- 1 [Fill in the Blank] Let X₁X,₁,..., X, be a sequence of i.i.d. random variables, each with mean E(X) = μ and standard deviation o, we defineX, X₁+X₂ + ··· +Xµ = n The (Weak) Law of Large Numbers (WLLN) states for all & >0 then, Which of the following statement is true? lim p{Xn-μ|ze}=0 2- A. B. lim pin-uze}=1 C. lim p Xn-μarrow_forwardA researcher claims that the number of homicide crimes by season is uniformly distributed. To test this claim, you randomly select 1,205 homicides from a recent year and record the season when each happened. The table shows the results. At α = 0.01, test the researcher's claim. Season Frequency, f Spring 301 Summer 319 Fall 299 Winter 286 State H0 and Ha and identify the claim. H0: The distribution of the number of homicide crimes by season Ha: The distribution of the number of homicide crimes by seasonarrow_forwardq6, a and b pleasearrow_forward
- Could you solve (d),(e),(f)? Thank you.arrow_forward(b) Describe the sigma-field F2. (Write down All elements of F2). Hint: How many outcomes if we toss a coin two times? Then how many subsets for the set including all of the outcomes? Given {N(t)} is a Poisson process, please calculate (a) P(N(4)=2|N(2)=1) (b) E[N(4)|N(2)=1] (c) Var[N(4)|N(2)=1] (d) P(N(4)=2|N(10)=5), also calculate E[N(4)|N(10)=5] and Var[N(4)|N(10)=5]arrow_forwardUsing the joint distribution describing X and Y, the following formula was derived E(XKym) = 2km+3(m+k)+4 (k+1)(k+2)(m+1)(m+2) where k and m are both nonnegative constants. Simplify Var( 2X - 3Y) [ Select ] - Var(X) [Select] [ Select ] Var(Y) [Select] [ Select ] Cov(X,Y)arrow_forward
- Consider a Poisson process of intensity λ > 0 events per hour. Suppose that exactly one event occurs in the first hour. Let X be the time at which this event occurs, measured in hours (so that the possible values of X are the reals in the interval [0, 1]). (a) What is P (0 ≤ X ≤ a) for a ∈ [0, 1]? (Of course, we are conditioning on exactly one event occurring in the first hour, as described.) Hint: Consider the number of events that occur in [0, a] and in (a, 1], similar to question #5 on the previous homework. (b) What is the distribution of X, under the same conditions? (You might want to wait until Monday’s class after break to do this last part.)arrow_forward7abcarrow_forwardConsider a random graph G(N, p) with .In the limit N → ∞ the average degree (k) is given by 0 0 0 0 2/3 ○ None of the above Therefore the random graph O has not O has a giant component in the limit N → ∞. P = e² In 2 3N3/2arrow_forward
- Use the Taylor series in Table 11.5 to find the first four nonzero terms of the Taylor series for the following functions centered at 0. 35. v aniwollo 36. sin x?arrow_forwardSuppose X₁, X2,... are i.i.d. with moment generating function Mx(t). Let N be a nonnegative integer-valued random variable, independent of the X₁, with moment generating function My(t). Consider the random sum S X₁. In terms of Mx and MN, find the moment generating function of S. Use this to rederive the mean of S. Specializing to the case where the X, are Poisson with mean λ and N is Poisson with mean μ, what is the moment generating function of S?arrow_forwardFor the Normal-Cauchy Bayes estimator: 8(x) = 5⁰⁰ ง -e-(x-9) ²/² dv -∞0⁰ 1 +92 e 1 ∞ 1 +9² e−(x−v)² dv Build an Accept-Reject algorithm based on the Cauchy candidate to generate a sample from the posterior distribution and then deduce the estimator.arrow_forward
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
 Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated
Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education
Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY
Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,


Advanced Engineering Mathematics
Advanced Math
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:9780073397924
Author:Steven C. Chapra Dr., Raymond P. Canale
Publisher:McGraw-Hill Education

Introductory Mathematics for Engineering Applicat...
Advanced Math
ISBN:9781118141809
Author:Nathan Klingbeil
Publisher:WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,

