Question

Transcribed Image Text:9:39 A
✰edfinity.com
(Challenge Problem) Charge is
distributed throughout a spherical
volume of radius R with a density
p = ar², where a is a constant (of
unit C/m5). Determine the electric
field due to the charge at points
both inside and outside the sphere.
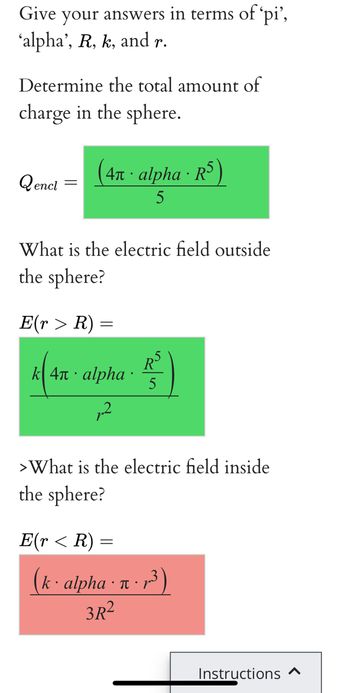
Give your answers in terms of ‘pi’,
‘alpha', R, k, and r.
Determine the total amount of
charge in the sphere.
(4ñ· alpha · R5)
Qen
encl
..lLTE 36
What is the electric field outside
the sphere?
E(r > R) =
k ( 4n - alpha · RS²)
5
₂.2
>What is the electri Instructions
Transcribed Image Text:Give your answers in terms of 'pi',
‘alpha’, R, k, and r.
Determine the total amount of
charge in the sphere.
Qencl
=
(4π- alpha - R5)
5
What is the electric field outside
the sphere?
E(r > R) =
4π
R
alpha. 5
₂²
>What is the electric field inside
the sphere?
E(r < R) =
3
(k · alpha · ñ·³)
3R²
Instructions ^
Expert Solution
arrow_forward
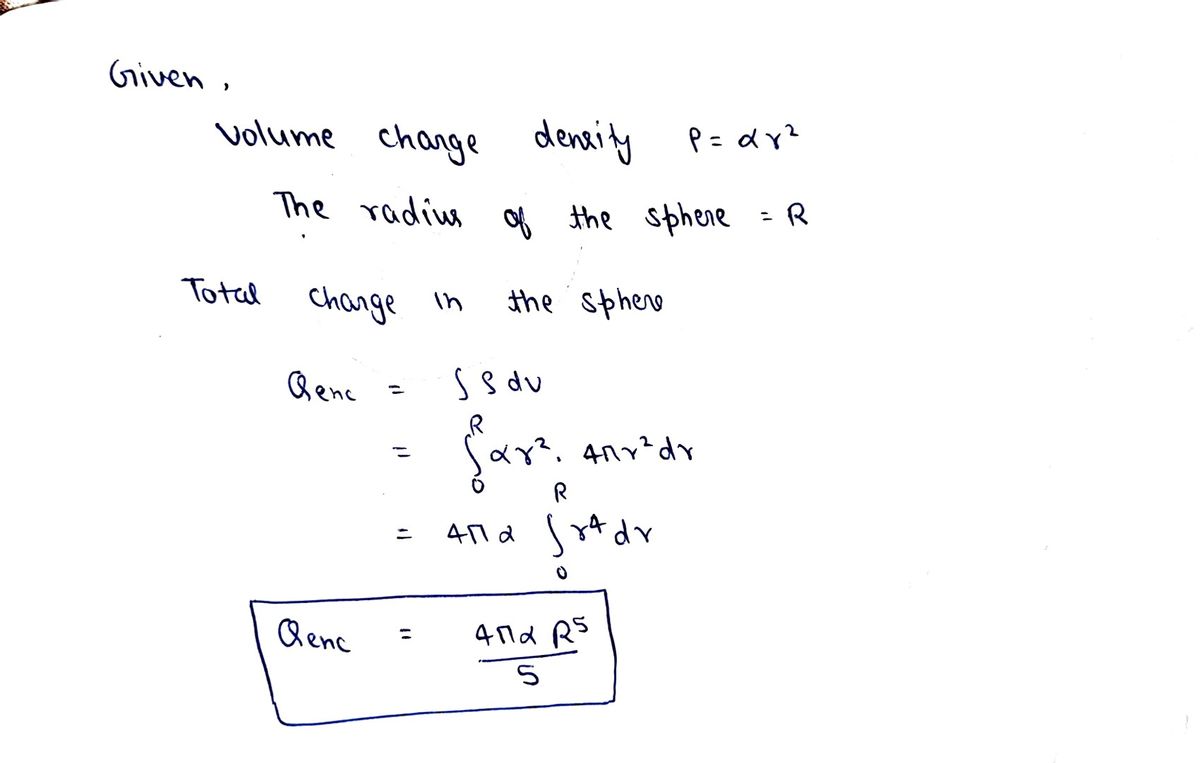
Step 1: Total amount of charge in the sphere
Step by stepSolved in 4 steps with 4 images

Knowledge Booster
Similar questions
- Please solve and answer the question correctly. Be sure to use the correct units and work the problem carefully. Thank you!!arrow_forwardCharge Q is distributed uniformly throughout the volume of an insulating sphere of radius R = 4.00 cm. At a distance of r = 8.00 cm from the center of the sphere, the electric field due to the charge distribution has magnitude 740 N/C. Part A What is the volume charge density for the sphere? Express your answer with the appropriate units. HA p = Value Units Part B What is the magnitude of the electric field at a distance of 2.00 cm from the sphere's center? Express your answer with the appropriate units. E= Value Unitsarrow_forwardTwo point charges are located on a horizontal line as Shown in diagram on the right. The first small circle Represents the charge Q1 the left and small circle on right Q2 . In the middle between the two charges is point P. a) Find the net electric field due to the 2 charges at point P by finding the magnitude and direction of each one if Q1 =3μC and Q2 =5μC and distance between charges = 50 cm.arrow_forward
arrow_back_ios
arrow_forward_ios