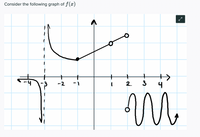
**Title: Understanding the Graph of Function \( f(x) \)** **Graph Explanation:** The image presents the graph of a function \( f(x) \) with distinct characteristics across different intervals on the x-axis. Below is a detailed explanation of the graph’s components: 1. **Discontinuity at x = -3:** - There is a vertical dashed line at \( x = -3 \), indicating a vertical asymptote. - As \( x \) approaches -3 from the left, \( f(x) \) sharply decreases towards negative infinity. 2. **Curve and Behavior from x = -3 to x = 1:** - Between \( x = -3 \) and \( x = 1 \), the function appears to be a decreasing curve that transitions into a linear segment. - There is a filled dot at approximately \( (-1, 0) \), indicating that the point is included in the graph. 3. **Linear Segment from x = 1 to x = 2:** - The graph includes an open circle at \( x = 1 \) and \( x = 2 \). This signifies that these points are not part of the graph, indicating possible discontinuities or endpoints. 4. **Oscillating Behavior Beyond x = 2:** - Beyond \( x = 2 \), the graph demonstrates an oscillating pattern, suggesting periodic behavior. - The oscillation includes an open circle, indicating a gap or undefined point in the function. Overall, this graph showcases different behaviors including discontinuities, linear sections, and oscillations as \( x \) ranges from negative to positive values. Understanding these elements can greatly contribute to comprehending the characteristics of function \( f(x) \).