Question
The force required to compress a non-standard spring varies as the spring is compressed, as shown by the plot of force vs displacement. Distances are x1 = 10, x2 = 25 cm, x3 = 55 cm, and x4=75cm, and the forces are F1 = 110 N and F2 = -60N. Calculate the work done in Joules, on the spring as it is compressed from origin to point x4.
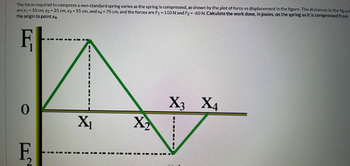
Transcribed Image Text:The force required to compress a non-standard spring varies as the spring is compressed, as shown by the plot of force vs displacement in the figure. The distances in the figure
arex 10cm x 25cm,xy-55 cm, and x 75 cm, and the forces are F1-110 N and F-60 N. Calculate the work done, in joules, on the spring as it is compressed from
the origin to point x
F
0
F
X3 X4
X2
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by stepSolved in 3 steps with 2 images

Knowledge Booster
Similar questions
- The graph shows the xx component of a force that acts on an object that moves on the x axis. The vertical spacing between adjacent grid lines is 4.93N, while the horizontal spacing between adjacent grid lines is 1.0m. What is the work, in joules, done by the force as the object moves from x=4m to x=5m?arrow_forwardThe force required to compress a non-standard spring as a function of displacement is given by the equationF(x) = -Asin(bx) + kx,where A = 11 N, b = 11 rad/m, and k = 78 N/m. Part (b) Calculate the work done in joules as the spring is compressed from x = 0 to x1 = 32 cm. Part (c) Calculate the work done in joules as the spring is compressed from x1 = 32 cm to x2 = 69 cmarrow_forwardA 12.0 kg box is given an initial push that starts it sliding across the floor. It eventually comes to a stop. If the box has an initial velocity of 3.5 m/s, how much work is done by friction to cause it to come to a stop? (Yes, you are correct, you do not know the coefficient of friction.)arrow_forward
- An object moves in the x-y plane while acted on by the force F(x,y) = k1yi + k2xj, where k1 and k2 are constants with units of N/m. The object moves along a straight line path from Cartesian coordinate (x1, y1) to (x2, y2). Write an expression for the work done on the object by this force.arrow_forwardThe figure gives the acceleration of a 5.0 kg particle as an applied force moves it from rest along an x axis from x-0 tox-9.0 m. The scale of the figure's vertical axis is set by a,- 6.0 m/s². How much work has the force done on the particle when the particle reaches (a) x-4.0 m, (b)x - 7.0 m, and (c) x-9.0 m? What is the particle's speed and direction (give positive answer if the particle moves along x axis in positive direction and negative otherwise) of travel when it reaches (d) x - 4.0 m, (e) x - 7.0 m, and (f)x - 9.0 m? (a) Number i (b) Number i (c) Number i (d) Number i (e) Number i (f) Number i (₂8/) v Units Units Units Units Units Units 2 x (m) < <arrow_forwardThe force on a particle is directed along an x axis and given by F = F0(x/x0 - 1) where x is in meters and F is in Newtons. If F0 = 1.6 N and x0 = 4.6 m, find the work done by the force in moving the particle from x = 0 to x = 2x0 m.arrow_forward
arrow_back_ios
arrow_forward_ios