Advanced Engineering Mathematics
10th Edition
ISBN: 9780470458365
Author: Erwin Kreyszig
Publisher: Wiley, John & Sons, Incorporated
expand_more
expand_more
format_list_bulleted
Related questions
Question
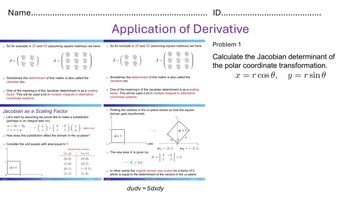
Calculate the Jacobian determinant of
the polar coordinate transformation.
X=rcosθ
Y=rsinθ
Transcribed Image Text:Name......
Lac
So for example in 2D and 3D (assuming square matrices) we have:
J =
of
oh oh
8f₂ მ.
Oy
Or
af
+
J= of
u= 3x - 2y
v=x+y
A = 1
55 55
of
Sometimes the determinant of this matrix is also called the
Jacobian too.
of
dz
fa fa fa
Jy
Da
One of the meaning's of the Jacobian determinant is as a scaling
factor. This will be used a lot in multiple integrals in alternative
coordinate systems.
Jacobian as a Scaling Factor
Let's start by assuming we would like to make a substitution
(perhaps in an integral later on):
- (9)-(3)()
How does this substitution affect the domain in the xy-plane?
Consider the unit square with area equal to 1.
a fa
(x,y)
(0,0)
(1,0)
(0, 1)
(1,1)
Transforming vertices
(u, v)
Matrix form
(0,0)
(3,1)
(-2,1)
(1,2)
.….... ID.…........
Application of Derivative
So for example in 2D and 3D (assuming square matrices) we have:
oh oh oh
oh oh oh
By
- (
J=
of
dz
ofa
Əz
A = 1
of
By
01₂
Jy
Sometimes the determinant of this matrix is also called the
Jacobian too.
J=
One of the meaning's of the Jacobian determinant is as a scaling
factor. This will be used a lot in multiple integrals in alternative
coordinate systems.
Plotting the vertices in the uv-plane shows us how the square
domain gets transformed.
are:
The new area A' is given by:
afa
Oz
w₁ (3,1)
=
A' =
A'= ?
3-2
-|- -|-5
=5
dudv = 5dxdy
Wy
==
w₂ (-2,1)
A'=5A
In other words the original domain was scaled by a factor of 5
which is equal to the determinant of the vectors in the uv-plane.
Advanced Calculu
PEARSON
Problem 1
Calculate the Jacobian determinant of
the polar coordinate transformation.
x = r cos 0, y = rsin
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by stepSolved in 3 steps with 2 images
Knowledge Booster
Similar questions
- Consider the following. T is the clockwise rotation (0 is negative) of 60° in R2, v = (1, 2). (a) Find the standard matrix A for the linear transformation T. A = (b) Use A to find the image of the vector v. T(v) =arrow_forwardYou should be able to do this in your head. Hint: This is a rotation matrix. [cos(6) [sin (0) - sin( cos(0) cos (0) x -sin (0) sin (0) x cos (0) Xarrow_forward
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
- Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated
Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education
Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY 
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Advanced Engineering Mathematics
Advanced Math
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Wiley, John & Sons, Incorporated
Numerical Methods for Engineers
Advanced Math
ISBN:9780073397924
Author:Steven C. Chapra Dr., Raymond P. Canale
Publisher:McGraw-Hill Education
Introductory Mathematics for Engineering Applicat...
Advanced Math
ISBN:9781118141809
Author:Nathan Klingbeil
Publisher:WILEY
Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,