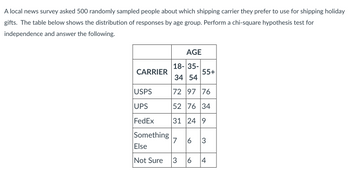
Part of performing a hypothesis test to determine the independence between shipping carrier preference and age is to calculate the expected number of survey respondents in each cell of the table above. Assuming independence between the two variables, how many 18-34 year olds would we expect to prefer USPS? ### Survey on Preferred Shipping Carriers for Holiday GiftsA local news survey was conducted with a sample size of 500 individuals to discover which shipping carrier people prefer for shipping holiday gifts. The survey results were categorized by age groups, and the distribution of responses is depicted in the table below.#### Distribution of Responses by Age Group| **Carrier** | **18-34** | **35-54** | **55+** ||-------------------|-----------|-----------|---------|| **USPS** | 72 | 97 | 76 || **UPS** | 52 | 76 | 34 || **FedEx** | 31 | 24 | 9 || **Something Else**| 7 | 6 | 3 || **Not Sure** | 3 | 6 | 4 |### InstructionsUsing the above data, you are required to perform a chi-square hypothesis test for independence. This will help to determine whether the preference for shipping carriers is independent of age group among the survey respondents.#### Steps to Perform Chi-square Test for Independence:1. **State the Hypotheses:** - Null Hypothesis (\(H_0\)): The preference for shipping carriers is independent of age. - Alternative Hypothesis (\(H_a\)): The preference for shipping carriers is not independent of age.2. **Construct the Contingency Table:** - Use the provided data to construct a contingency table for the chi-square calculation.3. **Calculate the Expected Frequencies:** - Use the formula \( E = \frac{(Row \ Total \times Column \ Total)}{Grand \ Total} \).4. **Compute the Chi-square Statistic:** - Apply the formula \( \chi^2 = \sum \frac{(O - E)^2}{E} \), where \(O\) represents the observed frequency and \(E\) represents the expected frequency.5. **Determine the Degrees of Freedom:** - Degrees of Freedom (df) = (Number of rows - 1) * (Number of columns - 1).6. **Compare with the Critical Value:** - Compare the calculated chi-square statistic with the critical value from the chi-square distribution table for the determined degrees of freedom and significance level (e.g., \( \alpha

here given , table of CARRIER and AGE here for Expected number of Survey Respondent for each cell…

Answered: calculate the expected number of survey…

calculate the expected number of survey respondents in each cell of the table above. Assuming independence between the two variables, how many 18-34 year olds would we expect to prefer USPS?

MATLAB: An Introduction with Applications

6th Edition

ISBN:9781119256830

Author:Amos Gilat

Publisher:Amos Gilat

Chapter1: Starting With Matlab

Section: Chapter Questions

Problem 1P

See similar textbooks

Similar questions

The table below summarizes data from a survey of a sample of women. Using a 0.01 significance level, and assuming that the sample sizes of 900 men and 400 women are predetermined, test the claim that the proportions of agree/disagree responses are the same for subjects interviewed by men and the subjects interviewed by women. Does it appear that the gender of the interviewer affected the responses of women? Gender of Interviewer Man Woman Women who agree 625 330 Women who disagree 275 70 Compute the test statistic, rounding to three decimal places. Find the critical value(s). (Round to three decimal places) What is the conclusion based on the hypothesis test?
A survey was conducted to determine whether hours of sleep per night are independent of age. A sample of individuals was asked to indicate the number of hours of sleep per night with categorical options: fewer than 6 hours, 6 to 6.9 hours, 7 to 7.9 hours, and 8 hours or more. Later in the survey, the individuals were asked to indicate their age with categorical options: age 39 or younger and age 40 or older. Sample data follow. Age Group Hours of Sleep 39 or younger 40 or older Fewer than 6 38 36 6 to 6.9 60 57 7 to 7.9 76 74 8 or more 66 93 (a) Conduct a test of independence to determine whether hours of sleep are independent of age. (b) What is your estimate of the percentages of individuals who sleep fewer than 6 hours, 6 to 6.9 hours, 7 to 7.9 hours, and 8 hours or more per night?
A survey of employees at a large financial organisation was conducted to determine the overall sentiment towards various aspects of how the company operates. 30 randomly selected departments in the organisation participated in the study. Every employee in these 30 randomly selected departments filled in a questionnaire, and the data were aggregated to calculate the following two variables of interest: Rating The percentage of favourable responses (in each department) to the overall running of the company. ComplaintsThe percentage of favourable responses (in each department) to how the company handles employee complaints Source: Chatterjee, S. and Price, B. (1977) Regression Analysis by Example. New York: Wiley. (Section 3.7, p.68ff of 2nd ed. (1991).) A simple linear regression was conducted. A scatter plot of Rating versus Complaints is shown in Figure 1 and the results of the regression analysis are shown in Table 1. Rating 80 70 60 50 40 40 Model 1 Figure 1: Rating versus…
An owner of an ice cream shop wants to investigate whether a new training program affects the scooping skills of ice cream shop employees. To assess this, the owner divides employees into two groups: those who receive training (CT) and those who do not (NT). The owner evaluates their scooping skills using a scooping proficiency test. The dataset includes the test scores of the NT (no training) group: 15.00, 7.00, 18.00, 10.00, 6.00, and 20.00, and the CT (trained) group: 15.00, 10.00, 14.00, 7.00, 21.00, and 21.00. The owner is aware of the known population mean for scooping proficiency, which is 20. The hypothesis is that employees who did not receive training (NT) will likely have lower scooping proficiency than the population mean. To investigate this hypothesis, the owner conducts a one-sample t-test with a significance level (alpha) set at 0.05. The objective is to determine if the mean scooping proficiency of the NT group is significantly different from the established population…
A company is carrying out a consumer satisfaction survey for two of its products, product A and product B. The company believes that product A receives a higher proportion of satisfied customers than product B. Out of the 80 product A customers surveyed, 52 said they were satisfied with the product and out of the 100 product B customers surveyed, 42 said they were satisfied with the product. Use these sample statistics to compare the proportion of product A customers that are satisfied, p 1, and the proportion of product B customers that are satisfied, p 2. i) Carry out a hypothesis test for a significant difference between the two population proportions, at significance level α = 0.05. The hypotheses being tested are: H 0: p 1 - p 2 = 0 H a: p 1 - p 2 ≠ 0. Complete the test by filling in the blanks in the following:An estimate of the difference in population proportions is .The standard error is (3 dec places).The distribution is (examples: normal / t12 /…
A survey was conducted to determine whether hours of sleep per night are independent of age. A sample of individuals was asked to indicate the number of hours of sleep per night with categorical options: fewer than 6 hours, 6 to 6.9 hours, 7 to 7.9 hours, and 8 hours or more. Later in the survey, the individuals were asked to indicate their age with categorical options: age 39 or younger and age 40 or older. Sample data follow. Hours of Sleep Age Group 39 or younger 40 or older Fewer than 6 40 38 6 to 6.9 60 57 7 to 7.9 77 75 8 or more 63 90 (a) Conduct a test of independence to determine whether hours of sleep are independent of age. State the null and alternative hypotheses. H0: Hours of sleep per night is not independent of age.Ha: Hours of sleep per night is independent of age. H0: Hours of sleep per night is independent of age.Ha: Hours of sleep per night is not independent of age. H0: The proportion of people who get 8 or more hours of sleep per…
The table below summarizes data from a survey of a sample of women. Using a 0.05 significance level, and assuming that the sample sizes of 800 men and 400 women are predetermined, test the claim that the proportions of agree/disagree responses are the same for subjects interviewed by men and the subjects interviewed by women. Does it appear that the gender of the interviewer affected the responses of women? Gender of Interviewer Man Woman Women who agree 535 344 Women who disagree 265 56 Click here to view the chi-square distribution table. LOADING... Identify the null and alternative hypotheses. Choose the correct answer below. A. H0: The proportions of agree/disagree responses are different for the subjects interviewed by men and the subjects interviewed by women. H1: The proportions are the same. B. H0: The proportions of agree/disagree…
What social media tools do marketers commonly use? The “2015 Social Media Marketing Industry Report” by Social Media Examiner (socialmediaexaminer.com) surveyed marketers who commonly use an indicated social media tool. Surveyed marketers who commonly use an indicated social media tool. Surveyed were both B2B marketers, marketers that primarily target consumers. Suppose the survey was based on 500B2B marketers and 500 B2C marketers and yielded the results in the following table below: SOCIAL MEDIA TOOL BUSINESS FOCUS (B2B) (%) BUSINESS FOCUS (B2C) (%) FACEBOOK 88 96 TWITTER 83 77 For each social media tool, at the 0.05 level of significance, determine whether there is a difference between B2B marketers and B2C marketers in the proportion who used each social media tool. State the complete hypotheses (hypothesis null and hypothesis alternative for question (a) above
A Doctor is interested in the proportion of Lyon county residents who were infected with covid-19 virus. There were 425 covid-19 cases reported among 25474 residents in Lyon county a.What is the experimental unit in this experiment? b.What is the population in this experiment? c. What is a parameters considered in this experiment? d. What is a statistics in this experiment? e. What variables should pollster collect in this experiment?
The National Study of the Changing Work Force conducted a study of 2958 wage and salaried workers on issuesranging from relationships with their bosses to household chores. The data were gathered through hour-longtelephone interview with a nationally representive sample. In response to the questions “What does your job meanto you?” 1538 people responded that they “Receive personal satisfaction from success at work.” Let p be thepopulation proportion of people who receive personal satisfaction from success at work. (a) Find a 95% CI for the true value of p. (b) How large of sample is needed if we wish to be 99% confident that the sample proportion of p is within1% of the true population proportion.