
Advanced Engineering Mathematics
10th Edition
ISBN: 9780470458365
Author: Erwin Kreyszig
Publisher: Wiley, John & Sons, Incorporated
expand_more
expand_more
format_list_bulleted
Question
![(c) Translate the pulse, i.e. move the nonzero value of the func-
tion to a different location while maintaining the width [for
example, let f(t) = 1 for 1 ≤ t ≤ 2 and zero elsewhere; then
try for f(t) = 1 for 2 ≤ t ≤ 3 and zero elsewhere; and so
on.] Show that it obeys the translation property of Laplace
Transforms, L{f(ta)} = e-as F(s).
(d) Widen the pulse, i.e. keep the other eage at zero and in-
crease the width of the nonzero value [for example, extend
to f(t) = 1 for 0 ≤ t ≤ 2 and zero elsewhere; the do for
f(t) = 1 for 0 ≤ t ≤ 3 and zero elsewhere]. Show that
<
you can get the scaling property of the Laplace Transform,
L {f(at)} = F (₁).](https://content.bartleby.com/qna-images/question/5189d69b-68a4-404a-8967-11b47e8a4835/30100ad3-c41c-4a3b-89c1-1ac0ee5f4e4c/uenkwp_thumbnail.jpeg)
Transcribed Image Text:(c) Translate the pulse, i.e. move the nonzero value of the func-
tion to a different location while maintaining the width [for
example, let f(t) = 1 for 1 ≤ t ≤ 2 and zero elsewhere; then
try for f(t) = 1 for 2 ≤ t ≤ 3 and zero elsewhere; and so
on.] Show that it obeys the translation property of Laplace
Transforms, L{f(ta)} = e-as F(s).
(d) Widen the pulse, i.e. keep the other eage at zero and in-
crease the width of the nonzero value [for example, extend
to f(t) = 1 for 0 ≤ t ≤ 2 and zero elsewhere; the do for
f(t) = 1 for 0 ≤ t ≤ 3 and zero elsewhere]. Show that
<
you can get the scaling property of the Laplace Transform,
L {f(at)} = F (₁).
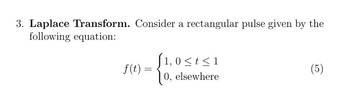
Transcribed Image Text:3. Laplace Transform. Consider a rectangular pulse given by the
following equation:
f(t)
( 1, 0 ≤ t ≤ 1
0, elsewhere
(5)
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution
Trending nowThis is a popular solution!
Step by stepSolved in 4 steps with 3 images

Knowledge Booster
Similar questions
Recommended textbooks for you
 Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated
Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education
Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY
Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,


Advanced Engineering Mathematics
Advanced Math
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:9780073397924
Author:Steven C. Chapra Dr., Raymond P. Canale
Publisher:McGraw-Hill Education

Introductory Mathematics for Engineering Applicat...
Advanced Math
ISBN:9781118141809
Author:Nathan Klingbeil
Publisher:WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,

