
Elementary Geometry For College Students, 7e
7th Edition
ISBN: 9781337614085
Author: Alexander, Daniel C.; Koeberlein, Geralyn M.
Publisher: Cengage,
expand_more
expand_more
format_list_bulleted
Concept explainers
Topic Video
Question
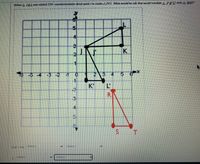
Transcribed Image Text:**Rotation and Translation of Triangles**
In the diagram shown, triangle \( \triangle JKL \) has been rotated 270° counterclockwise about point \( J \), resulting in triangle \( \triangle J'K'L' \).
**Graph Explanation:**
- The graph is a coordinate plane with the x-axis and y-axis ranging from -8 to 8.
- The original triangle \( \triangle JKL \) has the following coordinates: \( J (1, 2) \), \( K (4, 1) \), and \( L (4, 4) \).
- After the rotation, the new triangle \( \triangle J'K'L' \) is formed at the coordinates: \( J' (1, 2) \), \( K' (2, -1) \), and \( L' (-1, -1) \).
- A second triangle \( \triangle RST \) is shown in red, with coordinates \( R (2, -3) \), \( S (5, -4) \), and \( T (5, -7) \).
**Translation Task:**
The task is to determine the rule that will translate triangle \( \triangle J'K'L' \) onto triangle \( \triangle RST \).
**Graph Elements:**
- Points are represented as filled circles.
- Vectors connect the vertices of each triangle.
- \(\triangle JKL\) and \(\triangle J'K'L'\) are in black, while \(\triangle RST\) is in red.
**Interactive Components:**
- There are dropdown menus below the graph to select transformation rules for both x and y coordinates.
**Problem-Solving Strategy:**
To find the translation rule, observe the horizontal and vertical shifts needed to align corresponding points of \( J' \) with \( R \), \( K' \) with \( S \), and \( L' \) with \( T \). Analyze changes in x and y coordinates accordingly.
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution
Trending nowThis is a popular solution!
Step by stepSolved in 2 steps with 1 images

Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, geometry and related others by exploring similar questions and additional content below.Similar questions
Recommended textbooks for you
 Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage,
Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage, Elementary Geometry for College StudentsGeometryISBN:9781285195698Author:Daniel C. Alexander, Geralyn M. KoeberleinPublisher:Cengage Learning
Elementary Geometry for College StudentsGeometryISBN:9781285195698Author:Daniel C. Alexander, Geralyn M. KoeberleinPublisher:Cengage Learning

Elementary Geometry For College Students, 7e
Geometry
ISBN:9781337614085
Author:Alexander, Daniel C.; Koeberlein, Geralyn M.
Publisher:Cengage,

Elementary Geometry for College Students
Geometry
ISBN:9781285195698
Author:Daniel C. Alexander, Geralyn M. Koeberlein
Publisher:Cengage Learning