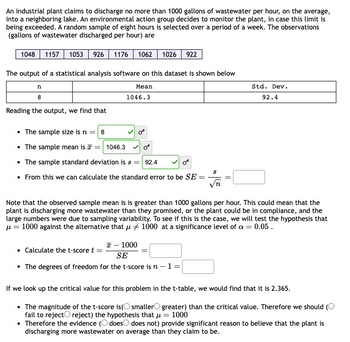
An industrial plant claims to discharge no more than 1000 gallons of wastewater per hour, on the average, into a neighboring lake. An environmental action group decides to monitor the plant, in case this limit is being exceeded. A random sample of eight hours is selected over a period of a week. The observations (gallons of wastewater discharged per hour) are 1048 1157 1053 926 1176 1062 1026 922 The output of a statistical analysis software on this dataset is shown below Mean n 8 Reading the output, we find that • The sample size is n = 8 • The sample mean is a 1046.3 1046.3 • The sample standard deviation is s = 92.4 • From this we can calculate the standard error to be SE √n • Calculate the t-score t - 1000 SE • The degrees of freedom for the t-score is n - 1 = 11 Std. Dev. 92.4 Note that the observed sample mean is is greater than 1000 gallons per hour. This could mean that the plant is discharging more wastewater than they promised, or the plant could be in compliance, and the large numbers were due to sampling variability. To see if this is the case, we will test the hypothesis that μ = 1000 against the alternative that μ1000 at a significance level of a = 0.05. If we look up the critical value for this problem in the t-table, we would find that it is 2.365. • The magnitude of the t-score is( smaller greater) than the critical value. Therefore we should (O fail to reject reject) the hypothesis that μ = 1000 • Therefore the evidence (does does not) provide significant reason to believe that the plant is discharging more wastewater on average than they claim to be.

Create an Excel Spreadsheet for a beer rating study Evaluate the impact of caffeine on crankiness Write the APA formatted results for this analysis, including R2  for the results and post hoc analyses if needed Participant ID.        Caffeine Level                Crankiness Score 1                                   0 MG                                  40 2                                  0 MG                                   42 3                                  0 MG                                  30 1                                  50 MG                                 30 2                                 50 MG.                                 45 3                                 50 MG                                   38 1                                 100 MG                                  53 2                                 100 MG                                   65 3                                 100 MG                                     64

Statistical Laboratory  Link of dataset: https://www.kaggle.com/datasets/johnharshith/world-happiness-report-2021-worldwide-mortality Country name Population 2020 Population 2019 United States 331002647 328239523 Egypt 102334403 100388073 Morocco 36910558 36471769 Lebanon 6825442 6855713 Saudi Arabia 34813867 34268528 Jordan 10203140 10101694 Syria 17500657 17070135 Turkey 84339067 83429615 Pakistan 220892331 216565318 Indonesia 273523621 270625568 Bangladesh 164689383 163046161 United Kingdom 67886004 66834405 France 65273512 67059887 Germany 83783945 83132799 Netherlands 17134873 17332850 Belgium 11589616 11484055 Spain 46754783 47076781 Italy 60461828 60297396 Poland 37846605 37970874 Hungary 9660350 9769949 Czech Republic 10708982 10669709 Romania 19237682 19356544 Sweden 10099270 10285453 Greece 10423056 10716322 Denmark 5792203 5818553 Iran 83992953 82913906 Hong Kong S.A.R. of China 7496988 7451000 Singapore 5850343…

A boat capsized and sank in a lake. Based on an assumption of a mean weight of 142 lb, the boat was rated to carry 60 passengers (so the load limit was 8,520 lb). After the boat sank, the assumed mean weight for similar boats was changed from 142 lb to 171 lb. Complete parts a and b below. a. Assume that a similar boat is loaded with 60 passengers, and assume that the weights of people are normally distributed with a mean of 178.5 lb and a standard deviation of 36.9 lb. Find the probability that the boat is overloaded because the 60 passengers have a mean weight greater than 142 lb. The probability is 1.000. (Round to four decimal places as needed.) C.. b. The boat was later rated to carry only 13 passengers, and the load limit was changed to 2,223 lb. Find the probability that the boat is overloaded because the mean weight of the passengers is greater than 171 (so that their total weight is greater than the maximum capacity of 2,223 lb). The probability is. (Round to four decimal…

What percentage of Contra Costa County adults supports a ban on assault-style weapons? Suppose that we survey a random sample of 100 adults living in Contra Costa County and find that 62% support a ban on assault-style weapons. According to  a  2015 study by  the Pew  Research Center, 57% of U.S.  adults  favor a ban on assault-style weapons. Assuming that the variability in random samples will be the same in Contra Costa County as in the U.S., find the 95% confidence interval to estimate the proportion of CCC adults that favor a ban on assault-style weapons.   g. Are we confident that the percentage of Contra Costa County residents that supports a ban is greater than the percentage nationwide as reported by the Pew Research Center? Why or why not?

A. Determine the test statistic  B. What are the critical values C. What is the p value

A researcher was interested in comparing the resting pulse rate of people who exercise regularly and people who do not exercise regularly. Independent random samples of 16 people aged 30-40 who do not exercise regularly (sample 1) and 12 people aged 30-40 who do exercise regularly (sample 2) were selected and the resting pulse rate of each person was measured. The summary statistics are as follows: Pulse Rate data   Group 1 (no exercise) Group 2 (exercise) average 72.7 69.7 standard deviation 10.9 8.2 sample size 16 12 Test the claim that the mean resting pulse rate of people who do not exercise regularly is greater than the mean resting pulse rate of people who exercise regularly, use 0.01 as the significance level. Round you answer to 3 decimal places. Group of answer choices p-value=0.207, evidence not support claim p-value=0.267, evidence support claim p-value=0.414, evidence not support claim p-value=0.793, evidence not support claim p-value=0.207, evidence…