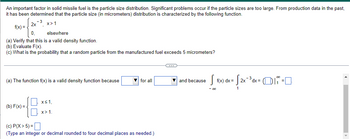
An important factor in solid missile fuel is the particle size distribution. Significant problems occur if the particle sizes are too large. From production data in the past, it has been determined that the particle size (in micrometers) distribution is characterized by the following function. 2x x>1 0₁ elsewhere (a) Verify that this is a valid density function. (b) Evaluate F(x). (c) What is the probability that a random particle from the manufactured fuel exceeds 5 micrometers? f(x) = (a) The function f(x) is a valid density function because 18 (b) F(x) = { x≤ 1, x>1. for all (c) P(X> 5) = (Type an integer or decimal rounded to four decimal places as needed.) | and because_ [ f(x) dx = √2x²³dx = Of=[ -00 1

Suppose X is exponentially distributed with mean 2. Let Y = eX. What is the density function of Y?

2. In probability, it is common to model the deviation of a day's temperature from the monthly average temperature using the Gaussian probability density function, 1.-/9 f(t) = V9T This means that the probability that the day's temperature will be between t = a and t = b different from the monthly average temperature is given by the area under the graph of y = f(t) between t a andt = b. A related function is %3D F(x) = -2/9 dt, 2 0. %3D V9n Jo This function gives the probability that the day's temperature is between t = -x and t = x different from the monthly average temperature. For example, F(1) 0.36 indicates that there's roughly a 36% chance that the day's temperature will be within 1 degree (between 1 degree less and 1 degree more) of the monthly average. 1 (a) Find a power series representation of F(x) (write down the power series using sigma notation). (b) Use your answer to (a) to find a series equal to the probability that the day's temperature will be within 2 degrees of the…

#1 please