Advanced Engineering Mathematics
10th Edition
ISBN: 9780470458365
Author: Erwin Kreyszig
Publisher: Wiley, John & Sons, Incorporated
expand_more
expand_more
format_list_bulleted
Related questions
Question
33
![23. a. Every elementary row operation is reversible.
2b. A5 x 6 matrix has six rows.
c. The solution set of a linear system involving variables
X₁,..., Xn is a list of numbers (S₁,..., Sn) that makes each
equation in the system a true statement when the values
S₁,..., S, are substituted for x₁, ..., xn, respectively.
d. Two fundamental questions about a linear system involve
existence and uniqueness.
24. a. Elementary row operations on an augmented matrix never
change the solution set of the associated linear system.
sup b. Two matrices are row equivalent if they have the same
BOB number of rows. Duping at 11 bis 28es dist
c. An inconsistent system has more than one solution.
bd. Two linear systems are equivalent if they have the same
solution set.
25. Find an equation involving g, h, and k that makes this
augmented matrix correspond to a consistent system:
Sirjeigule)
1
0
-2
-4
3
5
1201
27. Suppose the system below is consistent for all possible values
of f and g. What can you say about the coefficients c and d?
Justify your answer.
x₁ + 3x₂ = f
cx₁ + dx₂ = g
g
h | + 15- = (S-)-(B)2 +
ax₁ + bx₂ = f
cx₁ + dx₂ = g
1=px-p
NE+AE O
28. Suppose a, b, c, and d are constants such that a is not zero
and the system below is consistent for all possible values of
f and g. What can you say about the numbers a, b, c, and d?
Justify your answer.
29.
30.
[!
·[
1
31.
endogiA
32.
0-2
4 -7
1
3 -1
0-2
1 3 -4
0-5
104
5
-][
96
100
6
9
1.1 Systems of Linear Equations 11
-2
5
4 -1 3 -6
1
-2
1.1
0
0 -3 9
201 2500
1 4 -7
8
5
3 -1 6
2-5
1 -3 -2
0
UNO
* ~-4
5
On 1276
7
-50
-9 k
on et buds ads, L 1182 916, nousu
T₁ = (10+20+ T₂+ T4)/4,
26. Construct three different augmented matrices for linear sys-
o mare de igno
tems whose solution set is x₁ = -2, x₂ = 1, x3 = 0.
nomnd
amooon vignonte ne vou
nuits JameS
or
itsupa brosse od) nad W
28 Dobr
10°
geomotod
stark
-2
1
0
][!
0
3 -4
0-5
10°
1 -3
ܬ ܚ ܩ ܐ ܝ ܗ ܒ
1
0
5-2 8
1
2
0
1
0 0 0
1
7
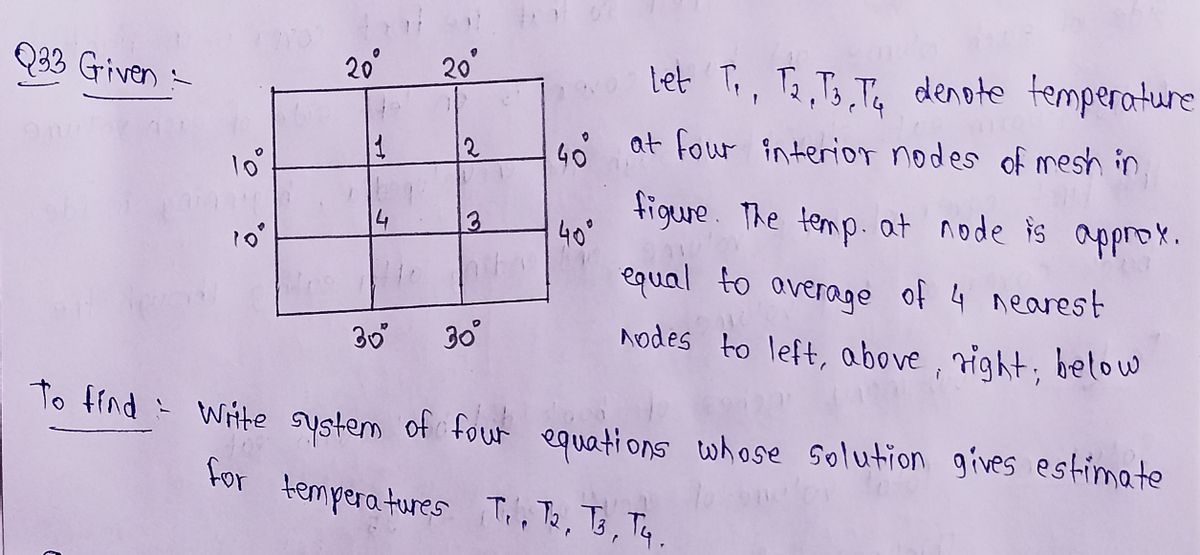
An important concern in the study of heat transfer is to determine
the steady-state temperature distribution of a thin plate when the
temperature around the boundary is known. Assume the plate
shown in the figure represents a cross section of a metal beam,
with negligible heat flow in the direction perpendicular to the
plate. Let T₁,..., T4 denote the temperatures at the four interior
nodes of the mesh in the figure. The temperature at a node is
approximately equal to the average of the four nearest nodes-
to the left, above, to the right, and below. For instance,
ort
4
20° 20°
-1 -6]
-5 0
-3
-3-2
or 4T₁-T₂-T4 = 30
It anottarps silt
2
3
0 -1
30° 30°
40°
leading entrica
40° (includi
33. Write a system of four equations whose solution gives esti-
mates for the temperatures T₁,..., T4.
34.
Solve the system of equations from Exercise 33. [Hint: To
speed up the calculations, interchange rows 1 and 4 before
starting "replace" operations.DUGA WOR
2MMO
01
u
In Exercises 29-32, find the elementary row operation that trans-
forms the first matrix into the second, and then find the reverse 1m2 See Frank M. White, Heat and Mass Transfer (Reading, MA:
row operation that transforms the second matrix into the first.
Addison-Wesley Publishing, 1991), pp. 145-149.
sw
samupinu bas sonbizizs (ainomsbaut brü rowenn i oleh od liv dingin di
0 0101 hoitop2 ni bez
wory i ximam odt 100 10 10diodw
em vms of asilqqs minogle od
noilooz aid to hug til ors
smil sot xrd botnsingus
alo inmoqmi o SOLUTIONS TO PRACTICE PROBLEMS VIST!
ed aroitinitob
1 200
on & 0) (05 OTOSTON
1. a. For "hand computation," the best choice is to interchange equations 3 and 4.
Another possibility is to multiply equation 3 by 1/5. Or, replace equation 4 by
its sum with -1/5 times row 3. (In any case, do not use the x2 in equation 2 to
eliminate the 4x2 in equation 1. Wait until a triangular form has been reached and
the x3 terms and x4 terms have been eliminated from the first two equations.)
b. The system is in triangular form. Further simplification begins with the x4 in the
fourth equation. Use the x4 to eliminate all x4 terms above it. The appropriate](https://content.bartleby.com/qna-images/question/2dd9a37c-a92c-4ea7-92a9-c508cbd79da9/43863383-bb51-4bc2-9004-029ae99103c2/1gkqlc_processed.jpeg)
Transcribed Image Text:23. a. Every elementary row operation is reversible.
2b. A5 x 6 matrix has six rows.
c. The solution set of a linear system involving variables
X₁,..., Xn is a list of numbers (S₁,..., Sn) that makes each
equation in the system a true statement when the values
S₁,..., S, are substituted for x₁, ..., xn, respectively.
d. Two fundamental questions about a linear system involve
existence and uniqueness.
24. a. Elementary row operations on an augmented matrix never
change the solution set of the associated linear system.
sup b. Two matrices are row equivalent if they have the same
BOB number of rows. Duping at 11 bis 28es dist
c. An inconsistent system has more than one solution.
bd. Two linear systems are equivalent if they have the same
solution set.
25. Find an equation involving g, h, and k that makes this
augmented matrix correspond to a consistent system:
Sirjeigule)
1
0
-2
-4
3
5
1201
27. Suppose the system below is consistent for all possible values
of f and g. What can you say about the coefficients c and d?
Justify your answer.
x₁ + 3x₂ = f
cx₁ + dx₂ = g
g
h | + 15- = (S-)-(B)2 +
ax₁ + bx₂ = f
cx₁ + dx₂ = g
1=px-p
NE+AE O
28. Suppose a, b, c, and d are constants such that a is not zero
and the system below is consistent for all possible values of
f and g. What can you say about the numbers a, b, c, and d?
Justify your answer.
29.
30.
[!
·[
1
31.
endogiA
32.
0-2
4 -7
1
3 -1
0-2
1 3 -4
0-5
104
5
-][
96
100
6
9
1.1 Systems of Linear Equations 11
-2
5
4 -1 3 -6
1
-2
1.1
0
0 -3 9
201 2500
1 4 -7
8
5
3 -1 6
2-5
1 -3 -2
0
UNO
* ~-4
5
On 1276
7
-50
-9 k
on et buds ads, L 1182 916, nousu
T₁ = (10+20+ T₂+ T4)/4,
26. Construct three different augmented matrices for linear sys-
o mare de igno
tems whose solution set is x₁ = -2, x₂ = 1, x3 = 0.
nomnd
amooon vignonte ne vou
nuits JameS
or
itsupa brosse od) nad W
28 Dobr
10°
geomotod
stark
-2
1
0
][!
0
3 -4
0-5
10°
1 -3
ܬ ܚ ܩ ܐ ܝ ܗ ܒ
1
0
5-2 8
1
2
0
1
0 0 0
1
7
An important concern in the study of heat transfer is to determine
the steady-state temperature distribution of a thin plate when the
temperature around the boundary is known. Assume the plate
shown in the figure represents a cross section of a metal beam,
with negligible heat flow in the direction perpendicular to the
plate. Let T₁,..., T4 denote the temperatures at the four interior
nodes of the mesh in the figure. The temperature at a node is
approximately equal to the average of the four nearest nodes-
to the left, above, to the right, and below. For instance,
ort
4
20° 20°
-1 -6]
-5 0
-3
-3-2
or 4T₁-T₂-T4 = 30
It anottarps silt
2
3
0 -1
30° 30°
40°
leading entrica
40° (includi
33. Write a system of four equations whose solution gives esti-
mates for the temperatures T₁,..., T4.
34.
Solve the system of equations from Exercise 33. [Hint: To
speed up the calculations, interchange rows 1 and 4 before
starting "replace" operations.DUGA WOR
2MMO
01
u
In Exercises 29-32, find the elementary row operation that trans-
forms the first matrix into the second, and then find the reverse 1m2 See Frank M. White, Heat and Mass Transfer (Reading, MA:
row operation that transforms the second matrix into the first.
Addison-Wesley Publishing, 1991), pp. 145-149.
sw
samupinu bas sonbizizs (ainomsbaut brü rowenn i oleh od liv dingin di
0 0101 hoitop2 ni bez
wory i ximam odt 100 10 10diodw
em vms of asilqqs minogle od
noilooz aid to hug til ors
smil sot xrd botnsingus
alo inmoqmi o SOLUTIONS TO PRACTICE PROBLEMS VIST!
ed aroitinitob
1 200
on & 0) (05 OTOSTON
1. a. For "hand computation," the best choice is to interchange equations 3 and 4.
Another possibility is to multiply equation 3 by 1/5. Or, replace equation 4 by
its sum with -1/5 times row 3. (In any case, do not use the x2 in equation 2 to
eliminate the 4x2 in equation 1. Wait until a triangular form has been reached and
the x3 terms and x4 terms have been eliminated from the first two equations.)
b. The system is in triangular form. Further simplification begins with the x4 in the
fourth equation. Use the x4 to eliminate all x4 terms above it. The appropriate
Expert Solution
arrow_forward
Step 1
Q33 asked and answered.
Trending nowThis is a popular solution!
Step by stepSolved in 2 steps with 2 images
Knowledge Booster
Similar questions
Recommended textbooks for you
- Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated
Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education
Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY 
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Advanced Engineering Mathematics
Advanced Math
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Wiley, John & Sons, Incorporated
Numerical Methods for Engineers
Advanced Math
ISBN:9780073397924
Author:Steven C. Chapra Dr., Raymond P. Canale
Publisher:McGraw-Hill Education
Introductory Mathematics for Engineering Applicat...
Advanced Math
ISBN:9781118141809
Author:Nathan Klingbeil
Publisher:WILEY
Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,