Question
4
Transcribed Image Text:Advanced Physics
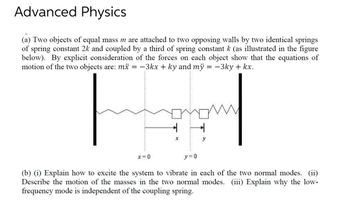
(a) Two objects of equal mass m are attached to two opposing walls by two identical springs
of spring constant 2k and coupled by a third of spring constant k (as illustrated in the figure
below). By explicit consideration of the forces on each object show that the equations of
motion of the two objects are: mx = -3kx + ky and mỹ = -3ky + kx.
Juf
x=0
X
y=0
y
ww
(b) (i) Explain how to excite the system to vibrate in each of the two normal modes. (ii)
Describe the motion of the masses in the two normal modes. (iii) Explain why the low-
frequency mode is independent of the coupling spring.
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by stepSolved in 2 steps with 1 images

Knowledge Booster
Similar questions
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios